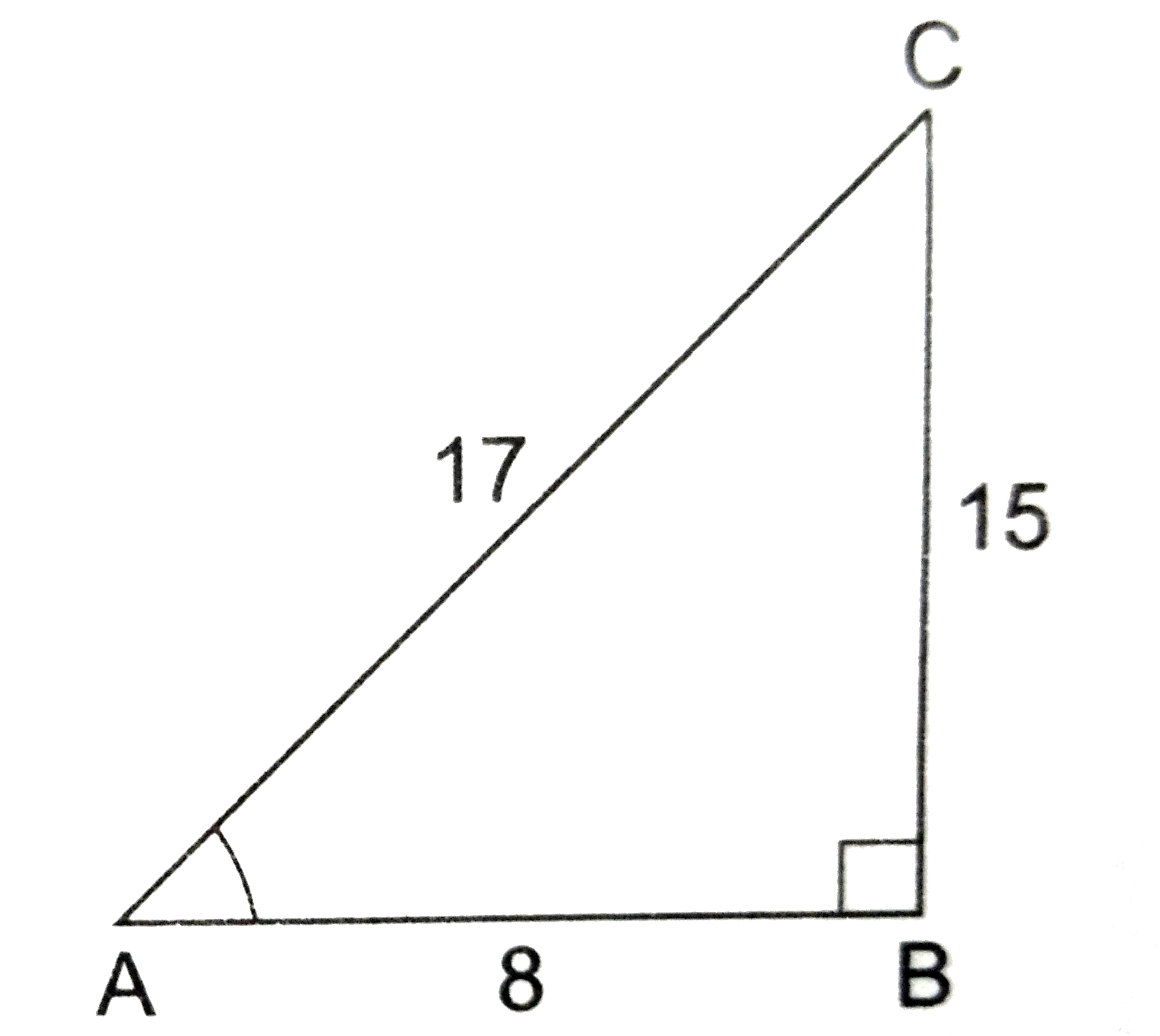
Given : $15 cot A = 8$ find $sin A and sec A$

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $sin A = \frac{15}{17}, sec A = \frac{17}{8}$

$cot A = \frac{8}{15} \Rightarrow tan A = \frac{15}{8} = \frac{B C}{A B} .$
$∴ A C^{2} = A B^{2} + B C^{2} = {(8)}^{2} + {(15)}^{2} = 64 + 225 = 289$
$\Rightarrow A C = \sqrt{289} = 17 .$
$∴ sin A = \frac{B C}{A C} = \frac{15}{17} and sec A = \frac{A C}{A B} = (17) = (8) .$

|

Updated on:21/07/2023

Class 10 MATHS TRIGONOMETRIC RATIOS AND IDENTITIES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If 15 $cot A = 8$ , the value of $sin A$ is :
A $\frac{8}{17}$
B $\frac{15}{17}$
C $\frac{17}{8}$
D $\frac{17}{15}$
Question 2 - Select One
If $0 < A < π / 2$ and sinA + cosA + tan A + cotA + secA + cosec A=7 and sinA and cosA are roots of equation $4 x^{2} - 3 x + a = 0$ . Then value of 25a is:
A28
B30
C26
D32

RS AGGARWAL-TRIGONOMETRIC RATIOS-Exercise 10

Home
Ask Doubt
Profile