Given P={x:5<2x−1≤11,x∈R},Q={x:−1≤3+4x<23,x∈I} where R = {real numbers}, I = {Integers}.
Represent P and Q on number lines. Write down the elements of P∩Q.
The correct Answer is:P∩Q={4}
Topper's Solved these Questions
- BOOK - ICSECHAPTER - INEQUALITIESEXERCISE - EXERCISE 11 (a)32videos
INEQUALITIES
- BOOK - ICSECHAPTER - INEQUALITIESEXERCISE - EXERCISE 11 (b)1videos
INEQUALITIES
- BOOK - ICSECHAPTER - INDEX NUMBERS AND MOVING AVERAGES EXERCISE - MULTIPLE CHOICE QUESTIONS10videos
INDEX NUMBERS AND MOVING AVERAGES
- BOOK - ICSECHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - MULTIPLE CHOICE QUESTIONS17videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
Similar Questions
Given P={x:9<2x−1≤13,x∈R}andQ={x:−5≤3+4x<15,x∈I}Where R={Real Numbers} and I={Integers}.Represent P and Q on number lines. Write down the elements of P∩Q
View SolutionGiven : P = {x:5<2x−1≤11,x∈R}
Q={x:1≤3+4x<23,x∈I}
where R = (real numbers) and I = (integers).
Represent P and Q on two different number lines. Write down the elements of P∩Q.View Solution
Knowledge Check
- Question 1 - Select One
If −1<3+4x≤23,x∈R (real numbers), then the solution set of x is
A−1≤x<5,x∈RB−1<x≤5,x∈RC−1≤x≤5,x∈RD−1<x<5,x∈R - Question 2 - Select One
The inequality −1≤2x+4<5, where x is an integer can be represented on the number line as:
A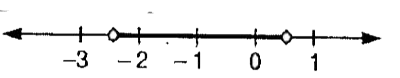 B
B C
C D
D
Given : A={x:3<2x−1<9,x∈R},B={x:11≤3x+2≤23,x∈R}
where R is the set of real numbers.
Represent A and B on number linesView SolutionGiven P={x:5<2x−1≤11,x∈R} and Q={x:−1≤3+4x<23,x∈I}
where R = {real number } and I = { integers}.View SolutionGive : A={x:−8<5x+2≤17,x∈I}
B={x:−2≤7+3x<17,x∈R}
Where R = {real numbers} and I ={integers}. Represent A and B on two different number lines. Write down the elements of A∩B.View SolutionGiven : A={x:3<2x−1<9,x∈R},B={x:11≤3x+2≤23,x∈R}
where R is the set of real numbers.
On the number line also mark A∩BView Solutionx∈ {real numbers} and −1<3−2x≤7, evaluate x and represent it on a number line.
View SolutionGiven A={x:−1<x≤5,x∈R}andB={x:−4≤x<3,x∈R}.
Represent on different number line :
A - BView Solution

