Given : A={x:3<2x−1<9,x∈R},B={x:11≤3x+2≤23,x∈R}
where R is the set of real numbers.
Represent A and B on number lines
To solve the problem, we need to find the sets A and B based on the given inequalities and then represent them on a number line.
Step 1: Solve for Set A
The set A is defined by the inequality:
3<2x−1<9
Step 1.1: Break down the compound inequality into two parts:
1. 3<2x−1
2. 2x−1<9
Step 1.2: Solve the first part:
3<2x−1
Add 1 to both sides:
3+1<2x
4<2x
Now, divide both sides by 2:
2<x
This means x>2.
Step 1.3: Solve the second part:
2x−1<9
Add 1 to both sides:
2x<9+1
2x<10
Now, divide both sides by 2:
x<5
Step 1.4: Combine the results:
From the two parts, we have:
2<x<5
So, set A can be written as:
A={x:2<x<5,x∈R}
Step 2: Solve for Set B
The set B is defined by the inequality:
11≤3x+2≤23
Step 2.1: Break down the compound inequality into two parts:
1. 11≤3x+2
2. 3x+2≤23
Step 2.2: Solve the first part:
11≤3x+2
Subtract 2 from both sides:
11−2≤3x
9≤3x
Now, divide both sides by 3:
3≤x
This means x≥3.
Step 2.3: Solve the second part:
3x+2≤23
Subtract 2 from both sides:
3x≤23−2
3x≤21
Now, divide both sides by 3:
x≤7
Step 2.4: Combine the results:
From the two parts, we have:
3≤x≤7
So, set B can be written as:
B={x:3≤x≤7,x∈R}
Step 3: Represent A and B on Number Lines
For Set A:
- The interval (2,5) is open at both ends, meaning 2 and 5 are not included.
- On a number line, we would represent this as an open interval between 2 and 5.
For Set B:
- The interval (3,7) is closed at both ends, meaning 3 and 7 are included.
- On a number line, we would represent this as a closed interval between 3 and 7.
Final Representation:
- Set A: Open interval (2, 5)
- Set B: Closed interval (3, 7)
The set A is defined by the inequality:
3<2x−1<9
1. 3<2x−1
2. 2x−1<9
3<2x−1
Add 1 to both sides:
3+1<2x
4<2x
Now, divide both sides by 2:
2<x
This means x>2.
2x−1<9
Add 1 to both sides:
2x<9+1
2x<10
Now, divide both sides by 2:
x<5
From the two parts, we have:
2<x<5
So, set A can be written as:
A={x:2<x<5,x∈R}
The set B is defined by the inequality:
11≤3x+2≤23
1. 11≤3x+2
2. 3x+2≤23
11≤3x+2
Subtract 2 from both sides:
11−2≤3x
9≤3x
Now, divide both sides by 3:
3≤x
This means x≥3.
3x+2≤23
Subtract 2 from both sides:
3x≤23−2
3x≤21
Now, divide both sides by 3:
x≤7
From the two parts, we have:
3≤x≤7
So, set B can be written as:
B={x:3≤x≤7,x∈R}
For Set A:
- The interval (2,5) is open at both ends, meaning 2 and 5 are not included.
- On a number line, we would represent this as an open interval between 2 and 5.
- The interval (3,7) is closed at both ends, meaning 3 and 7 are included.
- On a number line, we would represent this as a closed interval between 3 and 7.
- Set A: Open interval (2, 5)
- Set B: Closed interval (3, 7)
Topper's Solved these Questions
Similar Questions
Given : A={x:3<2x−1<9,x∈R},B={x:11≤3x+2≤23,x∈R}
where R is the set of real numbers.
On the number line also mark A∩B
View SolutionGiven : P = {x:5<2x−1≤11,x∈R}
Q={x:1≤3+4x<23,x∈I}
where R = (real numbers) and I = (integers).
Represent P and Q on two different number lines. Write down the elements of P∩Q.
View Solution
Knowledge Check
If −5<2x−3≤x+2,x∈R, then the solution set of x on the number line is
A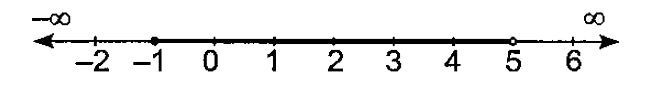 B
B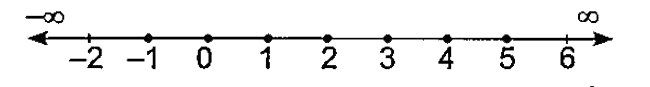 C
C D
D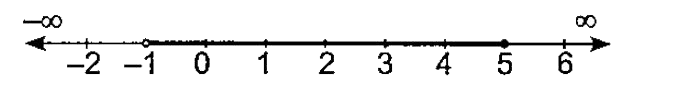
Give : A={x:−8<5x+2≤17,x∈I}
B={x:−2≤7+3x<17,x∈R}
Where R = {real numbers} and I ={integers}. Represent A and B on two different number lines. Write down the elements of A∩B.
View SolutionGiven P={x:5<2x−1≤11,x∈R} and Q={x:−1≤3+4x<23,x∈I}
where R = {real number } and I = { integers}.
View SolutionGiven A={x:−1<x≤5,x∈R}andB={x:−4≤x<3,x∈R}.
Represent on different number line :
A'∩B
View SolutionGiven P={x:9<2x−1≤13,x∈R}andQ={x:−5≤3+4x<15,x∈I}Where R={Real Numbers} and I={Integers}.Represent P and Q on number lines. Write down the elements of P∩Q
View SolutionGiven A={x:−1<x≤5,x∈R}andB={x:−4≤x<3,x∈R}.
Represent on different number line :
A - B
View Solutionx∈ {real numbers} and −1<3−2x≤7, evaluate x and represent it on a number line.
View SolutionIllustrate the set {x:−3≤x<0orx>2,x∈R} on a real number line.
View Solution
