Given the following information about the production and demand of a commodity.
Obtain the two regression lines :
PRODUCTION(X)DEMAND(Y)MEAN8590VARIANCE2536
Coefficient of correlation between X and Y is 0.6. Also estimate the demand when the production is 100 units.
The correct Answer is:100.8 units.
To solve the problem, we need to find the two regression lines based on the given data and then estimate the demand when the production is 100 units.
1. Identify the Given Data:
- Mean of Production (X̄) = 85
- Mean of Demand (Ȳ) = 90
- Variance of Production (σ²_X) = 25 → Standard Deviation (σ_X) = √25 = 5
- Variance of Demand (σ²_Y) = 36 → Standard Deviation (σ_Y) = √36 = 6
- Coefficient of Correlation (r) = 0.6
Hint: Write down all the given values clearly to avoid confusion later.
2. Calculate the Regression Coefficient of Y on X (B_YX):
BYX=r⋅σYσX=0.6⋅65=3650=0.72
Hint: Use the formula for regression coefficients carefully, ensuring you substitute the correct values.
3. Calculate the Regression Coefficient of X on Y (B_XY):
BXY=r⋅σXσY=0.6⋅56=3036=56
Hint: Remember that the regression coefficients are derived from the correlation coefficient and the standard deviations.
4. Formulate the Regression Equation of Y on X:
Y−Yˉ=BYX⋅(X−Xˉ)
Substituting the known values:
Y−90=0.72⋅(X−85)
Rearranging gives:
Y=0.72X−0.72⋅85+90
Y=0.72X−61.2+90
Y=0.72X+28.8
Hint: Ensure to simplify the equation correctly after substituting the values.
5. Estimate Demand (Y) when Production (X) is 100:
Substitute X = 100 into the regression equation:
Y=0.72(100)+28.8=72+28.8=100.8
Hint: Always double-check your arithmetic when substituting values into equations.
6. Formulate the Regression Equation of X on Y:
X−Xˉ=BXY⋅(Y−Yˉ)
Substituting the known values:
X−85=56⋅(Y−90)
Rearranging gives:
X=56(Y−90)+85
X=56Y−75+85
X=56Y+10
Hint: Follow the same steps as before to ensure the equation is correctly derived.
Final Results
- The regression equation of Y on X is:
Y=0.72X+28.8
- The regression equation of X on Y is:
X=56Y+10
- Estimated Demand when Production is 100 units:
Y=100.8
- Mean of Production (X̄) = 85
- Mean of Demand (Ȳ) = 90
- Variance of Production (σ²_X) = 25 → Standard Deviation (σ_X) = √25 = 5
- Variance of Demand (σ²_Y) = 36 → Standard Deviation (σ_Y) = √36 = 6
- Coefficient of Correlation (r) = 0.6
BYX=r⋅σYσX=0.6⋅65=3650=0.72
BXY=r⋅σXσY=0.6⋅56=3036=56
Y−Yˉ=BYX⋅(X−Xˉ)
Substituting the known values:
Y−90=0.72⋅(X−85)
Rearranging gives:
Y=0.72X−0.72⋅85+90
Y=0.72X−61.2+90
Y=0.72X+28.8
Substitute X = 100 into the regression equation:
Y=0.72(100)+28.8=72+28.8=100.8
X−Xˉ=BXY⋅(Y−Yˉ)
Substituting the known values:
X−85=56⋅(Y−90)
Rearranging gives:
X=56(Y−90)+85
X=56Y−75+85
X=56Y+10
- The regression equation of Y on X is:
Y=0.72X+28.8
- The regression equation of X on Y is:
X=56Y+10
- Estimated Demand when Production is 100 units:
Y=100.8
Topper's Solved these Questions
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 3. LINEAR REGRESSION (V. Activity questions:)
8 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 4. TIME SERIES (I) Choose the correct alternative.)
5 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 3. LINEAR REGRESSION (III. Fill in the following blanks:)
11 Videos
- PROBABILITY DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
9 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CHOICE QUESTIONS
8 Videos
Similar Questions
Given the following information about the production and demand of acommodity.
Obtain the two regression lines :
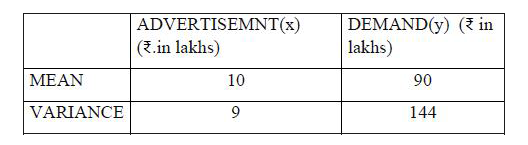
Coefficient of correlation between x and y is 0.8.
What should be the advertising budget if the company wants to attain the sales target of Rs.150 lakhs?
View SolutionThe co-efficient of correlation between two variables x and y is 0.5. Their co-variance is 15 and σx=6, Then σy=
View Solution
Knowledge Check
If the coefficient of correlation between x and y os 0.6, covariance is 27 and variance of y is 25, then what is the variance x?
A9/5B81/25C9D81The coefficient of correlation between X and Y is 0.6 U and V are two variables defined as U=x−32,V=y−23, then the coefficient of correlation between U and V is
A0.1B0.3C0.2D1If the correlation coefficient between x and y is 0.6, covariance is 27 and variance of y is 25, then what is the variance of x?
A95B8125C9D81
Coefficient of correlation between two variable X and Y is 0.32. Their covariance is 7.86. The variance of X is 10. Find the standard deviation of Y-series.
View SolutionFind (i) mean →xand→y, (ii) regression coefficeint byxandbxy, (iii) coefficeint of correlation between x and y for the following two regression lines 2x+3y-10=0 and 4x+y-5=0
View SolutionIf the two lines of regression are 3x+2y=26and6x+y=31, then the coefficient of correlation between x and y is ……………..
View SolutionThe coefficient of correlation between random variables X and Y is 0.28, covariance between X and Y is 7.6 and the variance of X is 9, then the standard deviation of Y series is
View SolutionWhen x falls, y also falls. There is perfect correlation between the two. The correlation coefficient between the two is
View Solution
