Coefficient of correlation between two variable X and Y is 0.32. Their covariance is 7.86. The variance of X is 10. Find the standard deviation of Y-series.
To find the standard deviation of the Y-series given the coefficient of correlation, covariance, and variance of X, we can follow these steps:
Step 1: Understand the relationship between the variables
The coefficient of correlation (r) is given by the formula:
r=Cov(X,Y)σXσY
where:
- Cov(X,Y) is the covariance between X and Y,
- σX is the standard deviation of X,
- σY is the standard deviation of Y.
Step 2: Rearrange the formula to find σY
From the formula, we can rearrange it to solve for σY:
σY=Cov(X,Y)r⋅σX
Step 3: Substitute the known values
We know:
- Cov(X,Y)=7.86
- r=0.32
- The variance of X is given as 10. Therefore, the standard deviation of X (σX) is:
σX=√Variance of X=√10
Step 4: Calculate σX
Calculating σX:
σX=√10≈3.162
Step 5: Substitute into the equation for σY
Now we can substitute the values into the equation for σY:
σY=7.860.32⋅3.162
Step 6: Calculate the denominator
Calculating the denominator:
0.32⋅3.162≈1.01184
Step 7: Calculate σY
Now substituting back:
σY=7.861.01184≈7.76
Conclusion
Thus, the standard deviation of the Y-series is approximately 7.76.
The coefficient of correlation (r) is given by the formula:
r=Cov(X,Y)σXσY
where:
- Cov(X,Y) is the covariance between X and Y,
- σX is the standard deviation of X,
- σY is the standard deviation of Y.
From the formula, we can rearrange it to solve for σY:
σY=Cov(X,Y)r⋅σX
We know:
- Cov(X,Y)=7.86
- r=0.32
- The variance of X is given as 10. Therefore, the standard deviation of X (σX) is:
σX=√Variance of X=√10
Calculating σX:
σX=√10≈3.162
Now we can substitute the values into the equation for σY:
σY=7.860.32⋅3.162
Calculating the denominator:
0.32⋅3.162≈1.01184
Now substituting back:
σY=7.861.01184≈7.76
Thus, the standard deviation of the Y-series is approximately 7.76.
Topper's Solved these Questions
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Solved Problems(Objective)
24 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Exercise 1
5 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Comprehension Type
6 Videos
- SET, RELATION & FUNCTIONBOOK - FIITJEECHAPTER - SET, RELATION & FUNCTIONEXERCISE - Exercise 3
8 Videos
- STRAIGHT LINE BOOK - FIITJEECHAPTER - STRAIGHT LINE EXERCISE - NUMERICAL BASED
4 Videos
Similar Questions
Knowledge Check
The coefficient of correlation between random variables X and Y is 0.28, covariance between X and Y is 7.6 and the variance of X is 9, then the standard deviation of Y series is
A9.8B10.1C9.05D10.05If the coefficient of correlation between x and y os 0.6, covariance is 27 and variance of y is 25, then what is the variance x?
A9/5B81/25C9D81Correlation coefficient r of two variables X and Y is positive when
AσX+Y>σX−YBσX−Y>σX+YCσX+Y=0Dnone of these
The co-efficient of correlation between two variables x and y is 0.5. Their co-variance is 15 and σx=6, Then σy=
View SolutionFind out coefficient of rank correlation between X and Y
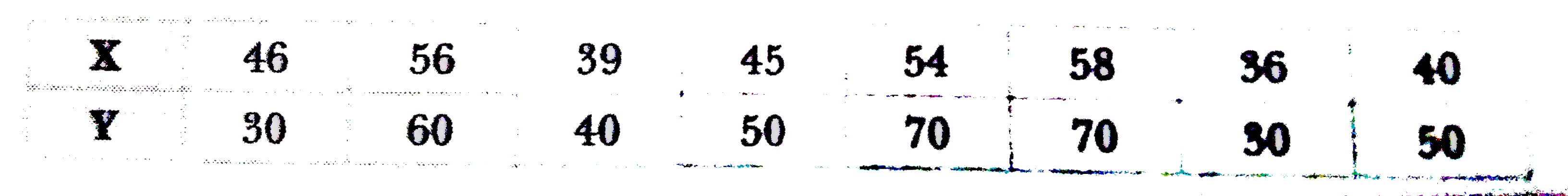
View SolutionThe standard deviationof a variable x is 10.Then the standard deviation of 50+5x is :
View SolutionThe standard deviationof a variable x is 10.Then the standard deviation of 50+5x is :
View SolutionThe standard deviation of a variable x is 10. Then , the standard deviation of 50+5x is
View Solution

