If $A (¯ a) and B (¯ b)$ are any two points in the space
and $R (¯ r)$ be a point on the line segment AB
dividing it internally in the ratio m : n , then prove that :
$¯ r = \frac{m ¯ b + n ¯ a}{m + n}$

Video Solution

Text Solution

Verified by Experts

As R is point on line segment AB (A - R - B ) and
$\frac{A R}{R B} = \frac{m}{n}$

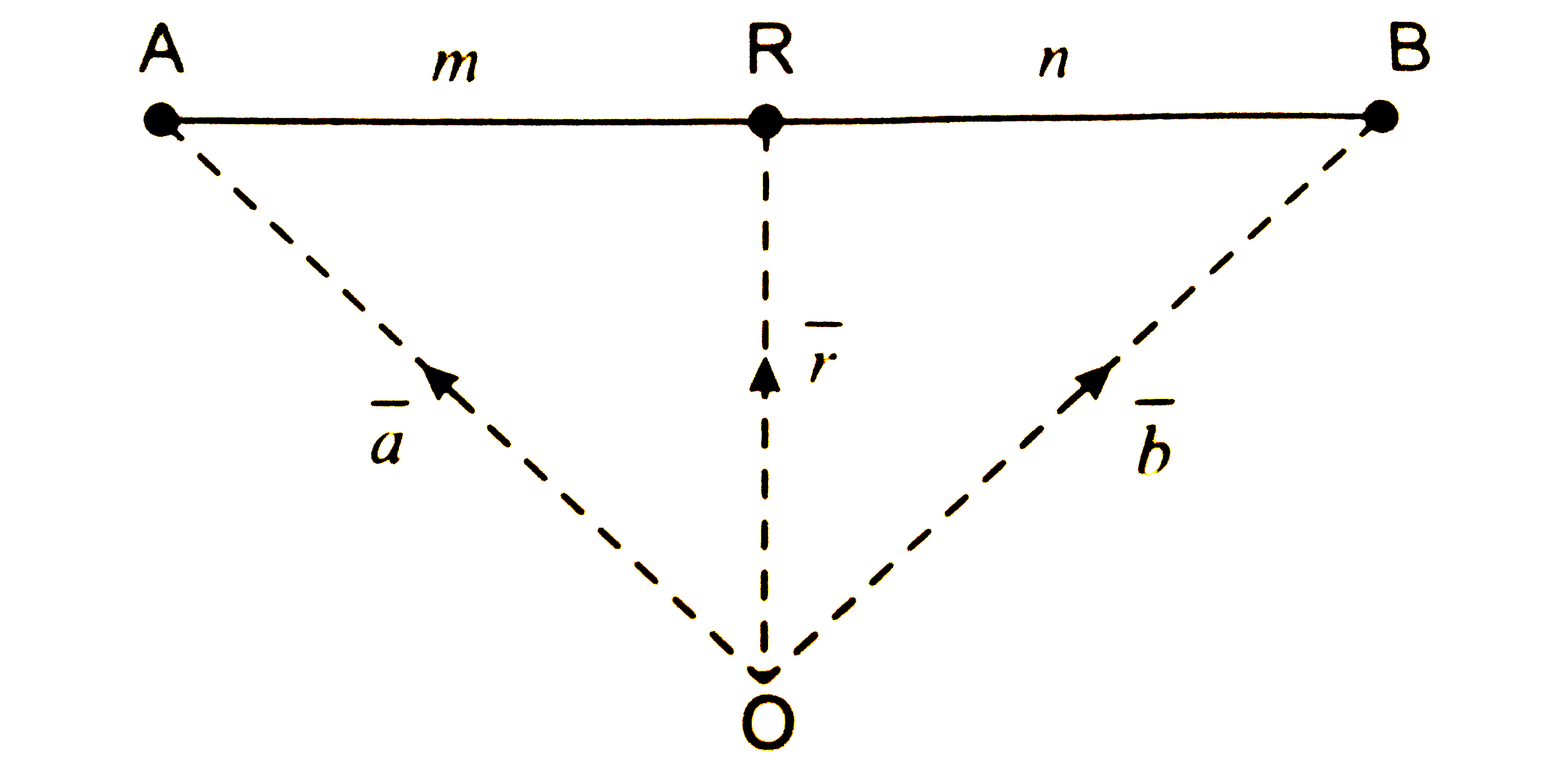
$∴ m (R B) = n (A R) and ¯ ¯¯¯¯ ¯ A R and ¯ ¯¯¯¯ ¯ A B$ are in same direction .
$∴ m ((¯ ¯¯¯¯ ¯ R B) = n (¯ ¯¯¯¯ ¯ A R)$
$\Rightarrow m (¯ ¯¯¯¯ ¯ O B - ¯ ¯¯¯¯ ¯ O R) = n (¯ ¯¯¯¯ ¯ O R - ¯ ¯¯¯¯ ¯ O A)$
$\Rightarrow m (¯ b - ¯ r) = n (¯ r - ¯ a)$
$\Rightarrow m ¯ b - m ¯ r = n ¯ r - n ¯ a$
$\Rightarrow m ¯ b + n ¯ a = m ¯ r + n^{2}$
$\Rightarrow ¯ r = \frac{m ¯ b + n ¯ a}{m + n}$ Hence Proved

Updated on:21/07/2023

If the point $¯ ¯ ¯ P (¯ p) = ¯ a + 2 ¯ b$ and the point $A (¯ a)$ divides seg PQ internally in the ratio 2: 3, then : $Q (¯ q)$ =
A $2 ¯ a - ¯ b$
B $¯ b - 2 ¯ a$
C $¯ a - 3 ¯ b$
D $¯ b$
The position vectors of the point which divides internally in the ratio $2 : 3$ the join of the points $2 ¯ a - 3 ¯ b and 3 ¯ a - 2 ¯ b$ , is
A $\frac{12}{5} ¯ a + \frac{13}{5} ¯ b$
B $\frac{12}{5} ¯ a - \frac{13}{5} ¯ b$
C $\frac{3}{5} ¯ a - \frac{2}{5} ¯ b$
D $\frac{2}{5} ¯ a = \frac{3}{5} ¯ b$