If a pair of perpendicular straight lines drawn through the origin forms an isosceles triangle with the line $2 x + 3 y = 6$ , then area of the triangle so formed is 36/13 (b) 12/17 (c) 13/5 (d) 17/14

A

$\frac{36}{13} sq. unit$

B

$\frac{12}{17} sq. unit$

C

$\frac{13}{5} sq. unit$

D

$\frac{17}{13} sq. unit$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

As shown in the following figure, pair of perpendicular lines through origin meets given line 2x+3y=6 at A and B such that AOB is right angled isosceles triangle.

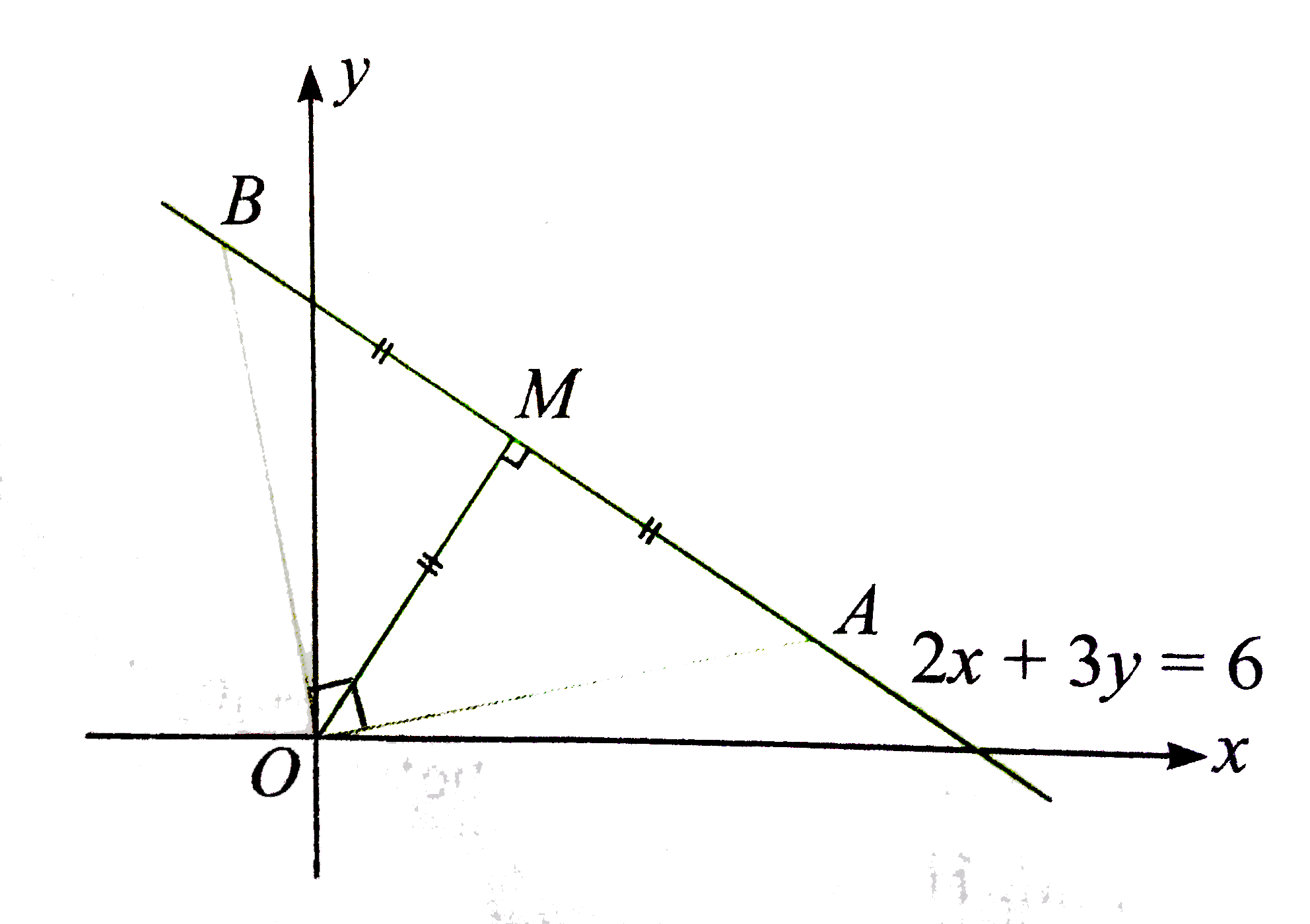
Now, OM=BM=AM, where OM $= \frac{| 0 + 0 - 6 |}{\sqrt{2^{2} + 3^{2}}} = \frac{6}{\sqrt{13}}$
$∴ A B = 2 A M = 2 O M = \frac{12}{\sqrt{13}}$
$∴ Area of triangle A O B = \frac{1}{2} \times \frac{12}{\sqrt{13}} \times \frac{6}{\sqrt{13}} = \frac{36}{13}$ sq. units.

|

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A pair of straight lines drawn through the origin form with the line $2 x + 3 y = 6$ an isosceles right angled triangled then the sides and the area of the triangle thus formed is
A $x - 5 y = 0$
$5 x + y = 0$
$Δ = \frac{36}{13}$
B $3 x - y = 0$
$x + 3 y = 0$
$Δ = \frac{12}{17}$
C $5 x - y = 0$
$x + 5 y = 0$
$D \leq t a = \frac{13}{5}$
DNone
Question 2 - Select One
Drawn from origin are two mutually perpendicular lines forming an isosceles triangle together with the straight line $2 x + y = a$ then the area of this triangle is
A $\frac{a^{2}}{2}$ sq units
B $\frac{a^{2}}{3}$ sq units
C $\frac{a^{2}}{5}$ sq units
DNone of these
Question 3 - Select One
The joint equation of pair of lines through the origin and making an equilateral triangle with the line $3 x + 4 y = 5$ , is
A $39 x^{2} + 48 x y + 11 y^{2} = 0$
B $39 x^{2} - 48 x y + 11 y^{2} = 0$
C $39 x^{2} + 96 x y + 11 y^{2} = 0$
D $39 x^{2} - 96 x y + 11 y^{2} = 0$

CENGAGE-STRAIGHT LINES-Exercise (Single)

Home
Ask Doubt
Profile