If AB is a diameter of a circle and C is any point on the circle, then show that the area of ΔABC is maximum, when it is isosceles.
the area of ΔABC is maximum when it is isosceles
the area of ΔABC is minimum when it is isosceles
the perimeter of ΔABC is maximum when it is isosceles
none of these
The correct Answer is:A
Step by step video & image solution for If AB is a diameter of a circle and C is any point on the circle, then show that the area of DeltaABC is maximum, when it is isosceles. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Chapter Test53videos
CIRCLES
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Section II - Assertion Reason Type12videos
CIRCLES
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test30videos
CARTESIAN PRODUCT OF SETS AND RELATIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test58videos
COMPLEX NUMBERS
Similar Questions
AB is a diameter of a circle and C is any point on the circle. Show that the area of ABC is maximum, when it is isosceles.
View SolutionAB is a diameter of a circle and C is any point on the circumference of the circle. Then (a) the area of ΔABC is maximum when it is isosceles (b) the area of ΔABC is minimum when it is isosceles (c) the perimeter of ΔABC is minimum when it is isosceles (d) none of these
View Solution
Knowledge Check
- Question 1 - Select One
In the figure shown , AB is a diameter of the circle and O is the center of the circle If A=(3,4), then what is the circumference of the circle?
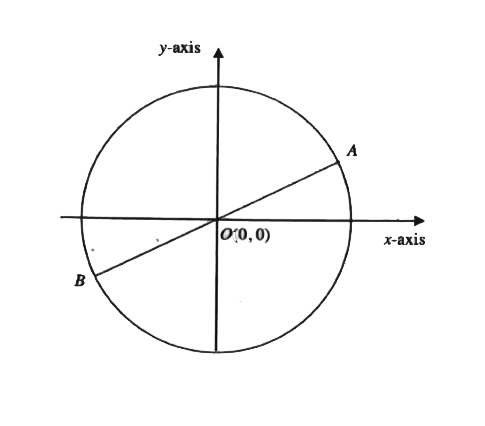 A3B4C4πD10π
A3B4C4πD10π
AB is a diameter of a circle and C is any point on the circumference of the circle. Then a) the area of ABC is maximum when it is isosceles b) the area of ABC is minimum when it is isosceles c) the perimeter of ABC is minimum when it is isosceles d) none of these
View SolutionIf AOB is a diameter of a circle and C is a point on the circle, then AC2+BC2=AB2.
View SolutionThe diameter of a circle is 28 cm. Find its :
areaView SolutionIn figure, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ACD+∠BED.
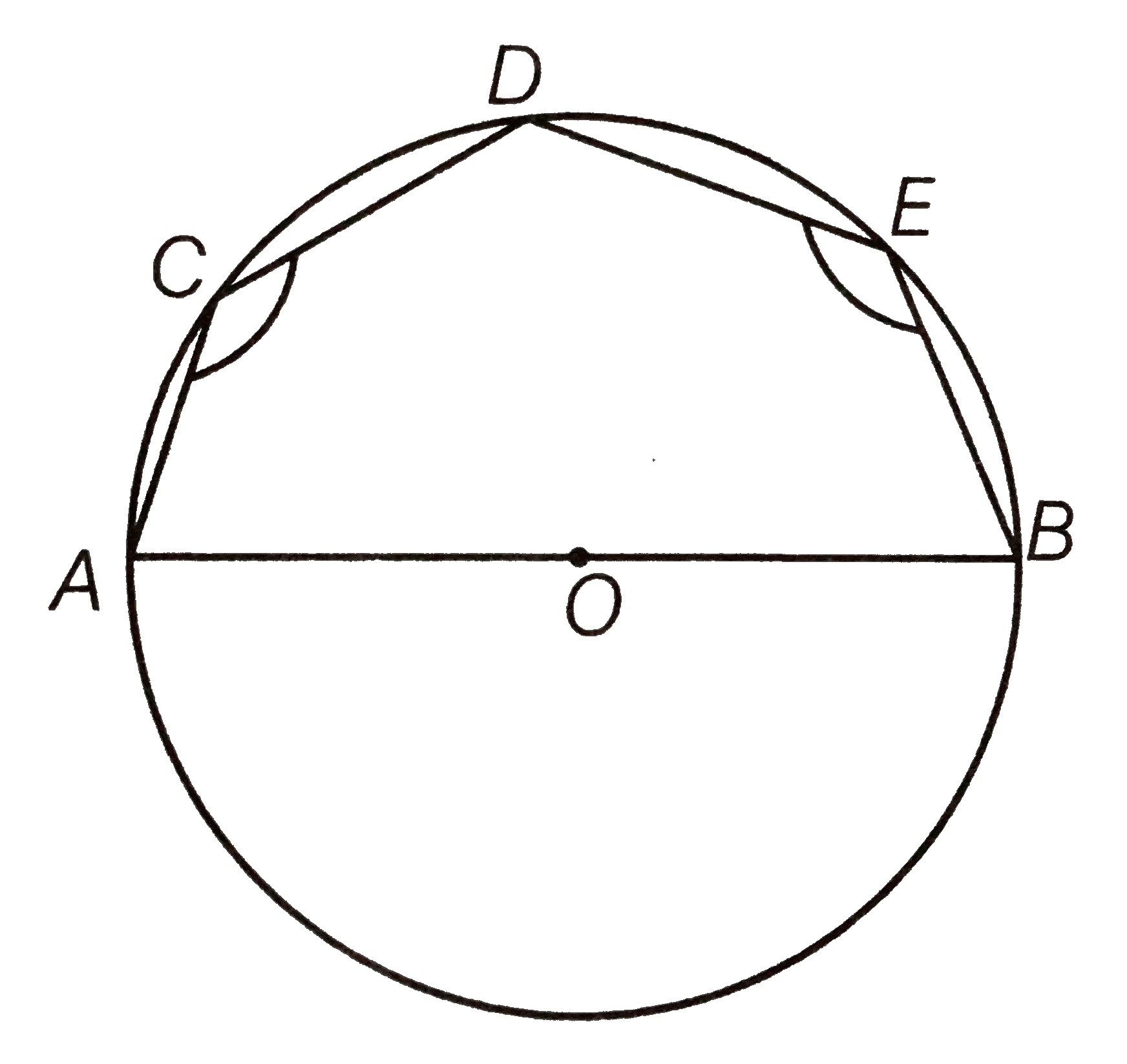 View Solution
View SolutionAn isosceles triangle of vertical angle 2θ is inscribed in a circle of radius a . Show that the area of the triangle is maximum when θ=π6 .
View SolutionAD is a diameter of a circle and AB is a chord. If AD = 34cm, AB = 30cm, the distance of AB form the centre of the circle is
View SolutionA rectangle is inscribed in a semi-circle of radius r with one of its sides on diameter of semi-circle. Find the dimensions of the rectangle so that its area is maximum. Find also the area.
View Solution

