If bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
Given, ABCD is a cyclic quadrilateral.
DP and QB are the bisectors of ∠Dand∠B, respectively.
To prove PQ is the diameter of a circle.
Construction Join QD and QC.
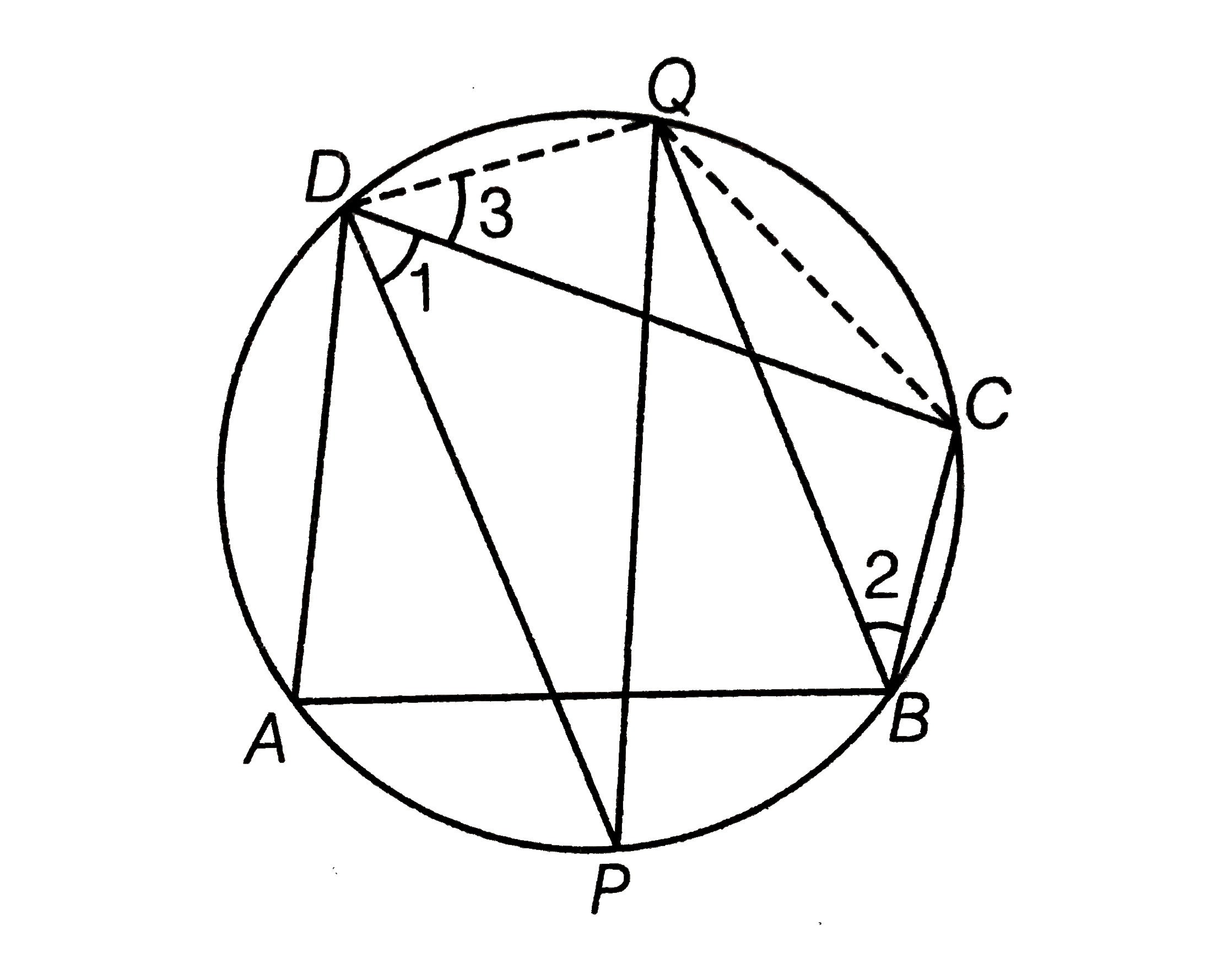
Proof Since, ABCD is a cyclic quadrilateral.
∴∠CDA+∠CBA=180∘
[sum of opposite angles of cyclic quadrilateral is 180∘]
On dividing both sides by 2, we get
12∠CDA+12∠CBA=12×180∘=90∘
⇒∠1+∠2=90∘...(i)
[∠1=12∠CDAand∠2=12∠CBA]
But ∠2=∠3 [ angles in the same segment QC are equal] ...(ii)
∠1+∠3=90∘
From Eqs. (i) and (ii), ∠PDQ=90∘
Hence, PQ is a diameter of a circle, because diameter of the circle.
Subtends a right angle at the circumference.
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLARCHAPTER - CIRCLESEXERCISE - Exercise 10.4
2 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLARCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Areas Of Parallelograms And Triangles
34 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
If the bisectors of the opposite angles ∠Pand∠R of a cyclic quadrilateral PQRS intersect the corresponding cicle at AandB respectively, then AB is a diameter of the circle.
View SolutionIf the bisectors of the opposite angle ∠Pand∠R of a Cyclic quadrilateral PQRS intersect the corresponding circle at A and B respectively; then AB is a diameter of the circle.
View Solution
Knowledge Check
The diagonals AC and BD of a cyclic quadrilateral ABCD intersect each other at the point P. Then,it is always true that
AAP. CP = BP. DPBAP.BP = CP. DPCAP. CD = AB. CPDBP. AB = CD.CPABCD is a cyclic quadrilateral such that AB is the diameter of the circle circumscribing it and ∠ADC=145∘ . What is the measure of ∠(BAC) ?
A50∘B35∘C55∘D40∘ABCD is a cyclic quadrilateral such that AB is the diameter of the circle circumscribing it and ∠ADC=155∘, then what is the measure of ∠BAC?
A35∘B55∘C65∘D45∘
If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices ofthe quadrilateral, prove that it is a rectangle
View SolutionIf diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
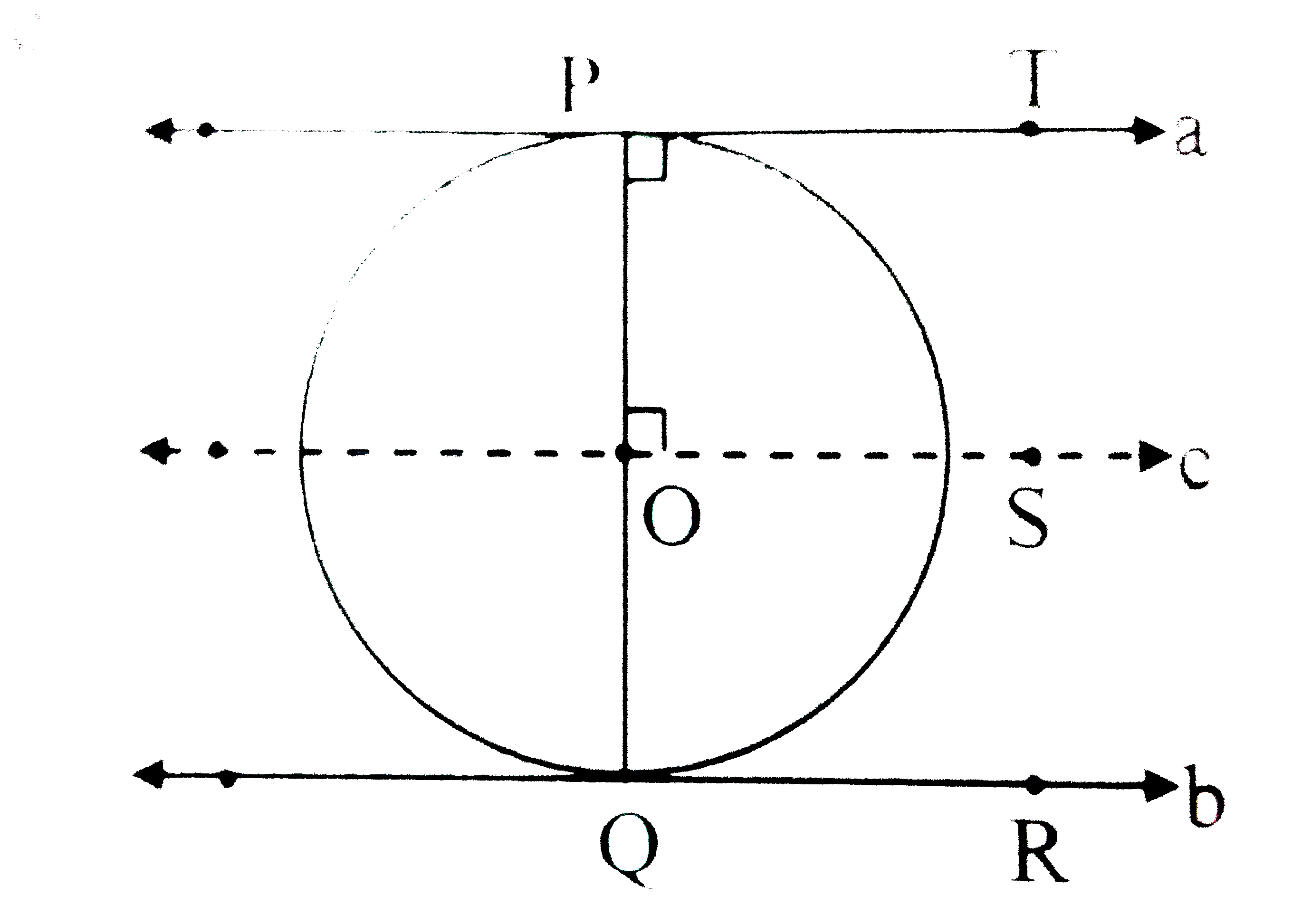
View SolutionPoint O is the centre of a circle . Line a and line b are parallel tangents to the circle at P and Q. Prove that segment PQ is a diameter of the circle.
View SolutionABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and angle ADC=146∘.∠BAC is equal to:
View SolutionABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC=140∘, then ∠BAC is equal to
View Solution
