If mode of a series exceeds its mean by 12, then mode exceeds the median by (a) 4 (b) 8 (c) 6 (d) 10
To solve the problem step by step, we will use the relationships between mode, median, and mean.
Step 1: Understand the given information
We are given that the mode exceeds the mean by 12. This can be expressed mathematically as:
Mode−Mean=12
Step 2: Use the relationship between mode, median, and mean
We know from statistical relationships that:
Mode=3×Median−2×Mean
Step 3: Substitute the expression for mode into the equation
Substituting the expression for mode into the equation from Step 1, we get:
(3×Median−2×Mean)−Mean=12
This simplifies to:
3×Median−3×Mean=12
Step 4: Factor out the common term
Factoring out the common term gives us:
3×(Median−Mean)=12
Step 5: Solve for the difference between median and mean
Dividing both sides by 3, we find:
Median−Mean=123=4
Step 6: Express mode in terms of median
Now, we can express the difference between mode and median. We know:
Mode=3×Median−2×Mean
Rearranging gives us:
Mode−Median=2×Median−2×Mean
This simplifies to:
Mode−Median=2×(Median−Mean)
Step 7: Substitute the value of median - mean
From Step 5, we found that:
Median−Mean=4
Substituting this into the equation gives:
Mode−Median=2×4=8
Conclusion
Thus, the mode exceeds the median by:
Mode−Median=8
The correct answer is (b) 8.
---
We are given that the mode exceeds the mean by 12. This can be expressed mathematically as:
Mode−Mean=12
We know from statistical relationships that:
Mode=3×Median−2×Mean
Substituting the expression for mode into the equation from Step 1, we get:
(3×Median−2×Mean)−Mean=12
This simplifies to:
3×Median−3×Mean=12
Factoring out the common term gives us:
3×(Median−Mean)=12
Dividing both sides by 3, we find:
Median−Mean=123=4
Now, we can express the difference between mode and median. We know:
Mode=3×Median−2×Mean
Rearranging gives us:
Mode−Median=2×Median−2×Mean
This simplifies to:
Mode−Median=2×(Median−Mean)
From Step 5, we found that:
Median−Mean=4
Substituting this into the equation gives:
Mode−Median=2×4=8
Thus, the mode exceeds the median by:
Mode−Median=8
Topper's Solved these Questions
Similar Questions
If for a moderately skewed distribution, mode = 60 and mean = 66, then median =
View SolutionFind mean, median and mode of the following data :
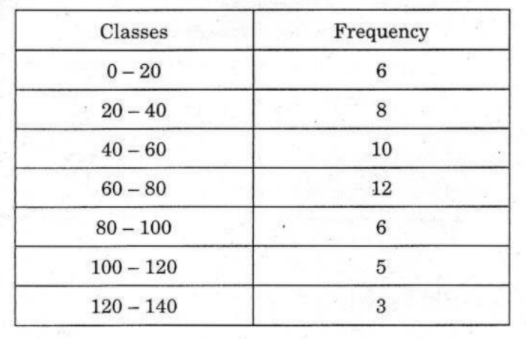 View Solution
View SolutionFind the mean, median and mode of the following data :
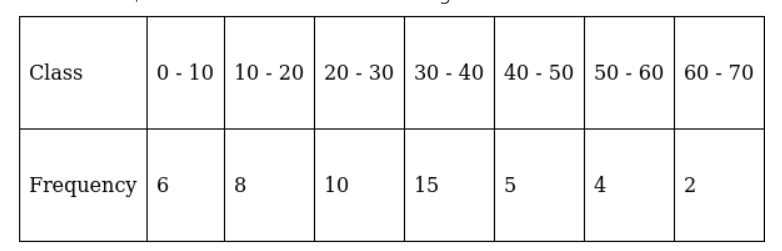 View Solution
View SolutionFind the mean, median and mode of the following data
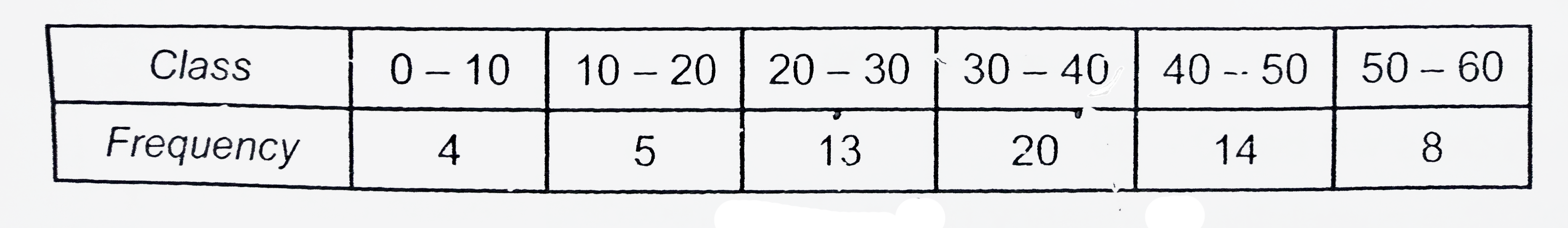 View Solution
View SolutionThe arithmetic mean of two positive numbers a and b exceeds their geometric mean by 32 and the geometric mean exceeds their harmonic mean by 65. If a+b=α and |a−b|=β, then the value of 10βα is equal to
View SolutionWrite the empirical relation between mean, mode and median.
View SolutionIf the difference of mode and median of a data is 24, then the difference of median and mean is (a) 12 (b) 24 (c) 8 (d) 36
View SolutionConsider two positive numbers a and b. If arithmetic mean of a and b exceeds their geometric mean by 3/2, and geometric mean of aand b exceeds their harmonic mean by 6/5 then the value of a2+b2 will be
View SolutionThe arithmetic mean and mode of a data are 24 and 12 respectively, then its median is (a) 25 (b) 18 (c) 20 (d) 22
View SolutionFor a normal distribution if the mean is M, mode is M0 and median is Md, then
View Solution

