If Sr=√r+√r+√r+√r+.....∞,r>0, then which of the following is/are correct.
A
Sr,S6,S12,S20, are in AP
B
S4,S9,S16 are irrational
C
(2S4−1)2,(2S5−1)2,(2S6−1)2 are in AP
D
S2,S12,S56 are in GP
Video Solution
Text Solution
The correct Answer is:A, B, C, D
∴Sr=√r+√r+√r+√......∞=√r+S
S2r−Sr−r=0
∴Sr=1+√(1+4r)2 [∴r>0]Sr−r=0
Alternate (a) S2,S6,S12,S20i.e.2,3,4,5 are in AP.
Alternate(b) S4,S9,S16,i.e.1+√172,1+√372,1+√652 are irrationals.
Alternate (c) (2S4−1)2,(2S5−1)2,(2S6−1)2i.e.,17,21,25 are in AP.
Alternate (d) S2,S12,S56i.e.,2,4,8 are in GP.
|
Topper's Solved these Questions
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise For Session 1
5 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise For Session 2
11 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
Similar Questions
Knowledge Check
If s=t+√r3q, what is the value of r in terms of q, s and t ?
A3√qs2−qtB3√s2−2st−t2qC3√qs2−2qst+qt2D√qs2+2qst−t23
n∑r=1sin−1(√r−√r−1√r(r+1)) is equal to
View SolutionIntegrate the following w.r.t x
√x
View SolutionDifferentiate the following w.r.t x
√sinx
View SolutionIntegrate the following w.r.t x
√1−sinx
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
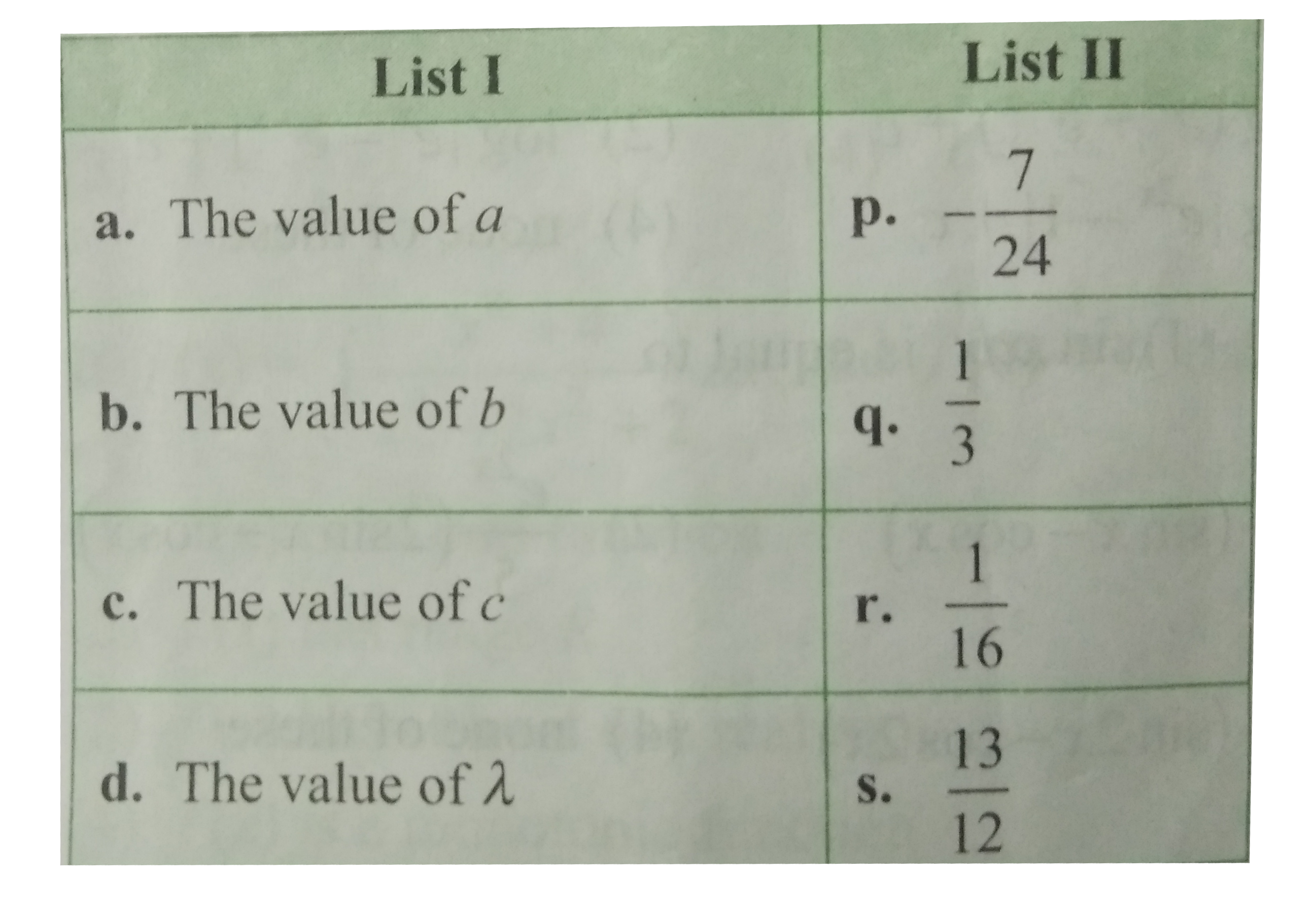
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View SolutionDifferentiate √sin−1√x w.r.t. x, (0<x<1)
View SolutionIf r is positive real number such that 4√r−14√r=4, then find the value of 6√r+16√r.
View Solution
