If the diagonals of a parallelogram are equal and intersect at right angles, then the parallelogram is a square.

Video Solution

Text Solution

Verified by Experts

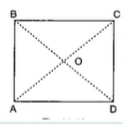
Consider a parallelogram ABCD
In ABCD the diagonals are AC and BD both intersect at right angle at O. So In triangle AOB and BOC
AO=OC (Diagonals are equal so half are also
So now in triangle ACB and ADC and $C = 90^{\circ}$
$A n g \leq A O B = A n g \leq B O C (90^{\circ})$
OB=OB(Common)
So by SAS Triangle AOB-BOC so AB=BC Similarly DOC and AOD Are also congruent so AD=DC
SO IF, AD=DC and AB=BC
So, We can conclude AD=AB also
AD=BC
Angle ADC-ABC(cpct)
AB=DC
SO IF ABC AND ADC ARE CONGRUENT so We can conclude that Angle A and B are equal
So If A=B,So $A + B = 180$
$A + A = 180$ (Because both are equal)
$2 A = 180 A = \frac{180}{2}$
$A = 90^{\circ}$
SO, B is also $90^{\circ}$
So If C=D
So $C + D = 180^{\circ}$
$C + C = 180$ (Because both are equal)
$2 C = 180$
$C = \frac{180}{2}$
So, D is also $90^{\circ}$
So all angle of square are $90^{\circ}$ hence proved it is a square.

|

Updated on:21/07/2023

Class 9 MATHS QUADRILATERALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If diagonal of a parallelogram are of same length and cut at right angle, then the quadrilateral is
Aequiperpendicular
Bsquare
Crhombus
Dractangle

RD SHARMA-QUADRILATERALS-All Questions

Home
Ask Doubt
Profile