IF the radius of a circle is doubled, by how much will the area ?
2 times
3 times
4 times
5 times
The correct Answer is:C
To solve the question "If the radius of a circle is doubled, by how much will the area increase?", we can follow these steps:
1. Understand the formula for the area of a circle:
The area A of a circle is given by the formula:
A=πr2
where r is the radius of the circle.
Hint: Remember the formula for the area of a circle, which involves the radius squared.
2. Calculate the area with the original radius:
Let the original radius be r. The area of the circle with this radius is:
A1=πr2
Hint: Substitute the original radius into the area formula to find the initial area.
3. Determine the new radius when it is doubled:
If the radius is doubled, the new radius becomes:
r′=2r
Hint: Think about how doubling the radius affects its value.
4. Calculate the area with the new radius:
Now, we can find the area of the circle with the new radius:
A2=π(2r)2
Simplifying this gives:
A2=π(4r2)=4πr2
Hint: Remember to square the entire term when substituting the new radius into the area formula.
5. Compare the two areas:
Now we compare the original area A1 and the new area A2:
- Original area: A1=πr2
- New area: A2=4πr2
Hint: Look at how the new area relates to the original area by factoring.
6. Calculate the increase in area:
The increase in area can be calculated as:
Increase in Area=A2−A1=4πr2−πr2=(4−1)πr2=3πr2
Hint: Subtract the original area from the new area to find the increase.
7. Determine the factor of increase:
To find out by how much the area has increased in terms of the original area, we can express the new area in relation to the original area:
A2A1=4πr2πr2=4
Hint: Dividing the new area by the original area shows how many times larger the new area is.
Final Answer:
The area of the circle increases by a factor of 4 when the radius is doubled.
The area A of a circle is given by the formula:
A=πr2
where r is the radius of the circle.
Let the original radius be r. The area of the circle with this radius is:
A1=πr2
If the radius is doubled, the new radius becomes:
r′=2r
Now, we can find the area of the circle with the new radius:
A2=π(2r)2
Simplifying this gives:
A2=π(4r2)=4πr2
Now we compare the original area A1 and the new area A2:
- Original area: A1=πr2
- New area: A2=4πr2
The increase in area can be calculated as:
Increase in Area=A2−A1=4πr2−πr2=(4−1)πr2=3πr2
To find out by how much the area has increased in terms of the original area, we can express the new area in relation to the original area:
A2A1=4πr2πr2=4
The area of the circle increases by a factor of 4 when the radius is doubled.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - WARMUP EXERCISE10videos
PERIMETER AND AREA
- BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - EXERCISE 17.115videos
PERIMETER AND AREA
- BOOK - ICSECHAPTER - PERCENTAGE, PROFIT AND LOSSEXERCISE - REVISION EXERCISE26videos
PERCENTAGE, PROFIT AND LOSS
- BOOK - ICSECHAPTER - PROBABILITYEXERCISE - TRY THIS14videos
PROBABILITY
Similar Questions
If the radius of a circle is tripled, what is the ratio of the area of half the original circle to the area of the whole new circle? (Area of a circle =πr2, where r = radius)
View SolutionThe radius of a circle is 14 cm. Find the radius of the circle whose area is double of the area of the circle.
View Solution
Knowledge Check
- Question 1 - Select One
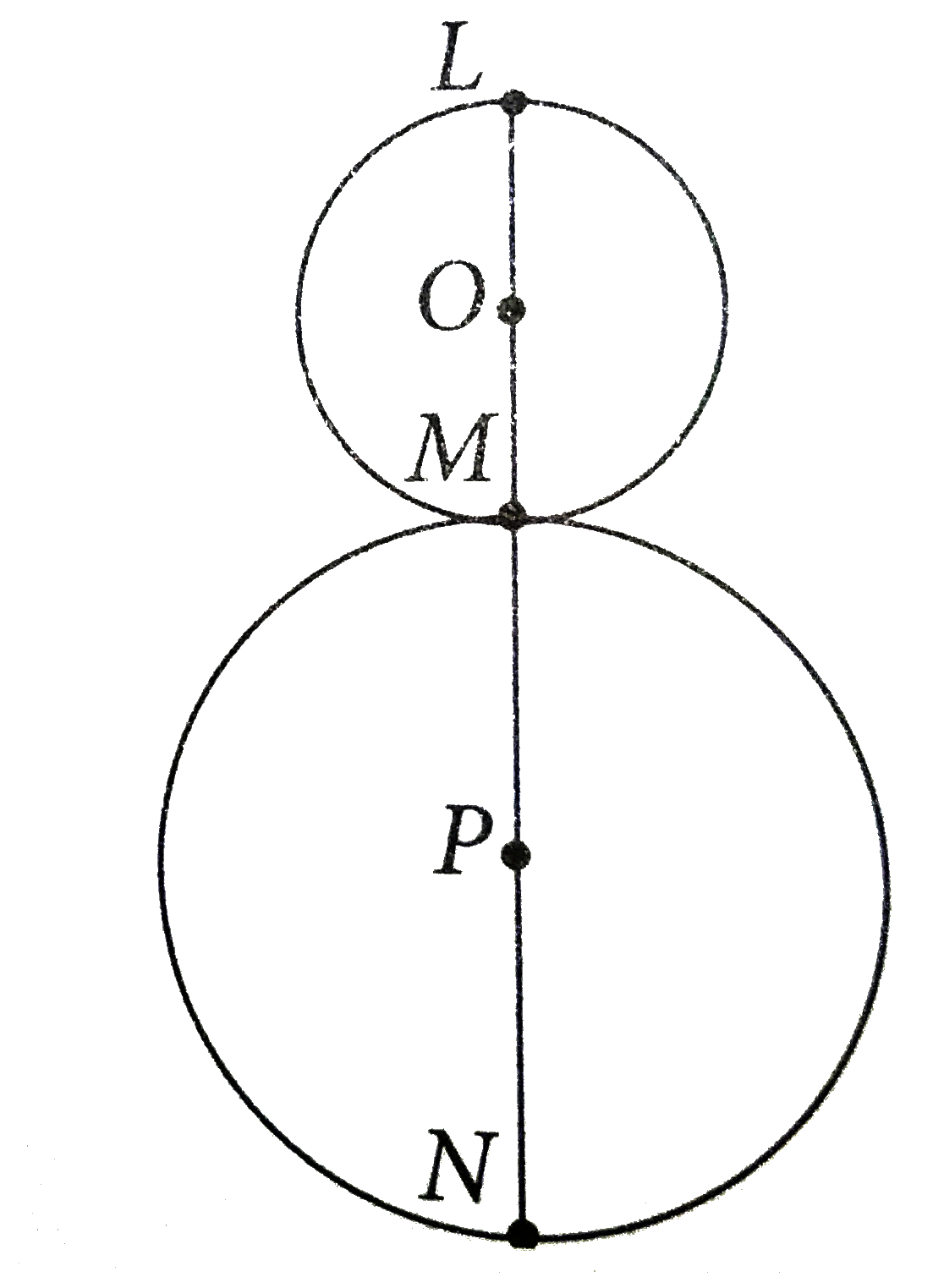
In the figure above, LM is 13 of LN. If the radius of the circle with center P is 6, what is the area of the area of the circle with center O?A4πB9πC12πD18π
Rectangle ABCD is inscribed in a circle. If the radius of the circle is 2 and ¯¯¯¯¯¯CD=2, what is the area of the shaded region ?
 View Solution
View SolutionIf the pressure of a gas is doubled and the temperature is tripled, by how much will the mean free path of a gas molecule in a vessel change?
View SolutionIf the radius of a cylinder is doubled and height is halved, the volume will be doubled.
View SolutionAssertion If radius of cylinderical wire is doubled, then this wire can bear four times stress.
Reason By doubling the radius, area of cross-section will become four times.View SolutionIf the radius of a circle is increased three times, by how many times will the circumference increase ?
View SolutionThe radius of a circle is 7. What is the area?
View SolutionThe radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having area equal to the sum of the areas of the two circles.
View Solution

