If two chords AB and CD of a circle AYDZBWCX intersect at right angles, then prove that arc CXA+arc DZB=arc AYD+arc BWC =semi-circle.
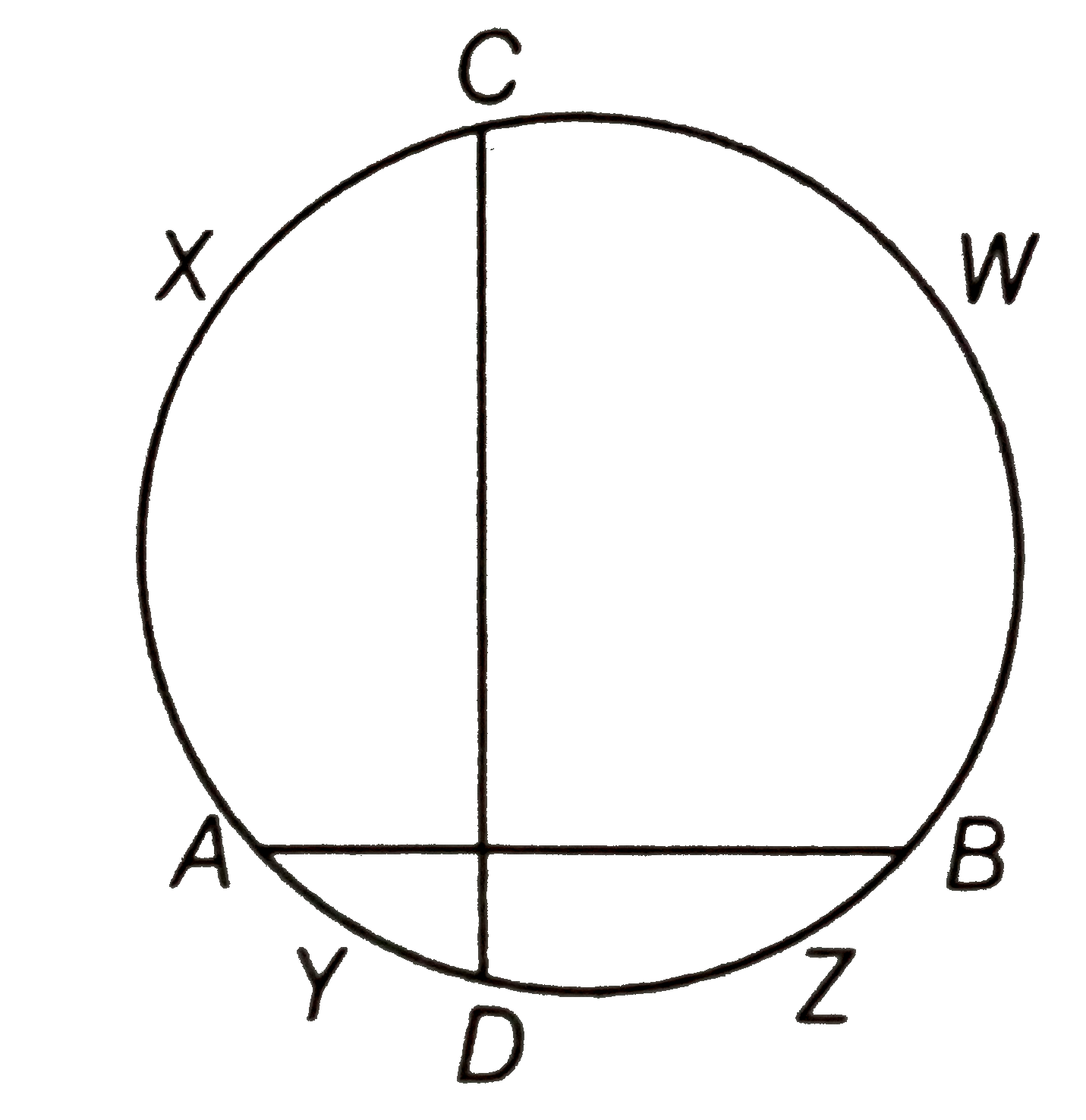
Given In a circle AYDZBWCX, two chords AB and CD intersect at tight angles.
To prove arc CXA+arc DZB=arc AYD+ arc BWC =Semi-circle.
Construction Draw a diameter EF parallel to CD having centre M.
Proof Since, CD∣∣EF
∴ AreEC=arcFD ...(i)
Also, arc ECXA=arc EWB [ symmetrical about diameter of a circle]
and arc AF=arc BF ....(ii)
We know that, arc ECXAYDF =Semi-circle
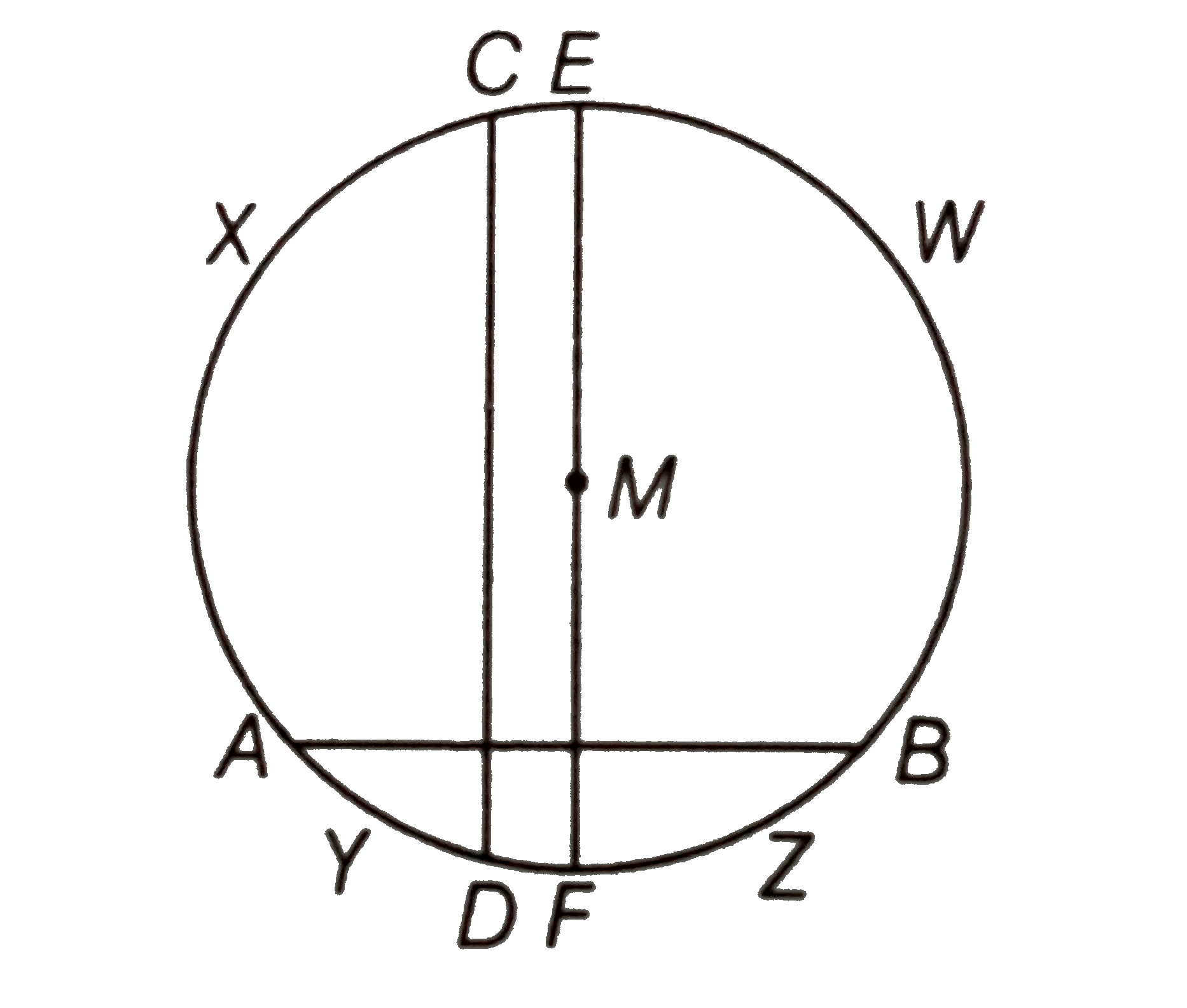
arc EA+arc AF=Semi-circle
⇒ arc EC+arc CXA + arc FB=Semi-circle [from Eq. (ii)]
⇒ arc DF+arc CXA+arc FB =Semi-circle [from Eq. (i)]
⇒ arc DF+arc FB+ arc CXA= Semi-circle
⇒ arc DZB+arc C×A=Semi-circle
We know that, circle divides itself in two semi-circles, therefore the remaining portion of the circle is also equal to the semi- circle.
∴ arc AYD+ arc BWC=Semi-circle.
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLARCHAPTER - CIRCLESEXERCISE - Exercise 10.4
2 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLARCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Areas Of Parallelograms And Triangles
34 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
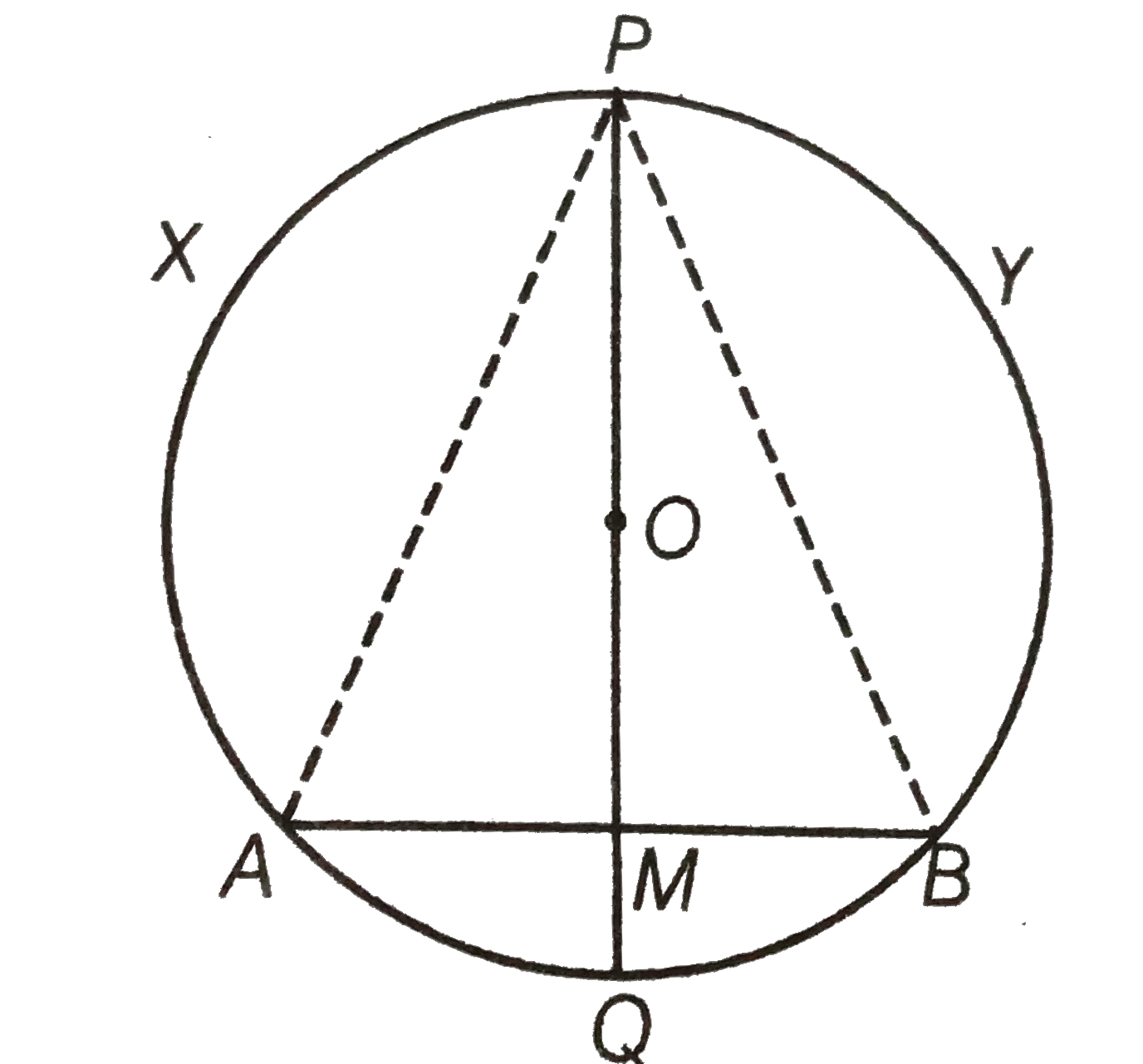
If the perpendicular bisector of a chord AB of a circle PXAQBY intersects the circle at P and Q, prove that arc PXA≅arc PYB.
View SolutionIf the perpendicular bisector of a chord AB of a circle PXAQBY intersects the circle at P and Q, prove that arc PXA≅arc PYB.
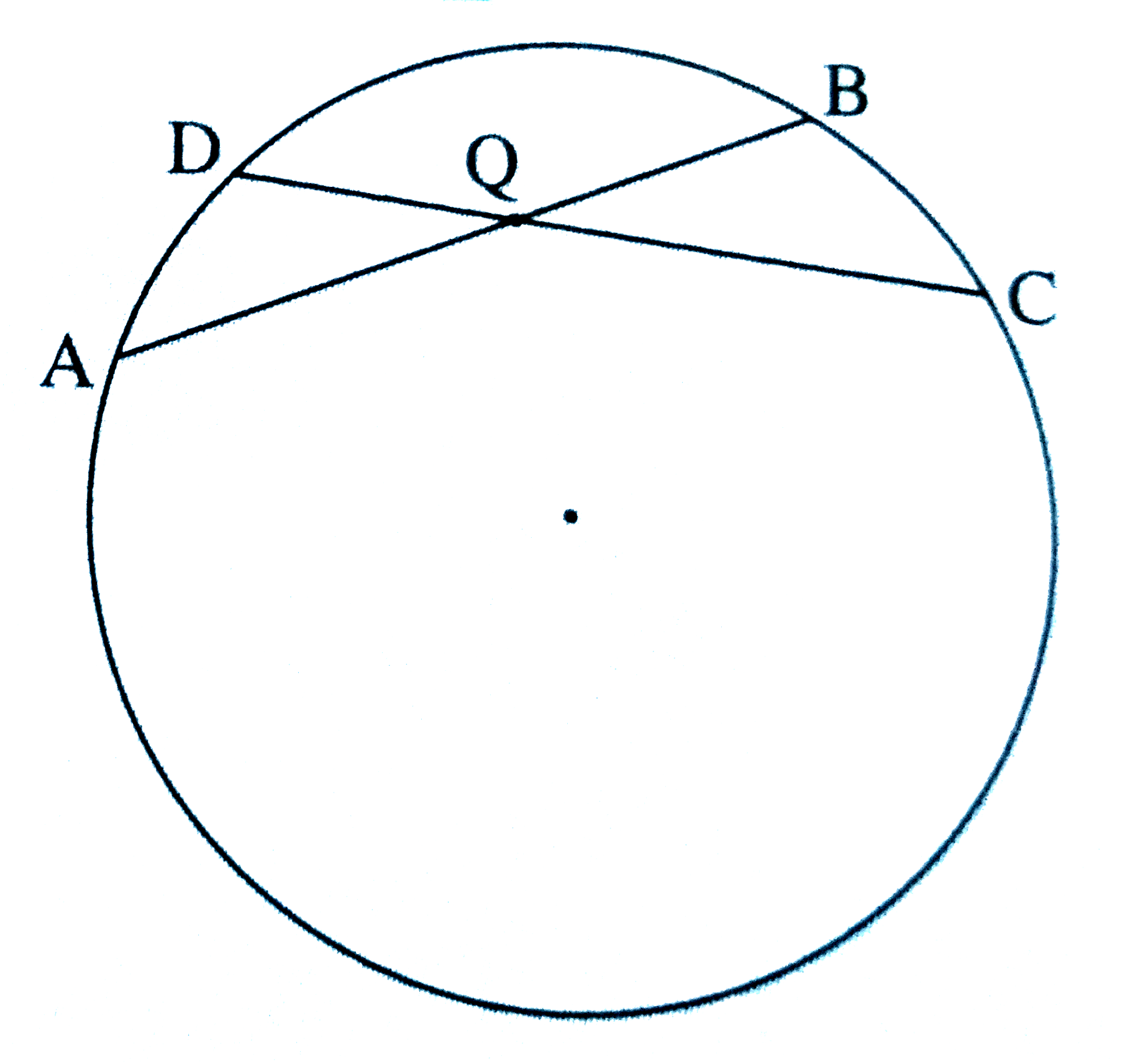
View SolutionChords AB and CD of a circle intersect in point Q in interior of a circle. If m(arc AD)=25∘ and m(arc BC)=31∘, find ∠BQC.
View SolutionThe arc of a circle subtending a right angle at any point of the circle in its alternate segment is a semi-circle.
View SolutionThe arc of a circle subtending a right angle at any point of the circle in its alternate segment is a semi-circle.
View SolutionMajor arc……...minor arc of the circle.
View SolutionChords AB andCD of a circle with centre O are congruent. If m(arc AXB) =120∘ then what is the m(arc( CYD).
View SolutionIn the figure, chord AB ≅ chord CD
Prove that ,
ar AC ≅ arc BD
View SolutionTwo chords AB and CD intersect at P inside the circle . Prove that the sum of the angles substended by the arcs AC and BD at the centre O is equal to twice the angle APC.
View SolutionIf two arcs of a circle are congruent; then corresponding chords are equal
View Solution
