If two triangles are similar; prove that the ratio of corresponding sides is equal to the ratio of corresponding altitudes.
Video Solution
Text Solution
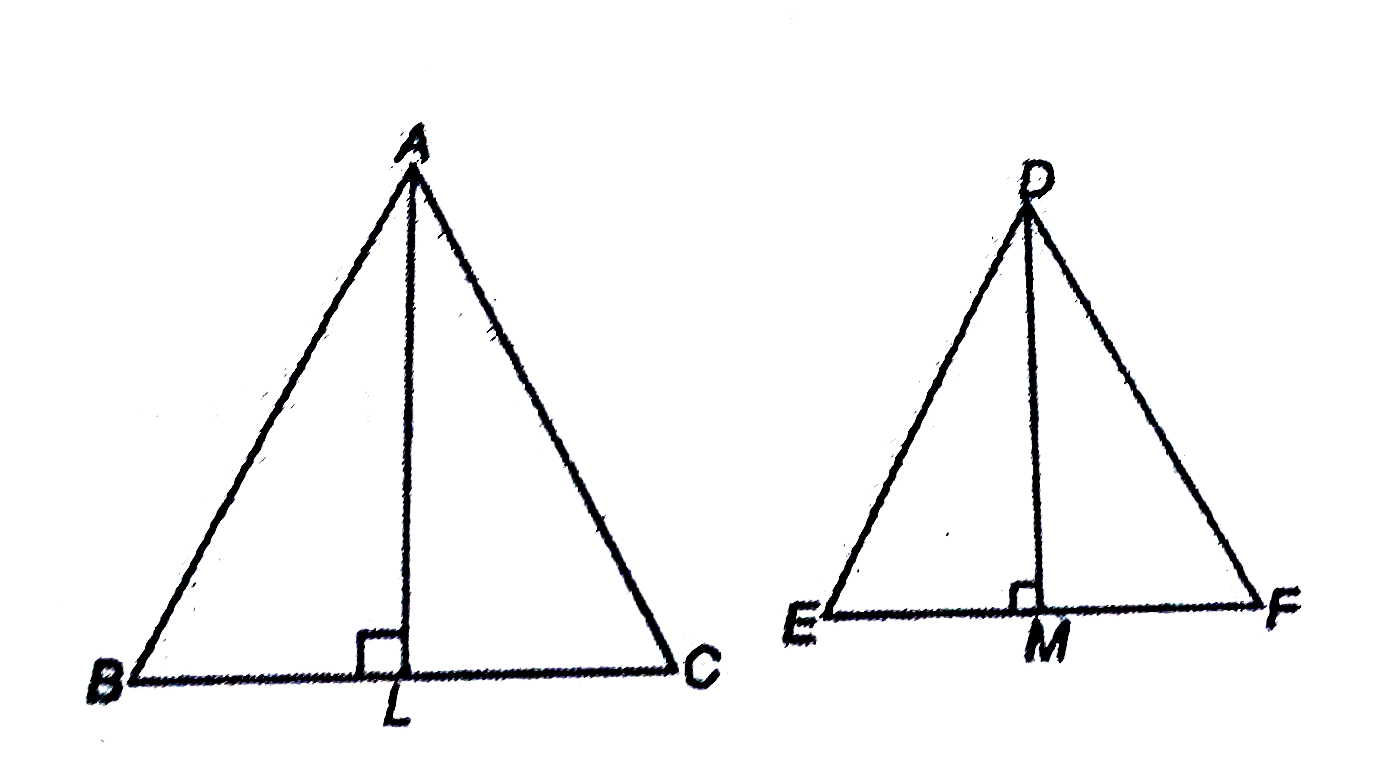
Given : △ABC~△DEFAL⊥BCandDM⊥EF
To prove : ar(△ABC)ar(△DEF)=AL2DM2
proof: in △ALBand△DME,
∠B=∠E(given△ABC~△DEF)
∠ALB=∠DME=90∘ (each)
△ABL~△DEM (by AA similarity)
⇒ ABDE=ALDM..(1)
Now , ar(△ABC)ar(△DEF)=AB2DE2...(2)
( the ratio of areas of two similar triangles is equal to ratio of squares of the corresponding sides)
Form (1) and (2) we have
ar(△ABC)ar(△DEF)=AL2DM2 Hence proved.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar14videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6a23videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions5videos
STATISTICS
- BOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
Knowledge Check
- Question 1 - Select One
If area of two similar triangle are equal then ratio of their corresponding altitude is.
A1:1B1:√2C1:2DCannot be determined - Question 2 - Select One
If the ratio of the altitudes of two triangles be 3:4 and the ratio of their corresponding areas be 4 : 3, then the ratio of their corresonding lengths of bases is
A1:1B16:9C1:2D2:1 - Question 3 - Select One
If the angles of a triangle are in the ratio 1:2:3,the corresponding sides are in the ratio
A2:3:1B√3:2:1C2:√3:1D1:√3:2
Two triangles are similar if their corresponding sides are ………….
View SolutionProve that the ratio of the perimeters of two similar triangles is the same as the ratio of their corresponding sides.
View SolutionTheorem 6.3 : If in two triangles, corresponding angles are equal, then their corresponding sides are in the same ratio (or proportion) and hence the two triangles are similar.
View SolutionIf ratio of areas of two triangles are 64:121 , then the ratio of corresponding perimeter is
View SolutionArea of two similar triangles are in the ratio of 5:3 then the ratio of their corresponding sides is :
View Solution

