If x is the number of tails in three tosses of a coin, then determine the standard deviation of X.

Video Solution

Text Solution

Verified by Experts

Given that, random variable X is the number of tails in three toses of the coin.
So, X=0,1,2,3.

$\Rightarrow P (X = x) =^{n} C_{x} {(p)}^{x} q^{n - x}$
Where n=3,p $= 1 / 2$ andx=0,1,2,3

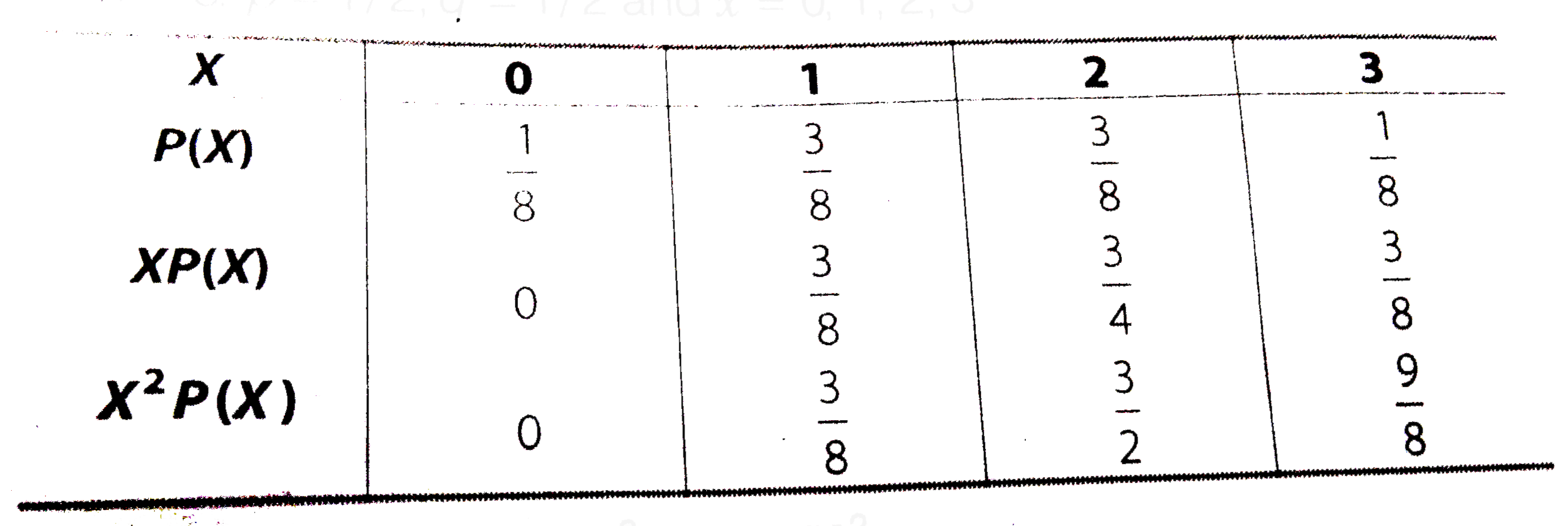
We know that, Var(X)= $E (X^{2}) - {[E (X)]}^{2}$
where, $E (X^{2}) = \sum_{i = 1}^{n} x_{i}^{2} P (x_{i}) and E (X) = \sum_{i = 1}^{n} x_{i} P (x_{i})$
$∴ E (X^{2}) = \sum_{i = 1}^{n} x_{i}^{2} P (X_{i}) = 0 + \frac{3}{8} + \frac{3}{2} + \frac{9}{8} = \frac{24}{8} = 3$
and ${[E (X)]}^{2} = {[\sum_{i = 0}^{n} x_{i}^{2} P (x_{i})]}^{2} = {[0 + \frac{3}{8} + \frac{3}{4} + \frac{3}{8}]}^{2} = {[\frac{12}{8}]}^{2} = \frac{9}{4}$
$∴ V a r (X) = 3 - \frac{9}{4} = \frac{3}{4}$
and standard deviation of X= $\sqrt{V a r (X)} = \sqrt{\frac{3}{4}} = \frac{\sqrt{3}}{2}$

Updated on:21/07/2023