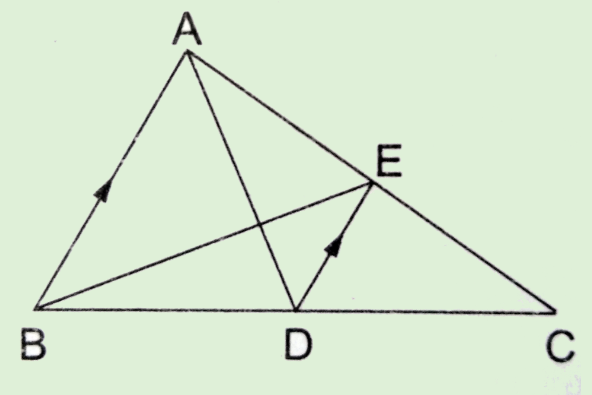
In is the median through and is the mid-point of . produced meets in (Figure). Prove that
Construction
DK||BF
In E is the midpoint od AD and EF||DK
F is the midpoint of AK
AF=FK-(1)
K is the mid point of FC
FK=KC-(2)
FK=KC=KC
AC=AF+FK+KC
AC=AF+AF+AF=3AF
.
Topper's Solved these Questions
Similar Questions
In ABC,AD is the median through A and E is the mid-point of AD . BE produced meets AC in F (Figure). Prove that AF=13AC.
View SolutionIn Figure, AD is the median and DE∣∣AB . Prove that BE is the median.
View SolutionE is the mid-point of the median AD of ΔABC. Line segment BE meets AC at point F when produce, prove that AF=13AC.
View SolutionABCD is a parallelogram. AD is a produced to E so that DE=DC and EC produced meets AB produced in F. Prove that BF=BC.
View SolutionIn Figure, ABCandBDE are two equilateral triangles such that D is the mid-point of BC,AE intersects BC in F Prove that: ar(FED)=18ar(AFC)
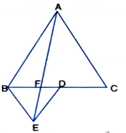View SolutionIn Figure, ABC and BDE are two equilateral triangls such that D is the mid-point of BC.AE intersects BC in F. Prove that: ar ( BFE)= 2 ar (EFD)
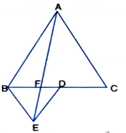View SolutionIn Figure, ABCandBDE are two equilateral triangles such that D is the mid-point of BC,AE intersects BC in F
Prove that: ar(BFE)=ar(AFD)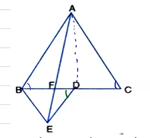
View SolutionD is the mid-point of side BC of ABC and E is the mid-point of BD. If O is the mid-point of AE , prove that ar(BOE)=18ar(ABC)
View SolutionIn Figure, ABCandBDE are two equilateral triangles such that D is the mid-point of BC,AE intersects BC in F
Prove that: ar(BDE)=14ar(ABC)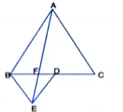
View SolutionIn Figure, AD and BE are medians of ABC and BE∣∣DF . Prove that CF=14AC
Consider △ABC Since AD is Median
⇒BD=BC⋯(1)
Since BE is Median⇒AE=EC⋯(2)
Consider △BECSince BE∣∣DF
By Basic Proportionality theorm, ⇒BDDC=EFFC
From (1)⇒CF=EF
⇒CF=12AE
From (2)⇒CF=14ACView Solution