In a lot of 12 Microwave ovens, there are 3 defective units. A person has ordered 4 of these units and since each is identically packed, the selection will be random. What is the probability that i. all 4 units are good. ii. Exactly 3 units are good, iii. at least 2 units are good.
To solve the problem, we need to calculate the probabilities for three different scenarios regarding the selection of microwave ovens from a lot of 12, which includes 3 defective units and 9 good units.
Step 1: Identify Total and Good Units
- Total microwave ovens = 12
- Defective units = 3
- Good units = 12 - 3 = 9
Step 2: Calculate Total Ways to Choose 4 Units
The total number of ways to choose 4 units from 12 can be calculated using the combination formula:
Total ways=(nr)=n!r!(n−r)!
Where n is the total number of items, and r is the number of items to choose.
So, we calculate:
Total ways=(124)=12!4!(12−4)!=12×11×10×94×3×2×1=495
Step 3: i. Probability that All 4 Units are Good
To find the probability that all 4 selected units are good, we calculate the number of ways to choose 4 good units from the 9 available:
Ways to choose 4 good units=(94)=9!4!(9−4)!=9×8×7×64×3×2×1=126
Now, the probability is:
P(All 4 good)=Ways to choose 4 goodTotal ways=126495=1455
Step 4: ii. Probability that Exactly 3 Units are Good
To find the probability that exactly 3 units are good, we need to choose 3 good units from 9 and 1 defective unit from 3:
Ways to choose 3 good units=(93)=9!3!(9−3)!=9×8×73×2×1=84
Ways to choose 1 defective unit=(31)=3
Thus, the total ways to choose exactly 3 good and 1 defective unit is:
Total ways=84×3=252
Now, the probability is:
P(Exactly 3 good)=252495=2855
Step 5: iii. Probability that At Least 2 Units are Good
To find the probability that at least 2 units are good, we can calculate the probabilities of the complementary events (0 good units and 1 good unit) and subtract from 1.
1. Probability of 0 good units (all defective):
Ways to choose 0 good units=(90)=1
Ways to choose 4 defective units=(34)=0(impossible)
So, P(0 good)=0.
2. Probability of 1 good unit:
Ways to choose 1 good unit=(91)=9
Ways to choose 3 defective units=(33)=1
Thus, total ways to choose 1 good and 3 defective:
Total ways=9×1=9
Now, the probability is:
P(1 good)=9495=155
Now, we calculate P(At least 2 good):
P(At least 2 good)=1−P(0 good)−P(1 good)=1−0−155=5455
Final Answers
- i. Probability that all 4 units are good: 1455
- ii. Probability that exactly 3 units are good: 2855
- iii. Probability that at least 2 units are good: 5455
- Total microwave ovens = 12
- Defective units = 3
- Good units = 12 - 3 = 9
The total number of ways to choose 4 units from 12 can be calculated using the combination formula:
Total ways=(nr)=n!r!(n−r)!
Where n is the total number of items, and r is the number of items to choose.
Total ways=(124)=12!4!(12−4)!=12×11×10×94×3×2×1=495
To find the probability that all 4 selected units are good, we calculate the number of ways to choose 4 good units from the 9 available:
Ways to choose 4 good units=(94)=9!4!(9−4)!=9×8×7×64×3×2×1=126
Now, the probability is:
P(All 4 good)=Ways to choose 4 goodTotal ways=126495=1455
To find the probability that exactly 3 units are good, we need to choose 3 good units from 9 and 1 defective unit from 3:
Ways to choose 3 good units=(93)=9!3!(9−3)!=9×8×73×2×1=84
Ways to choose 1 defective unit=(31)=3
Thus, the total ways to choose exactly 3 good and 1 defective unit is:
Total ways=84×3=252
Now, the probability is:
P(Exactly 3 good)=252495=2855
To find the probability that at least 2 units are good, we can calculate the probabilities of the complementary events (0 good units and 1 good unit) and subtract from 1.
Ways to choose 0 good units=(90)=1
Ways to choose 4 defective units=(34)=0(impossible)
So, P(0 good)=0.
Ways to choose 1 good unit=(91)=9
Ways to choose 3 defective units=(33)=1
Thus, total ways to choose 1 good and 3 defective:
Total ways=9×1=9
Now, the probability is:
P(1 good)=9495=155
P(At least 2 good)=1−P(0 good)−P(1 good)=1−0−155=5455
- i. Probability that all 4 units are good: 1455
- ii. Probability that exactly 3 units are good: 2855
- iii. Probability that at least 2 units are good: 5455
Topper's Solved these Questions
Similar Questions
A class consists of 10 boys and 8 girls. Three students are selected at random. What is the probability that the selected group has (i) all boys? (ii) all girls? (iii) at least one girl?
View SolutionA box contains 100 bulbs 20 of which are defective. 10 bulbs are selected for inspection. Find the probability that: i. all 10 are defective ii. all 10 are good ii. at least one is defective iv. none is defective.
View SolutionA basket contains 20 apples and 10 oranges out of which 5 apples and 3 oranges are defective. If a person takes out 2 at random what is the probability that either both are apples or both are good?
View SolutionA basket contains 20 apples and 10 oranges out of which 5 apple and 3 orange are defective. If a person takes out 2 at random. What is the probability that either both are apples or both are good?
View SolutionBulbs are packed in cartons each containing 40 bulbs, seven hundred cartons were examined for defective bulbs and the results are given in the following table.
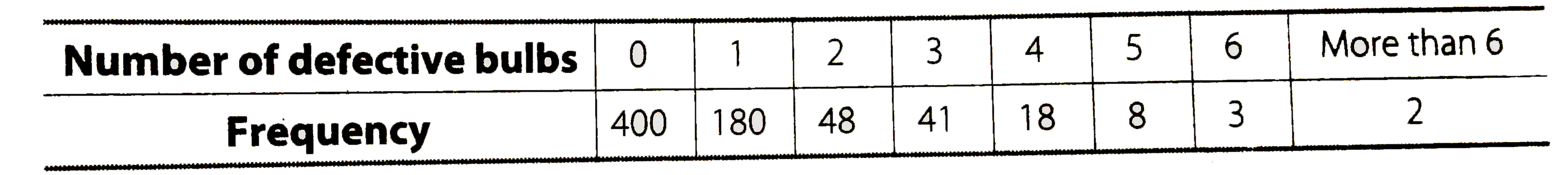
One carton was selected at random. What is the probability that it has
i) no defective bulb?
ii) defective bulbs from 2-6?
iii) defective bulbs less than 4?View SolutionA room has 3 lamps . From a collection of 10 light bulbs of which 6 are no good , a person selects 3 at random and puts them in a socket . What is the probability , that he will have light ?
View SolutionIn a factory manufacturing certain spare parts 2% of the output is found defective . If spares are packed in 200 units , the probability that there are 4 defective spares in a pack is
View SolutionTwo vectors have magnitudes 3 unit and 4 unit respectively. What should be the angel between them if the magnitude of the resultant ils a. 1 unit, b. 5 unit and c. 7 unit.
View SolutionFind the equation of the straight line parallel to Y - axis and at a distance (i) 3 units to the right (ii) 2 units to the left
View SolutionIn a bundle of 50 shirts, 44 are good, 4 have minor defects and 2 have major defects. What is the probability that :
(ii) it is acceptable to a trader who rejects only a shirt with major defects ?View Solution

