In a right-angled triangle ABC, right angled at B, an altitude BD is dropped on AC. If AB=8 and BC=6, what is the length of AD?
2.4
3.6
4.8
6.4
The correct Answer is:D
To find the length of AD in the right-angled triangle ABC where AB=8 and BC=6, we can follow these steps:
Step 1: Calculate the length of AC using the Pythagorean theorem.
In triangle ABC, since it is a right triangle with AB and BC as the two legs, we can use the Pythagorean theorem:
AC2=AB2+BC2
Substituting the known values:
AC2=82+62
Calculating the squares:
AC2=64+36=100
Taking the square root:
AC=√100=10
Step 2: Set up the relationship using cosine.
Let θ be the angle ∠ABC. We can express cosθ in two different ways.
First, in triangle ABC:
cosθ=ABAC=810=0.8
Next, in triangle ABD:
cosθ=ADAB=AD8
Step 3: Equate the two expressions for cosθ.
Since both expressions equal cosθ, we can set them equal to each other:
AD8=810
Step 4: Solve for AD.
Cross-multiplying gives:
AD⋅10=8⋅8
Calculating the right side:
10AD=64
Dividing both sides by 10:
AD=6410=6.4
Conclusion
The length of AD is 6.4.
---
In triangle ABC, since it is a right triangle with AB and BC as the two legs, we can use the Pythagorean theorem:
Let θ be the angle ∠ABC. We can express cosθ in two different ways.
Since both expressions equal cosθ, we can set them equal to each other:
Cross-multiplying gives:
The length of AD is 6.4.
Topper's Solved these Questions
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Grib-In
32 Videos
- ADDITIONAL TOPICS IN MATH BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST
24 Videos
- COMPLEX NUMBERSBOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE
6 Videos
Similar Questions
Knowledge Check
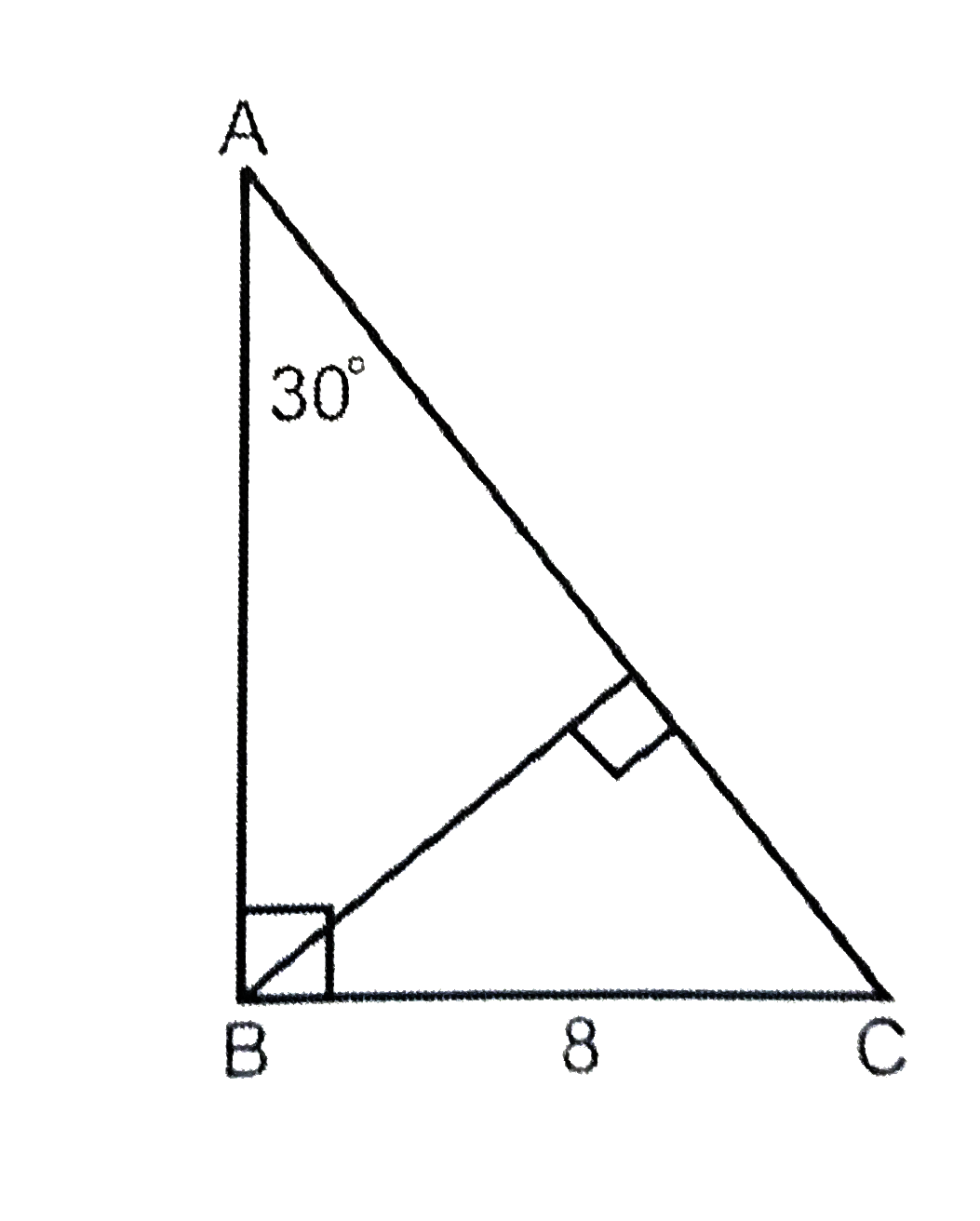
In the figure shown, ABC is a triangle, right angled at B. Through B, a line is drawn perpendicular to AC which meets AC in D. What is the length of BD?A4√3B8√3C2√3D4√3
In the adjoining figure, ABC is a triangle right-angled at vertex A and AD is altitude.
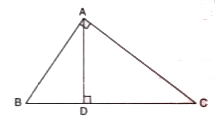
If BD = 3.6 cm and CD = 6.4 cm, find the length of AD.
View SolutionConstruct a right-angled triangle ABC, right-angled at A, with hypotenuse BC = 10 cm long and one of the sides AB = 6 cm long. Also, find the midpoint D of the hypotenuse. Join AD and measure AD.
View SolutionConstruct a right-angled triangle ABC, right-angled at A, with hypotenuse BC = 6.3 cm long and one of the sides AB = 4.8 cm long. Also, find the mid-point D of the hypotenuse. Join AD and measure AD.
View SolutionABC is a right-angled triangle with the right angle at vertex B. BD is the altitude through B.given BD=12cm and AD=9cm.
Name the triangles which are similar to triangle ADB (Proof not required).
View SolutionΔABC is right-angled at C. If AC=5cmandBC=12cm find the length of AB.
View SolutionIn a triangle ABC, right-angled at B, side BC = 20 cm and angle A= 30°. Find the length of AB.
View SolutionConstruct a right-angled triangle ABC, right-angled at A, with hypotenuse BC = 7.5 cm and one of the sides AB = 5.5 cm. Also, from the point A, drop a perpendicular on BC, intersecting BC at D. Find AB : AD.
View Solution
