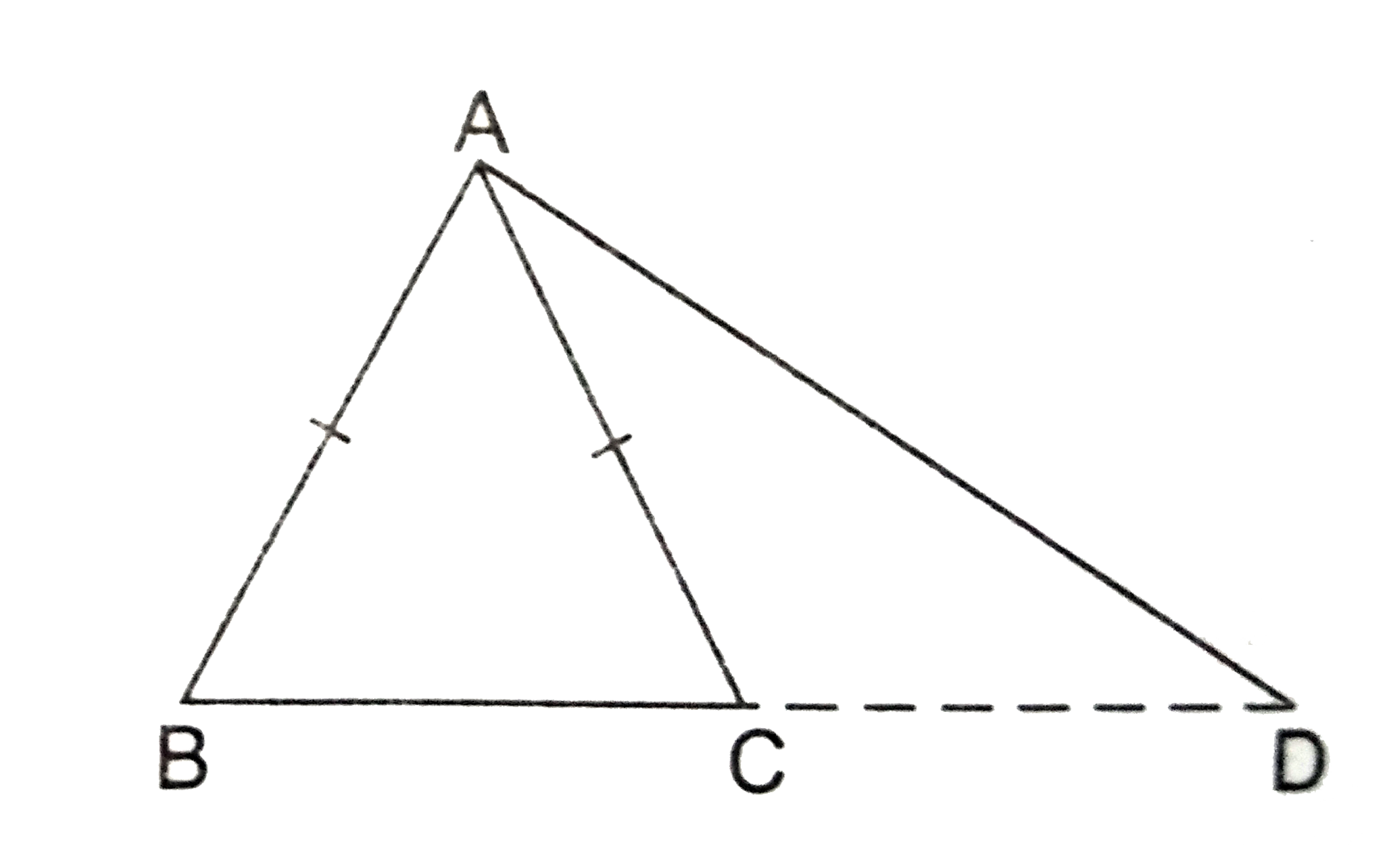
In ΔABC,AB<AC. Then, ........ holds good
∠A<∠B
∠B<∠C
∠C<∠A
∠C<∠B
The correct Answer is:A, B, C
To solve the problem, we need to analyze the relationship between the sides and angles of triangle ABC given that AB<AC.
1. Identify the Given Information:
We are given a triangle ABC where AB<AC.
2. Understand the Relationship:
In any triangle, the sides are directly related to the angles opposite to them. Specifically, if one side is shorter than another, the angle opposite the shorter side is also smaller than the angle opposite the longer side.
3. Apply the Triangle Inequality Theorem:
Since AB<AC, we can conclude that the angle opposite side AB (which is angle C) is less than the angle opposite side AC (which is angle B). Therefore, we can write:
∠C<∠B
4. Conclude the Result:
Thus, the relationship that holds true in triangle ABC is:
If AB<AC, then ∠C<∠B.
Final Answer:
The statement that holds good is: If AB<AC, then ∠C<∠B.
---
We are given a triangle ABC where AB<AC.
In any triangle, the sides are directly related to the angles opposite to them. Specifically, if one side is shorter than another, the angle opposite the shorter side is also smaller than the angle opposite the longer side.
Since AB<AC, we can conclude that the angle opposite side AB (which is angle C) is less than the angle opposite side AC (which is angle B). Therefore, we can write:
∠C<∠B
Thus, the relationship that holds true in triangle ABC is:
If AB<AC, then ∠C<∠B.
The statement that holds good is: If AB<AC, then ∠C<∠B.
Topper's Solved these Questions
- TRIANGLESBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - TRIANGLESEXERCISE - SUMS TO ENRICH REMEMBER
9 Videos
- TRIANGLESBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - TRIANGLESEXERCISE - SKILL TESTING EXERCISE
19 Videos
- SURFACE AREAS AND VOLUMESBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - MULTIPLE CHOICE QUESTIONS
18 Videos
Similar Questions
Knowledge Check
In ΔABC,AB=AC. The poins D and E are on BC. Such that AD = AE. If∠ACE=48∘ and ∠DAE=32∘, then measure of ∠BAD is
A26∘B22∘C24∘D29∘ABC is an isosceles triangle with AB = AC. Side BA is produced to D such that AB=AD. Find the ∠BCD:
A100∘B60∘C80∘D90∘In △ABC, if AB=AC and ∠BAC=40∘ ,then the measure of ∠B is
त्रिभुज ABC में, यदि AB=AC है तथा ∠BAC=40∘ है, तो कोण B का मान क्या होगा ?A50∘B60∘C40∘D70∘
In ΔABC,AB=6cm,BC=7cm and AC=5cm .The area of ΔABC is
View SolutionIn A ΔABC, AB = 10 AC = 18 AD is angle bisector If area of ΔABC is 28 . find area of ADB ?
View SolutionIn an isoscles ΔABC,AB=ACandD is a point on BC. Prove that AB2−AD2=BD⋅CD.
View SolutionIn ΔABC, if ∠A=80∘ and AB=AC, then ∠B=____________.
View SolutionIn ΔABC, if ∠A<∠B<45∘, then ABC is a/ an __________________ triangle.
View Solution