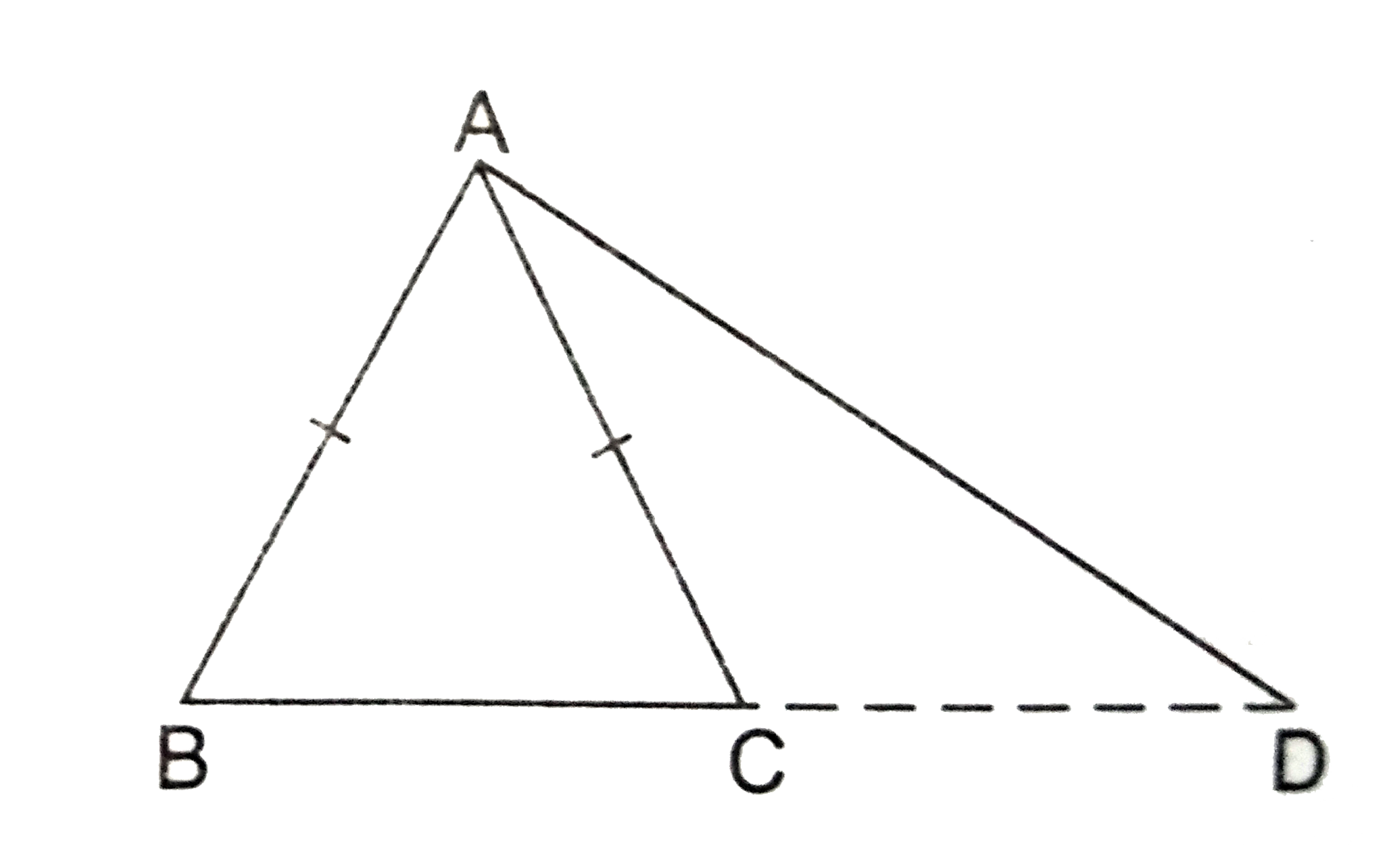
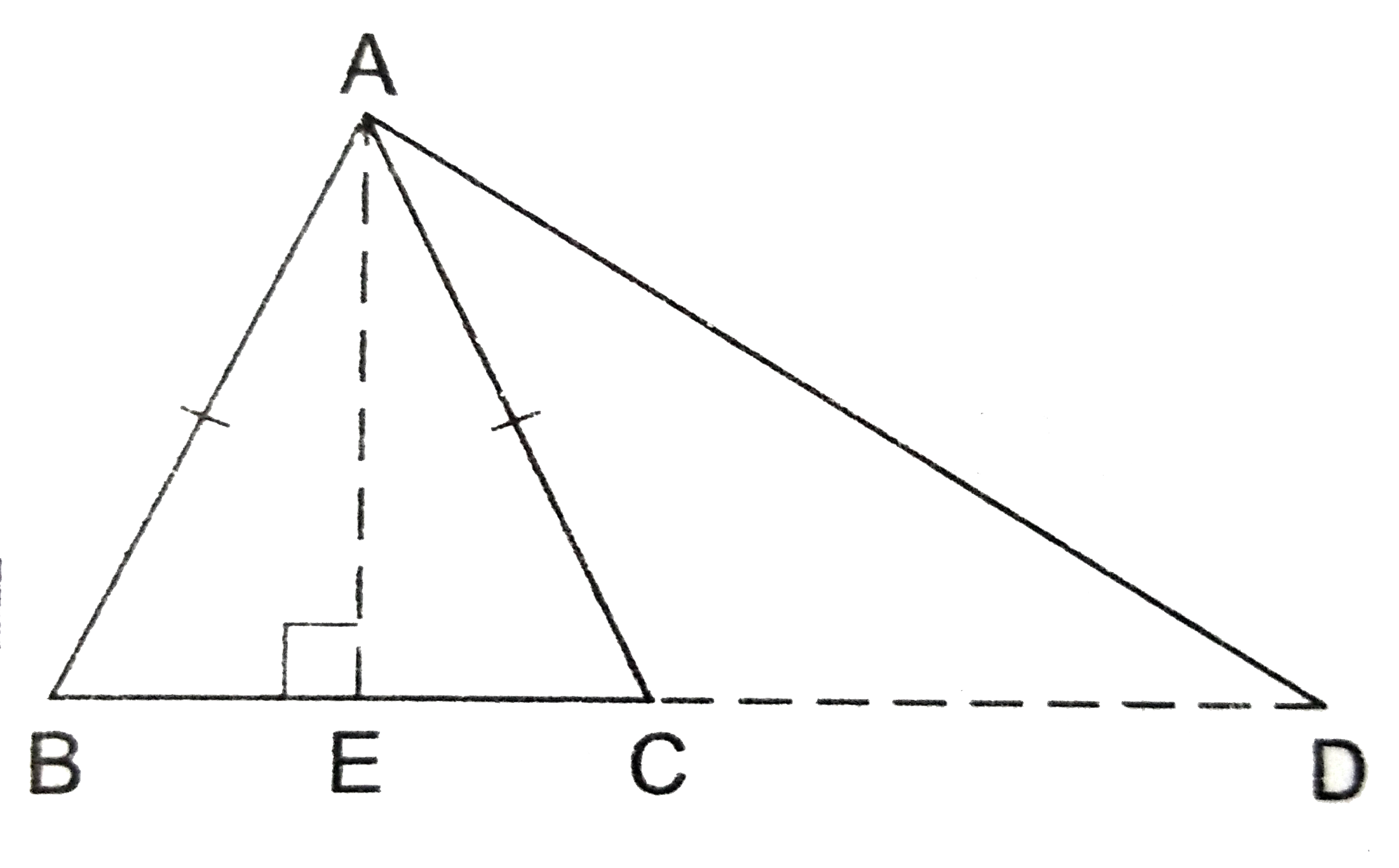
In $Δ A B C, A B = A C$ . Side BC is produced to D. Prove that $(A D^{2} - A C^{2}) = B D \cdot C D$ .

Video Solution

Text Solution

Verified by Experts

Draw $A E ⊥ B C$ . Then BE=CE
$A D^{2} = A E^{2} + D E^{2} and A C^{2} = A E^{2} + C E^{2}$
$\Rightarrow (A D^{2} - A C^{2}) = D E^{2} - C E^{2}$
$= (D E + C E (D E - C E)$
$= (D E + B E) (D E - C E) [∴ C E = B E]$
$= B D \cdot C D$

|

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7E
30videos
TRIANGLES
BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Multiple Choice Questions (Mcq)
53videos
TRIANGLES
BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7C
13videos
TRIANGLES
BOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27videos
T-RATIOS OF SOME PARTICULAR ANGLES
BOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20videos
TRIGONOMETRIC IDENTITIES

Similar Questions

Knowledge Check

Question 1 - Select One
ABC is an isosceles triangle with AB = AC. Side BA is produced to D such that $A B = A D$ . Find the $∠ B C D$ :
A $100^{\circ}$
B $60^{\circ}$
C $80^{\circ}$
D $90^{\circ}$
Question 2 - Select One
$Δ A B C, A B = A C$ and BA is produced to D such that AC = AD. Then the $∠ B C D$ is
A $100^{\circ}$
B $60^{\circ}$
C $80^{\circ}$
D $90^{\circ}$

RS AGGARWAL-TRIANGLES-Exercise 7D

Home
Ask Doubt
Profile