In ΔPQR,Pq=PR and S is the mid-point of PQ. A line drawn from S parallel to QR, intersects the line PR at T. Prove that PS = PT.
To prove that PS = PT in triangle PQR where PQ = PR and S is the midpoint of PQ, we will follow these steps:
1. Identify the Triangle and Midpoint:
- We have triangle PQR with PQ = PR.
- Let S be the midpoint of PQ.
Hint: Remember that the midpoint divides a line segment into two equal parts.
2. Draw a Line Parallel to QR:
- Draw a line ST from point S that is parallel to QR. This line intersects PR at point T.
Hint: When two lines are parallel, certain properties about angles and segments can be used.
3. Apply the Converse of the Midpoint Theorem:
- According to the Converse Midpoint Theorem, if a line segment is drawn from the midpoint of one side of a triangle parallel to another side, it will bisect the third side.
- Since S is the midpoint of PQ and ST is parallel to QR, T must be the midpoint of PR.
Hint: Recall that the converse of the midpoint theorem states that if a line is drawn parallel to one side of a triangle from the midpoint of another side, it divides the third side into two equal segments.
4. Establish Relationships:
- Since T is the midpoint of PR, we can write:
- PT = TR (because T divides PR into two equal segments).
Hint: Use the property of midpoints to establish equality of segments.
5. Use Given Information:
- We know that PQ = PR. Since S is the midpoint of PQ, we can say:
- PS = SQ (because S divides PQ into two equal segments).
Hint: Midpoints create equal segments on the lines they divide.
6. Relate PS and PT:
- Since PQ = PR, we can express this in terms of the segments:
- PS + SQ = PT + TR.
- Given that PS = SQ and PT = TR, we can conclude:
- PS = PT.
Hint: Use the fact that equal segments can be substituted for each other in equations.
7. Conclusion:
- Therefore, we have proved that PS = PT.
Final Statement:
Thus, we have shown that in triangle PQR, with S as the midpoint of PQ and ST parallel to QR, it follows that PS = PT.
---
- We have triangle PQR with PQ = PR.
- Let S be the midpoint of PQ.
- Draw a line ST from point S that is parallel to QR. This line intersects PR at point T.
- According to the Converse Midpoint Theorem, if a line segment is drawn from the midpoint of one side of a triangle parallel to another side, it will bisect the third side.
- Since S is the midpoint of PQ and ST is parallel to QR, T must be the midpoint of PR.
- Since T is the midpoint of PR, we can write:
- PT = TR (because T divides PR into two equal segments).
- We know that PQ = PR. Since S is the midpoint of PQ, we can say:
- PS = SQ (because S divides PQ into two equal segments).
- Since PQ = PR, we can express this in terms of the segments:
- PS + SQ = PT + TR.
- Given that PS = SQ and PT = TR, we can conclude:
- PS = PT.
- Therefore, we have proved that PS = PT.
Thus, we have shown that in triangle PQR, with S as the midpoint of PQ and ST parallel to QR, it follows that PS = PT.
Topper's Solved these Questions
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Revision Exercise (very Short Answer Questions)
10 Videos
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Revision Exercise (short Answer Questions )
10 Videos
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8a
29 Videos
- PROBABILITYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - PROBABILITYEXERCISE - Revision Exercise (very Short Answer /short Answer Questions)
10 Videos
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - Revision Exercise
12 Videos
Similar Questions
If S is the mid-point of side QR of a ΔPQR, then prove that PQ+PR=2PS.
View SolutionP is the mid-point of the side CD of a parallelogram ABCD. A line through C parallel to PA intersects AB at Q and DA produced at R. Prove that DA = AR and CQ = QR.
View SolutionIf Q is a point on the side SR of a triangle ΔPSR such that PQ=PR then prove that PS>PQ
View SolutionConsider the circle x2+y2−8x−18y+93=0 with the center C and a point P(2,5) out side it. From P a pair of tangents PQ and PR are drawn to the circle with S as mid point of QR. The line joining P to C intersects the given circle at A and B. Which of the following hold (s)
View SolutionThe line drawn through the mid-point of one side of a triangle, parallel to another side, intersects the third side at its mid-point.
View SolutionIn the adjoining figure, ΔPQR is formed by the sides PQ, QR and RP which are drawn parallel to sides AB,BC and CA respectively of ΔABC. Prove that
PQ+QR+RP=2(AB+BC+CA).

View SolutionConsider a curve ax2+2hxy+by2=1 and a point P not on the curve. A line drawn from the point P intersect the curve at points Q and R. If he product PQ.PR is (A) a pair of straight line (B) a circle (C) a parabola (D) an ellipse or hyperbola
View SolutionIn trapezium ABCD, AB∣∣DC and L is the mid-point of BC. Through L, a line PQ∣∣AD has been drawn which meets AB in P and DC produced in Q. Prove that ar (ABCD) = ar (APQD).
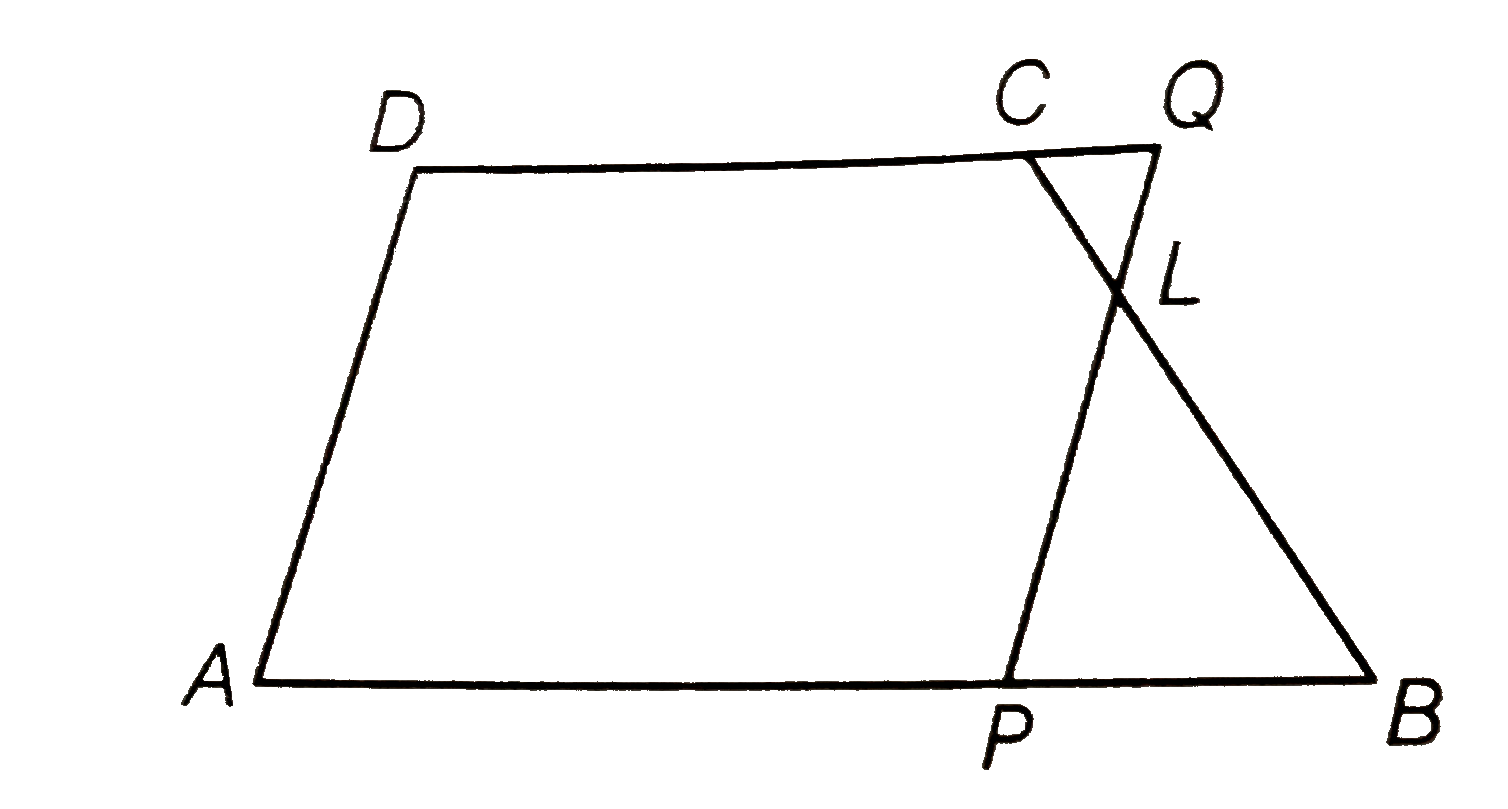
View SolutionIn ΔPQR,PQ=PR.A is a point in PQ and B is a point in PR , so that
QR = RA = AB = BP
Find the vlaue of ∠Q.
View SolutionM and N are mid point on sides QR and PQ respectively of △PQR, right-anggled at Q. Prove that :
4PM2=4PQ2+QR2.
View Solution
