In fig. 10, A,B,C and D are four points, and no three points are collinear. AC and BD intersect at O. there are eight triangle that you can observe. Name all the triangles.
To solve the problem of naming all the triangles formed by the points A, B, C, and D, where AC and BD intersect at O, we can follow these steps:
1. Identify the Points and Intersection:
We have four points: A, B, C, and D. The lines AC and BD intersect at point O.
2. Visualize the Diagram:
Imagine or draw the quadrilateral formed by points A, B, C, and D, with diagonals AC and BD intersecting at point O.
3. List the Triangles Formed:
To find the triangles, we will consider combinations of the points that include the intersection point O. The triangles can be formed by selecting any three points from the set {A, B, C, D, O}.
4. Write Down Each Triangle:
- Triangle AOD
- Triangle AOB
- Triangle BOC
- Triangle COD
- Triangle ADC
- Triangle ABC
- Triangle DAB
- Triangle BCD
5. Count the Triangles:
After listing, we can count the triangles to ensure we have eight distinct triangles.
Final List of Triangles:
1. Triangle AOD
2. Triangle AOB
3. Triangle BOC
4. Triangle COD
5. Triangle ADC
6. Triangle ABC
7. Triangle DAB
8. Triangle BCD
We have four points: A, B, C, and D. The lines AC and BD intersect at point O.
Imagine or draw the quadrilateral formed by points A, B, C, and D, with diagonals AC and BD intersecting at point O.
To find the triangles, we will consider combinations of the points that include the intersection point O. The triangles can be formed by selecting any three points from the set {A, B, C, D, O}.
- Triangle AOD
- Triangle AOB
- Triangle BOC
- Triangle COD
- Triangle ADC
- Triangle ABC
- Triangle DAB
- Triangle BCD
After listing, we can count the triangles to ensure we have eight distinct triangles.
1. Triangle AOD
2. Triangle AOB
3. Triangle BOC
4. Triangle COD
5. Triangle ADC
6. Triangle ABC
7. Triangle DAB
8. Triangle BCD
Topper's Solved these Questions
Similar Questions
Take four distinct points, A,B,C and D no three of which are collinear.
Name the line segments.
View SolutionTake four distinct point A,B ,C and D , out of which three points are collinear
Name the line segments.
View SolutionTake four distinct point A,B ,C and D , out of which three points are collinear
Find the number of lines that can be drawn passing through these points.
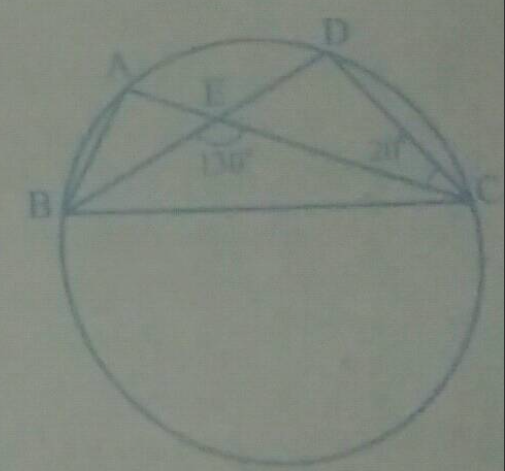
View SolutionIn Fig. 10.39, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC\ =\ 130o and ∠ECD\ =\ 20⊙ Find ∠BAC.
View SolutionTake four distinct points, A,B,C and D no three of which are collinear.
Find the number of lines that can be drawn passing through these points
View SolutionMark four points A, B, C and D in your notebook such that no three of them are collinear. Draw all the lines which join them in pairs as shown in Fig.
How many such lines can be drawn? Write the names of these lines. Name the lines which are concurrent at A .
View SolutionA, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC =130∘ and ∠ECD=20∘ Find ∠BAC∘
View SolutionIn fig.9, D is a point on side BC of a ABC. AD is joined. Name all the triangle that you can observe in the figure. How many are they?
View SolutionIn fig.9, D is a point on side BC of a ABC. AD is joined. Name all the triangle that you can observe in the figure. How many are they?
View SolutionA,B,C,D are any four points, prove that →AB.→CD+→BC.→AD+→CA.→BD=4(Area of△ABC).
View Solution
