In Figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find (i) the length of the boundary (ii) the area of the shaded region.
To solve the problem step by step, we will first find the length of the boundary and then calculate the area of the shaded region.
Step 1: Identify the Radii
- The diameter of the largest semicircle is 14 cm, so its radius R is:
R=142=7 cm
- The diameter of the smallest semicircle is 3.5 cm, so its radius r is:
r=3.52=1.75 cm
Step 2: Calculate the Length of the Boundary
The length of the boundary consists of:
- The length of the largest semicircle
- The lengths of the two middle semicircles (which are equal)
- The lengths of the two smallest semicircles (which are also equal)
The formula for the length of a semicircle is given by:
Length of semicircle=12×π×diameter
1. Length of the largest semicircle:
L1=12×π×14=7π cm
2. Length of the middle semicircles (2 semicircles of diameter 3.5 cm):
L2=2×(12×π×3.5)=2×1.75π=3.5π cm
3. Length of the smallest semicircles (2 semicircles of diameter 1.75 cm):
L3=2×(12×π×1.75)=2×0.875π=1.75π cm
Now, add all these lengths together:
Total Length=L1+L2+L3=7π+3.5π+1.75π=12.25π cm
Using π≈3.14:
Total Length≈12.25×3.14≈38.5 cm
Step 3: Calculate the Area of the Shaded Region
The area of the shaded region is given by:
Area=Area of largest semicircle+Area of middle semicircle−2×Area of smallest semicircle
1. Area of the largest semicircle:
A1=12×π×R2=12×π×72=49π2 cm2
2. Area of the middle semicircle:
A2=12×π×3.52=12×π×12.25=12.25π2 cm2
3. Area of the smallest semicircle:
A3=12×π×(1.75)2=12×π×3.0625=3.0625π2 cm2
Now, substituting these values into the area formula:
Area=A1+A2−2×A3=49π2+12.25π2−2×3.0625π2
=49π+12.25π−6.125π2=55.125π2 cm2
Using π≈3.14:
Area≈55.125×3.142≈86.5 cm2
Final Answers:
(i) The length of the boundary is approximately 38.5 cm.
(ii) The area of the shaded region is approximately 86.5 cm².
- The diameter of the largest semicircle is 14 cm, so its radius R is:
R=142=7 cm
- The diameter of the smallest semicircle is 3.5 cm, so its radius r is:
r=3.52=1.75 cm
The length of the boundary consists of:
- The length of the largest semicircle
- The lengths of the two middle semicircles (which are equal)
- The lengths of the two smallest semicircles (which are also equal)
Length of semicircle=12×π×diameter
L1=12×π×14=7π cm
L2=2×(12×π×3.5)=2×1.75π=3.5π cm
L3=2×(12×π×1.75)=2×0.875π=1.75π cm
Total Length=L1+L2+L3=7π+3.5π+1.75π=12.25π cm
Total Length≈12.25×3.14≈38.5 cm
The area of the shaded region is given by:
Area=Area of largest semicircle+Area of middle semicircle−2×Area of smallest semicircle
A1=12×π×R2=12×π×72=49π2 cm2
A2=12×π×3.52=12×π×12.25=12.25π2 cm2
A3=12×π×(1.75)2=12×π×3.0625=3.0625π2 cm2
Area=A1+A2−2×A3=49π2+12.25π2−2×3.0625π2
=49π+12.25π−6.125π2=55.125π2 cm2
Area≈55.125×3.142≈86.5 cm2
(i) The length of the boundary is approximately 38.5 cm.
(ii) The area of the shaded region is approximately 86.5 cm².
Topper's Solved these Questions
Similar Questions
The boundary of the shaded region in the given figure consists of three semicircular areas, the smaller ones being equal. If the diameter of the larger one is 14 cm, calculate
(i) the length of the boundary
(ii) the area of the shaded regionView SolutionIn figure, find the area of the shaded region [Useπ=3.14]
 View Solution
View SolutionIn figure, find the area of the shaded region [Useπ=3.14]
View SolutionIn the given figure, find the area of the shaded region [Useπ=3.14]
View SolutionIn the given figure, PQ = 24 cm PR = 7 cm and O is the centre of the circle. Find the area of the shaded region. (Use π=3.14)
 View Solution
View SolutionIn the adjoining figure radius is 14 cm ,calculate:
(i) the length of minor arc ACB
(ii) area of shaded sectorView SolutionIn figure arcs have been drawn with radii 14 cm each and with centres P, Q and R. Find the area of the shaded region .
 View Solution
View SolutionIn the figure, AB is a diameter of the circle with centre O and OA = 7cm. Find the area of the shaded region
View SolutionThree semicircles each of diameter 3 cm, a circle of diameter 4·5 cm and a semicircle of radius 4·5 cm are drawn in the given figure. Find the area of the shaded region.
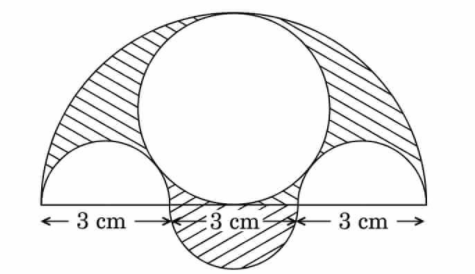 View Solution
View SolutionThree semicircles each of diameter 3 cm, a circle of diameter 4.5 cm and a semicircle of radius 4.5 cm are drawn in the adjoining figure. Find the area of the shaded region.
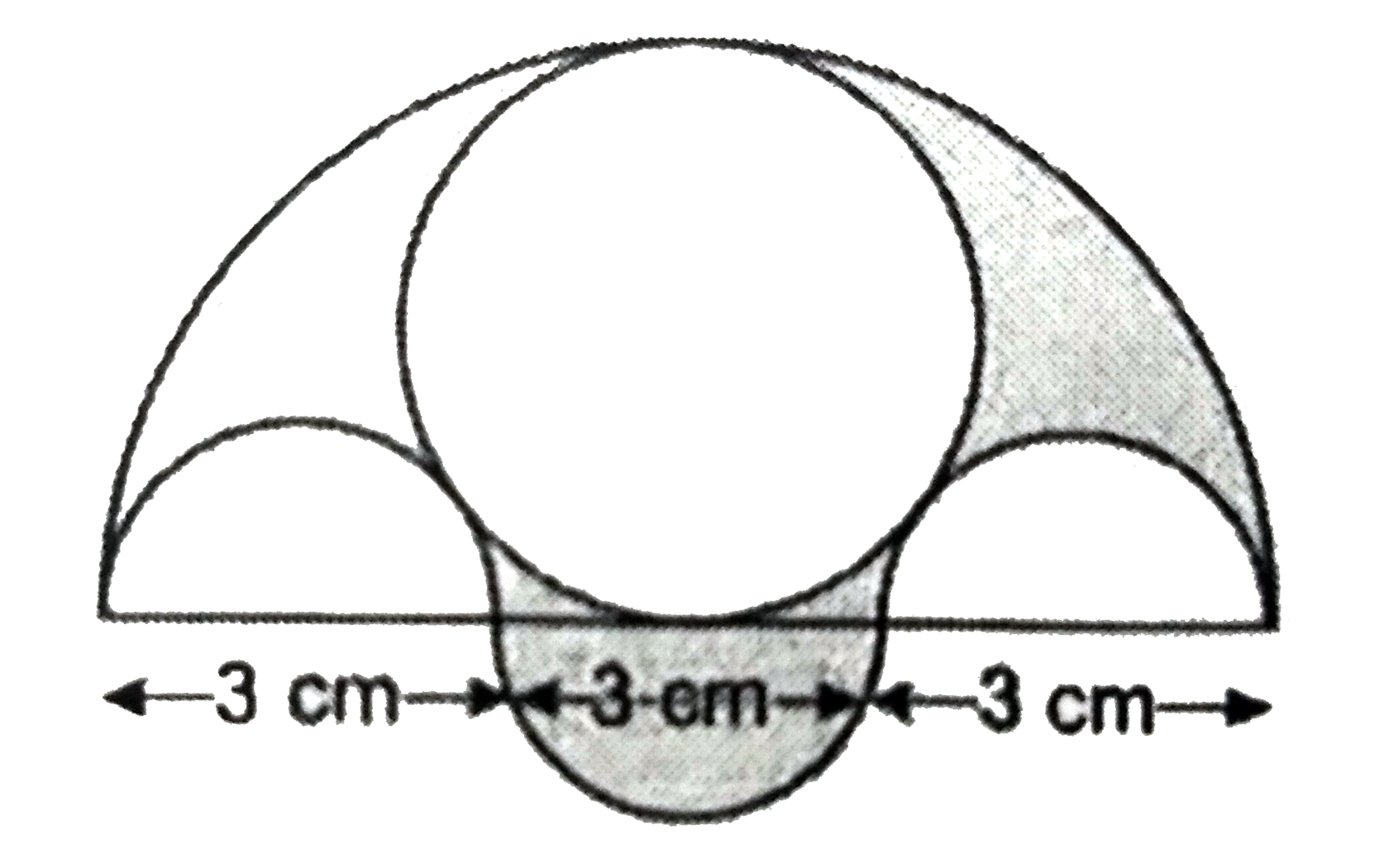 View Solution
View Solution

