Question

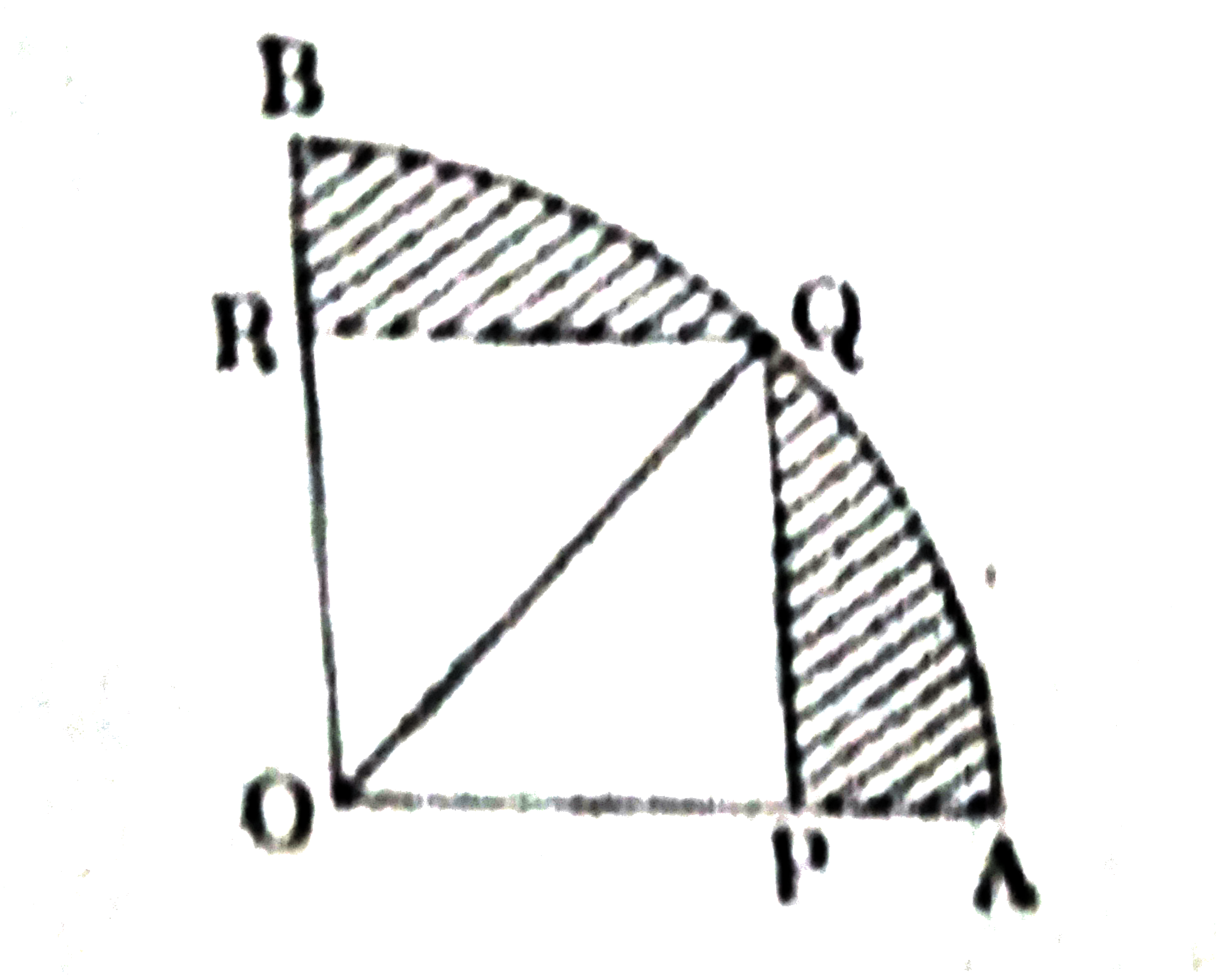
In Fig. 15.99, a square $OABC$
is
inscribed in a quadrant $OPBQ$
of a
circle. If $OA=21cm$
, find the area of the shaded region.
(FIGURE)

Explanation

To find the area of the shaded region in the given figure, we will follow these steps:

Step 1: Understand the Problem
We have a square \( OABC \) inscribed in a quadrant \( OPBQ \) of a circle. The side length of the square \( OA \) is given as \( 21 \, cm \).

Step 2: Calculate the Area of the Square
The area of a square is calculated using the formula:
\( \text{Area of square} = \text{side} \times \text{side} \)
Given that \( OA = 21 \, cm \):
\( \text{Area of square} = 21 \times 21 = 441 \, cm^2 \)

Step 3: Determine the Radius of the Circle
The diagonal of the square \( OB \) is equal to the radius of the circle. The diagonal of a square can be calculated using the formula:
\( \text{Diagonal} = \text{side} \times \sqrt{2} \)
So, the diagonal \( OB \) is:
\( OB = 21 \times \sqrt{2} \, cm \)

Step 4: Calculate the Area of the Quadrant
The area of a quadrant of a circle is given by the formula:
\( \text{Area of quadrant} = \frac{1}{4} \pi r^2 \)
Substituting \( r = 21\sqrt{2} \):
\( \text{Area of quadrant} = \frac{1}{4} \times \frac{22}{7} \times (21\sqrt{2})^2 \)
Calculating \( (21\sqrt{2})^2 \):
\( (21\sqrt{2})^2 = 441 \times 2 = 882 \)
Now substituting back:
\( \text{Area of quadrant} = \frac{1}{4} \times \frac{22}{7} \times 882 \)
Calculating this:
\( \text{Area of quadrant} = \frac{22 \times 882}{28} = \frac{19384}{28} = 693 \, cm^2 \)

Step 5: Calculate the Area of the Shaded Region
The area of the shaded region is the area of the quadrant minus the area of the square:
\( \text{Area of shaded region} = \text{Area of quadrant} - \text{Area of square} \)
Substituting the values we calculated:
\( \text{Area of shaded region} = 693 \, cm^2 - 441 \, cm^2 = 252 \, cm^2 \)

Final Answer
The area of the shaded region is \( 252 \, cm^2 \).

---