In statistical survey of 1003 families has neither a radio nor a TV, 794 families in that group having both a radio and a TV is
36
41
32
None of these
The correct Answer is:B
Let R be the set of famulies having a redio and T be the set of families having a TV.
Then
n(R∪T)
Number of families having both a radio and a TV .
=1003- 63 = 940
n( R) = 794 and n (T) = 187
Let x be the Number of families having both a radio and a TV
Then number of families who have only Radio = 794 - x
Number of families who have only Radio = 794 - x
Number of families who have only TV= 187 - x
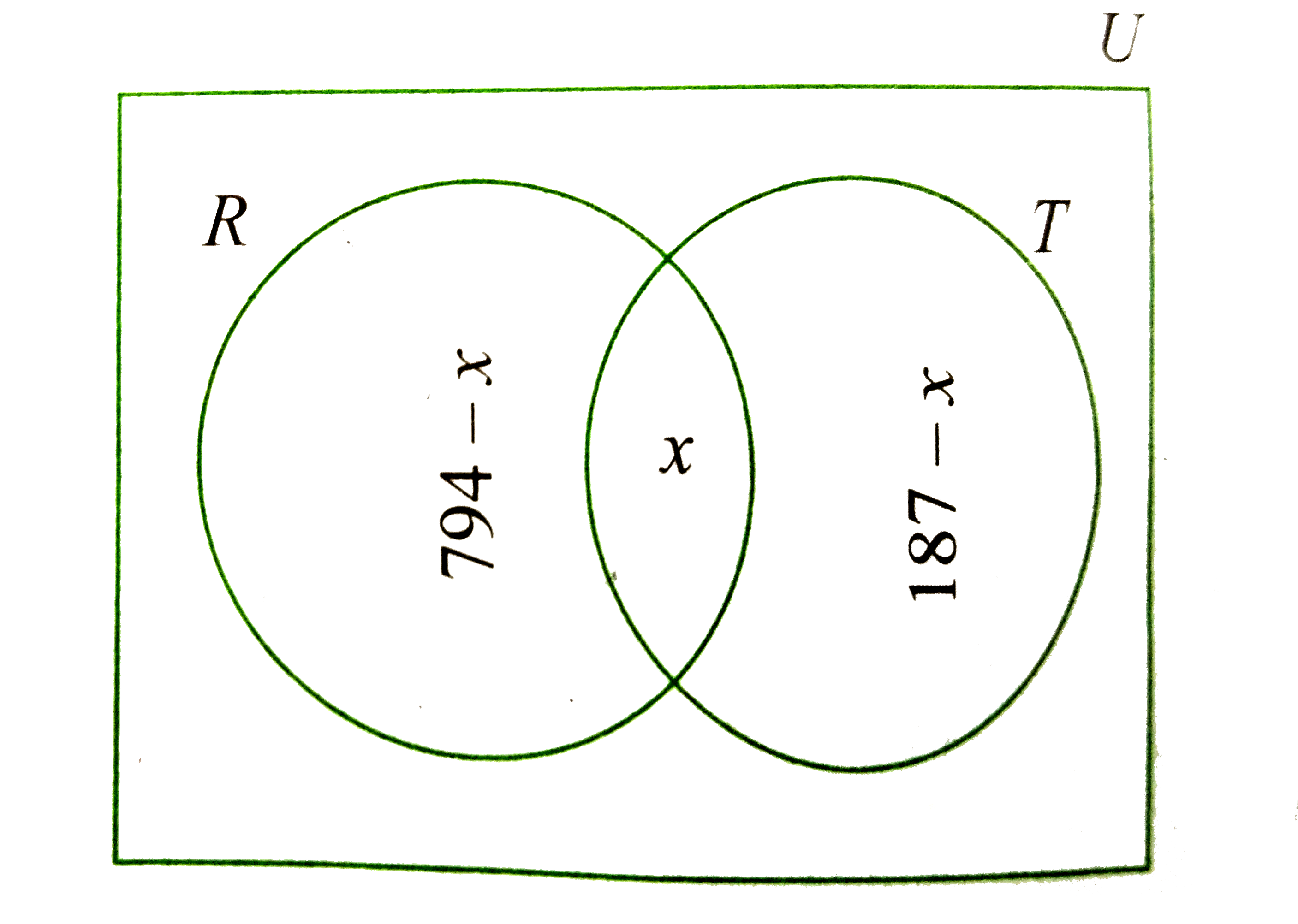
From Venn diagram
794 - x +x+187 - x = 940
⇒ 981- x = 940
∴ x= 981 -940 = 41
Hence the required number of families having boht a redio and a TV is 41 .
Topper's Solved these Questions
- SET THEORY AND REAL NUMBER SYSTEM BOOK - CENGAGECHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Archieves
1 Video
- SET THEORY AND REAL NUMBER SYSTEM BOOK - CENGAGECHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Exercise 1.5
12 Videos
- SEQUENCE AND SERIESBOOK - CENGAGECHAPTER - SEQUENCE AND SERIESEXERCISE - Question Bank
36 Videos
- SETS AND RELATIONSBOOK - CENGAGECHAPTER - SETS AND RELATIONSEXERCISE - Question Bank
15 Videos
Similar Questions
In a statistical investigation of 1003 families of Calcutta, it was found that 63 families has neither a radio nor a t.v,794 families has a radio and 187 has T.V. The number of families in that group having both a radio and a T.V is
View SolutionIn a statistical investigation of 1003 families of Calcutta, it was found that 63 families has neither a radio nor a T.V, 794 families has a radio and 187 has T.V. The number of families in the group having both a radio and a T.V is
View Solution
Knowledge Check
In a statistical investigation of 1003 families of Calcutta, it was found that 63 families has neither a radio nor a T.V, 794 families has a radio and 187 has T.V. The number of families in that group having both a radio and a T.V is
A36B41C32DNone of theseIn a certain town 25% families own a phone and 15% own a car 65% own neither a phone nor a car. 2000 families own both a car and a phone. Consider the following statements in this regard
(1) 10% families own both a car and a phone
(2) 35% families own neither a car or a phone
(3) 40,000 families live in the town
Which one of these statements are correct?A1 and 2B1 and 3C2 and 3D1,2 & 3In a certain town, 25% of the families own a phone and 15% own a car, 65% families own neither a phone nor a car and 2,000 families own both a car and a phone. Consider the following three statements :
(A) 5% families own both a car and a phone
(B) 35% families own either a car or a phone
(C ) 40,000 families live in the town
Then,AOnly (A) and (C ) are correctBOnly (B) and (C ) are correctCAll (A), (B) and (C ) are correctDOnly (A) and (B) are correct
In a statistical investigation of 1,003 families of Calcutta, it was found that 63 families had neither a radio nor a T.V, 794 families had a radio and 187 had a T.V. The number of families in that group having both a radio and a T.V is (a) 36 (b)41 (c) 32 (d) None of these
View SolutionIn a certain town 25% families own a phone and 15% own a car, 65% families own neither a phone nor a car, 2000 families own both a car and a phone . How many families live in the town ?
View SolutionIn a certain town 25% families own a phone and 15% own a car, 65% families own neither a phone nor a car, 2000 families own both a car and a phone . How many families live in the town ?
View SolutionIn a certain town, 25% families own a phone and 15% own a car, 65% families own neither a phone nor a car. 2000 families own both a car and a phone, then
View SolutionIn a survey of families, chosen randomly, it was found that 375 families had two girls, 514 families had only one girl, and 111 had no girl. If a family is selected at random, what is the probability that it has two girls?
View Solution
