In the adjoining figure, OX=12XY,PX=12XZand OX =PX. Show that XY= XZ
To solve the problem, we need to show that XY=XZ given the conditions OX=12XY, PX=12XZ, and OX=PX.
1. Write down the given equations:
- From the problem, we have:
OX=12XY(1)
PX=12XZ(2)
OX=PX(3)
2. Substitute OX from equation (3) into equation (1):
- Since OX=PX, we can replace OX in equation (1):
PX=12XY(4)
3. Now, we have two expressions for PX:
- From equation (2), we have:
PX=12XZ(5)
4. Set equations (4) and (5) equal to each other:
- Since both equations equal PX, we can set them equal:
12XY=12XZ
5. Eliminate the common factor of 12:
- Multiply both sides by 2 to eliminate the fraction:
XY=XZ
6. Conclusion:
- We have shown that XY=XZ.
Final Result:
Thus, we conclude that XY=XZ.
---
- From the problem, we have:
OX=12XY(1)
PX=12XZ(2)
OX=PX(3)
- Since OX=PX, we can replace OX in equation (1):
PX=12XY(4)
- From equation (2), we have:
PX=12XZ(5)
- Since both equations equal PX, we can set them equal:
12XY=12XZ
- Multiply both sides by 2 to eliminate the fraction:
XY=XZ
- We have shown that XY=XZ.
Thus, we conclude that XY=XZ.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO EUCLID GEOMETRYEXERCISE - Exercise 5.4 Long Answer Type Questions5videos
INTRODUCTION TO EUCLID GEOMETRY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO EUCLID GEOMETRYEXERCISE - Exercise 5.2 Very Short Answer Type Questions9videos
INTRODUCTION TO EUCLID GEOMETRY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - HERON'S FORMULAEXERCISE - Long Answer Type Questions8videos
HERON'S FORMULA
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR EQUATION IN TWO VARIABLESEXERCISE - Exercise 4.81videos
LINEAR EQUATION IN TWO VARIABLES
Similar Questions
In the adjoining figure , if BX=12AB,BY=12BCandAB=BC, then show that BX =BY.
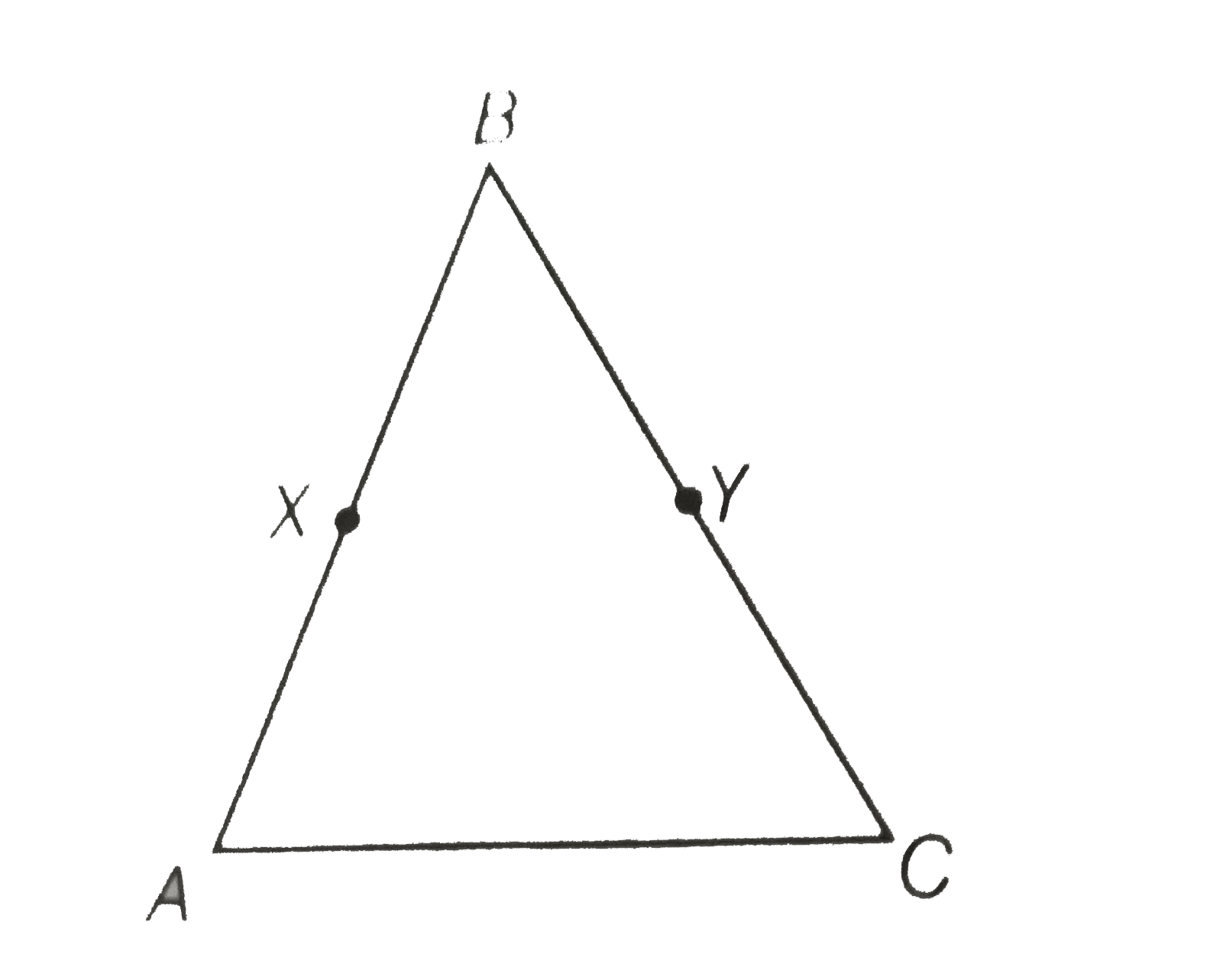 View Solution
View SolutionIn the adjoining figure, we have ∠1=∠2and=∠3. Show that ∠1=∠3.
 View Solution
View SolutionIn the adjoining figure, we have ∠1=∠3and∠2=∠4. Show that ∠A=∠C.
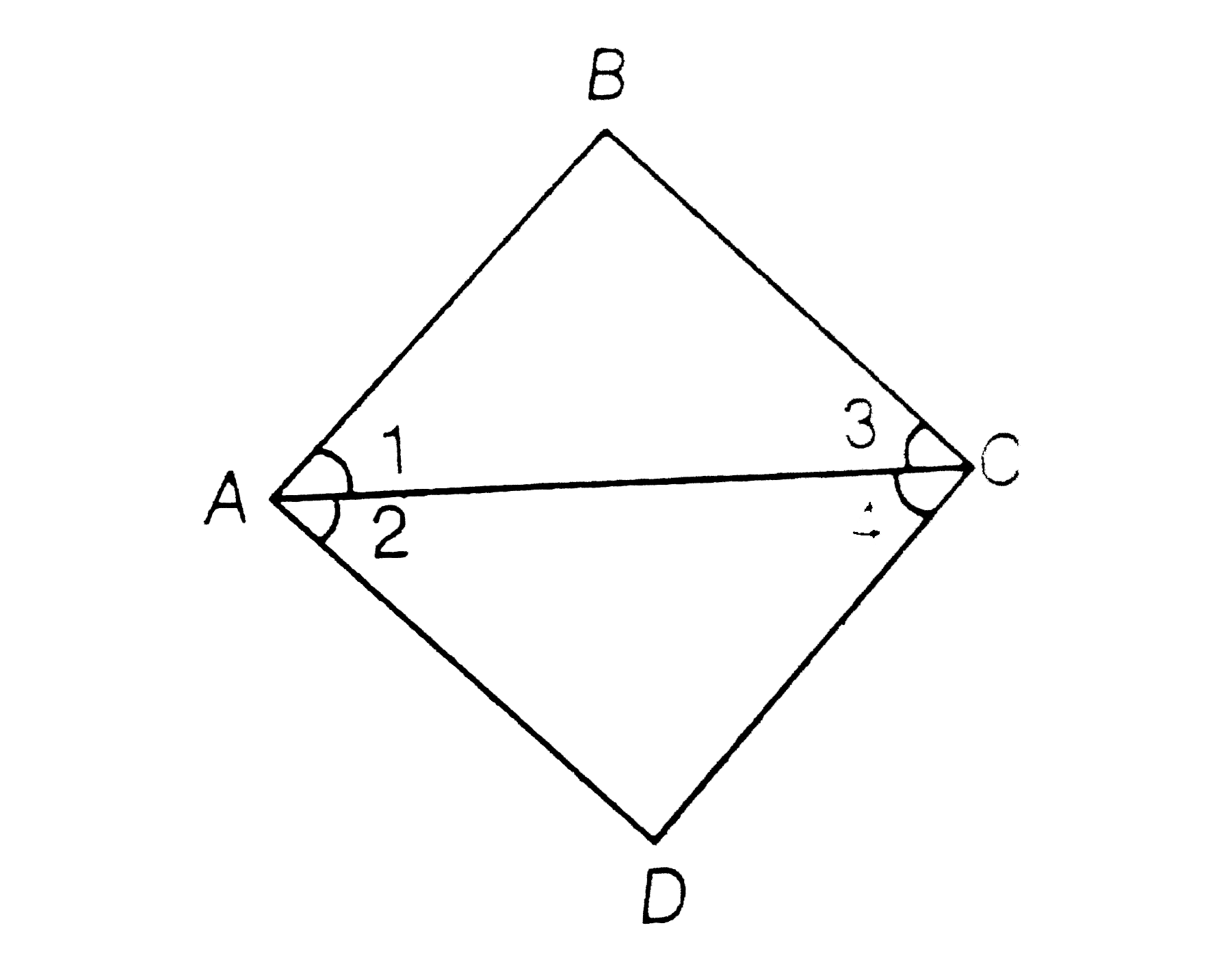 View Solution
View SolutionIn the adjoining figure, OX and RX are the bisectors of the angle Q and R respectively of the triangle PQR. If XS⊥QR and XT⊥PQ.
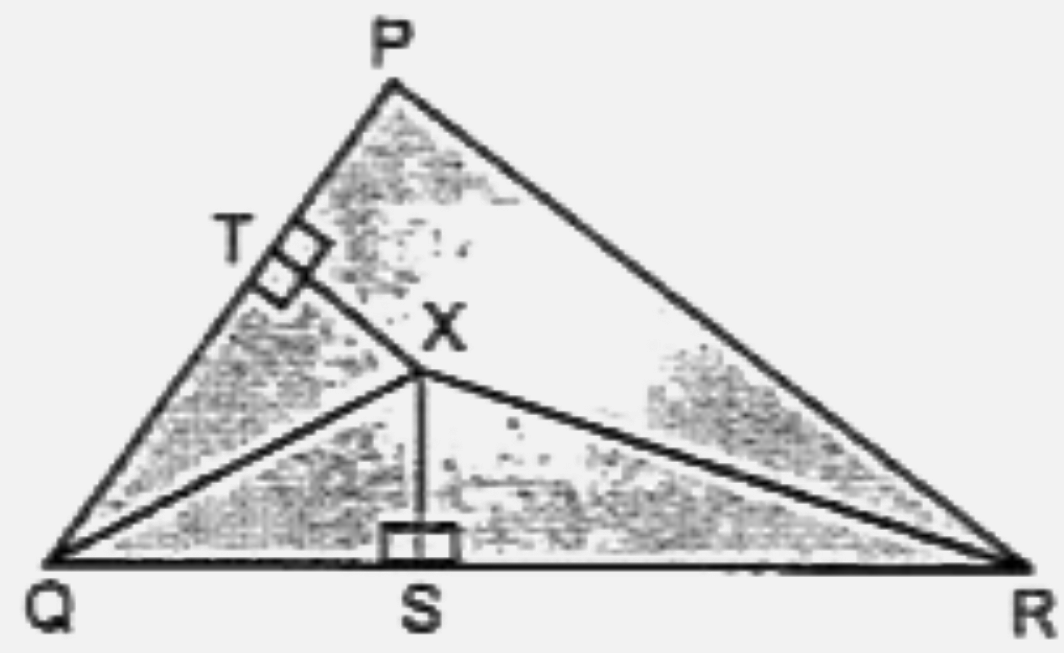
Prove that :
PX bisects angle P.View SolutionIn the adjoining figure, OX and RX are the bisectors of the angle Q and R respectively of the triangle PQR. If XS⊥QR and XT⊥PQ.
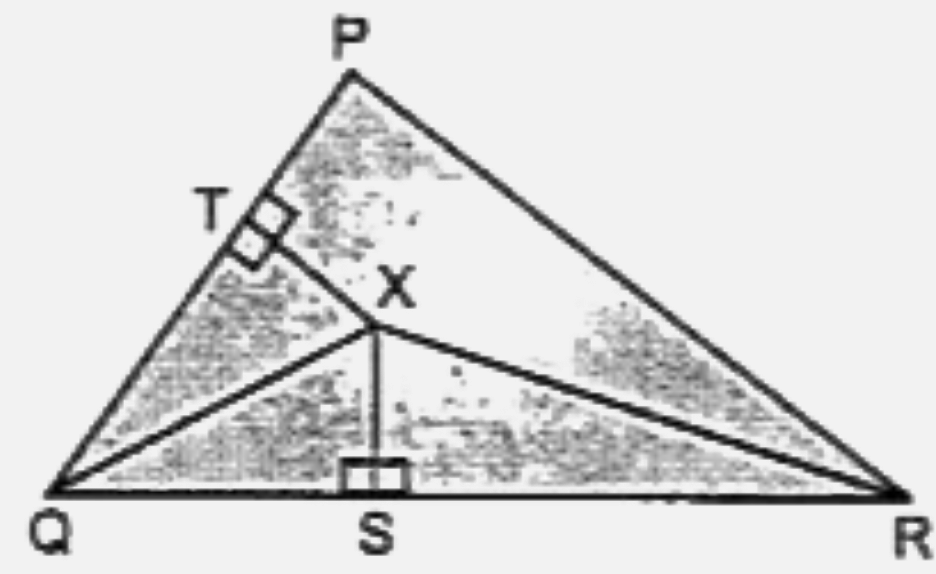
Prove that :
ΔXTQ≅ΔXSQView SolutionIn the adjoining figure, ∠AYZ=∠BYX=90∘,AY=YZ and XY=BY. Prove that AB=ZX.
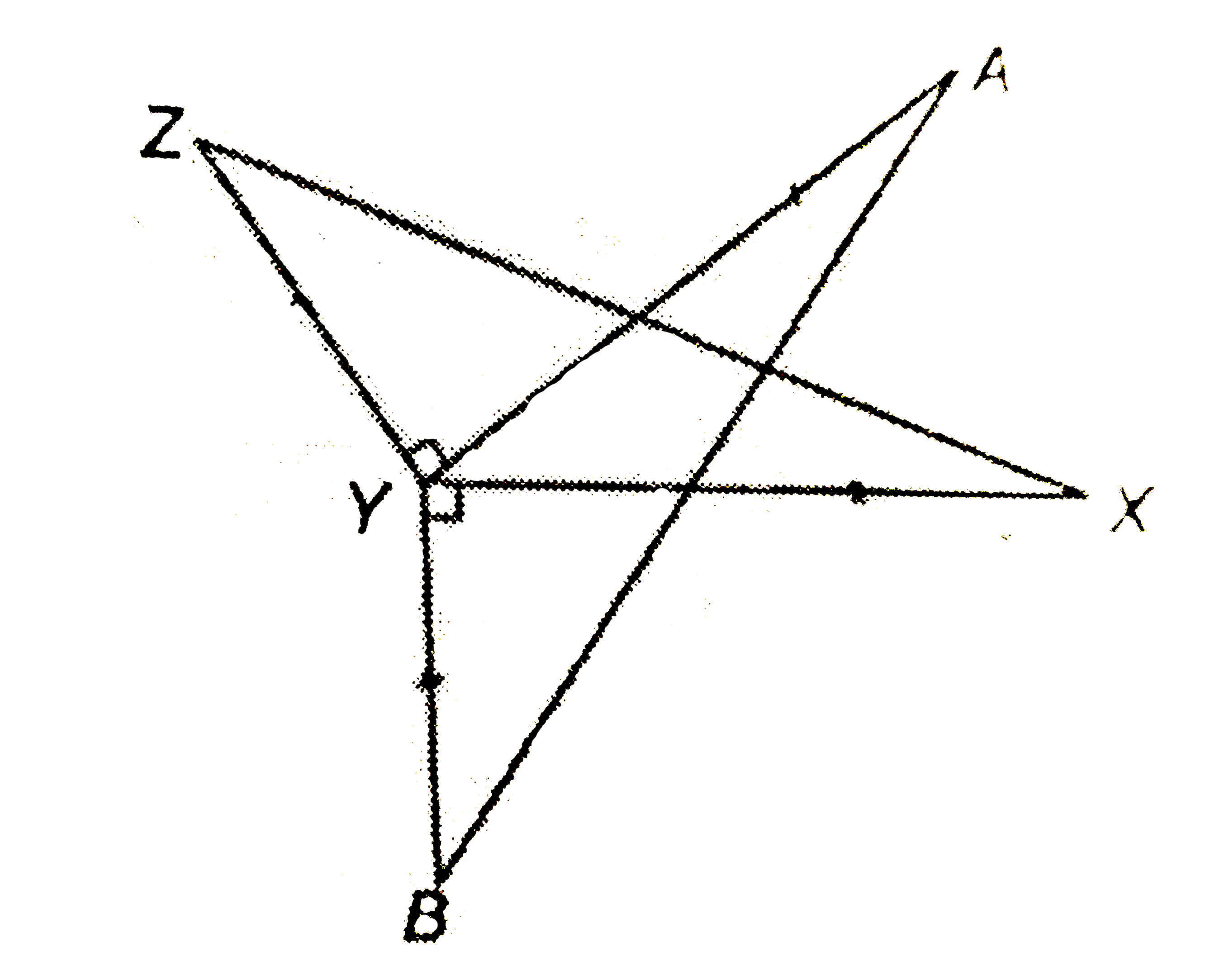 View Solution
View SolutionGraph between log k and 1T (k is rate constant in s−1 and T is the temperature in K) is a straight line. As shown in figure if OX = 5 and slope of the line =−12.303then Ea is :
 View Solution
View SolutionShow that the area bounded by the lines x = 0, y = 1, y = 2 and the hyperbola xy = 1 is log 2.
View SolutionIf xy=6,yz=10,xz=15,andx>0, then xyz=
View SolutionIf xz-plane divide the join of point (2,3,4)and(1,−1,5) in the ratio λ:1, then the integer λ should be equal to
View Solution
