In the first proof reading of a book containing 300 pages the following distribution of misprints was obtained: No. of misprints per page (x) : 0 1 2 3 4 5 No. of pages (f) : 154 95 36 9 5 1 Find the average number of misprints per page.
To find the average number of misprints per page in the given distribution, we will follow these steps:
Step 1: Organize the data
We will create a table with the number of misprints per page (x) and their corresponding frequencies (f).
| No. of Misprints (x) | Frequency (f) |
|-----------------------|---------------|
| 0 | 154 |
| 1 | 95 |
| 2 | 36 |
| 3 | 9 |
| 4 | 5 |
| 5 | 1 |
Step 2: Calculate the assumed mean (A)
We will assume the mean (A) to be 2, as it is a central value in the distribution.
Step 3: Calculate deviations (ui)
The deviation (ui) for each class can be calculated using the formula:
ui=xi−A
Where A is the assumed mean.
| No. of Misprints (x) | Frequency (f) | Deviation (u) = x - A |
|-----------------------|---------------|------------------------|
| 0 | 154 | 0 - 2 = -2 |
| 1 | 95 | 1 - 2 = -1 |
| 2 | 36 | 2 - 2 = 0 |
| 3 | 9 | 3 - 2 = 1 |
| 4 | 5 | 4 - 2 = 2 |
| 5 | 1 | 5 - 2 = 3 |
Step 4: Calculate fi⋅ui
Now we will calculate the product of frequency and deviation for each class.
| No. of Misprints (x) | Frequency (f) | Deviation (u) | fi⋅ui |
|-----------------------|---------------|----------------|----------------------|
| 0 | 154 | -2 | 154 * -2 = -308 |
| 1 | 95 | -1 | 95 * -1 = -95 |
| 2 | 36 | 0 | 36 * 0 = 0 |
| 3 | 9 | 1 | 9 * 1 = 9 |
| 4 | 5 | 2 | 5 * 2 = 10 |
| 5 | 1 | 3 | 1 * 3 = 3 |
Step 5: Sum up the frequencies and fi⋅ui
Now we will calculate the total of frequencies and the total of fi⋅ui.
- Total frequency ∑fi=154+95+36+9+5+1=300
- Total ∑(fi⋅ui)=−308−95+0+9+10+3=−381
Step 6: Calculate the average number of misprints
We will use the formula for the average number of misprints per page:
Average=A+∑(fi⋅ui)∑fi
Substituting the values:
Average=2+−381300
Calculating:
Average=2−1.27=0.73
Final Answer
The average number of misprints per page is 0.73.
---
We will create a table with the number of misprints per page (x) and their corresponding frequencies (f).
|-----------------------|---------------|
| 0 | 154 |
| 1 | 95 |
| 2 | 36 |
| 3 | 9 |
| 4 | 5 |
| 5 | 1 |
We will assume the mean (A) to be 2, as it is a central value in the distribution.
The deviation (ui) for each class can be calculated using the formula:
ui=xi−A
Where A is the assumed mean.
|-----------------------|---------------|------------------------|
| 0 | 154 | 0 - 2 = -2 |
| 1 | 95 | 1 - 2 = -1 |
| 2 | 36 | 2 - 2 = 0 |
| 3 | 9 | 3 - 2 = 1 |
| 4 | 5 | 4 - 2 = 2 |
| 5 | 1 | 5 - 2 = 3 |
Now we will calculate the product of frequency and deviation for each class.
|-----------------------|---------------|----------------|----------------------|
| 0 | 154 | -2 | 154 * -2 = -308 |
| 1 | 95 | -1 | 95 * -1 = -95 |
| 2 | 36 | 0 | 36 * 0 = 0 |
| 3 | 9 | 1 | 9 * 1 = 9 |
| 4 | 5 | 2 | 5 * 2 = 10 |
| 5 | 1 | 3 | 1 * 3 = 3 |
Now we will calculate the total of frequencies and the total of fi⋅ui.
- Total ∑(fi⋅ui)=−308−95+0+9+10+3=−381
We will use the formula for the average number of misprints per page:
Average=A+∑(fi⋅ui)∑fi
Substituting the values:
Average=2+−381300
Average=2−1.27=0.73
The average number of misprints per page is 0.73.
Topper's Solved these Questions
Similar Questions
The following table gives the number of children of 150 families in a village No. of children (x) : 0 1 2 3 4 5 No. of families (f) : 10 21 55 42 15 7 Find the average number of children per family.
View SolutionThe following distribution gives the number of accidents met by 160 workers in a factory during a month. No. of accidents (x) : 0 1 2 3 4 No. of workers (f) : 70 52 34 3 1 Find the average number of accidents per worker.
View SolutionIla read 25 pages of a book containing 100 pages. Lalita read 25 of the same book. Who read less?
View SolutionIsha read 25 pages of a book containing 100 pages. Nagma read 12 of the same book. Who read less?
View SolutionFind the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 1.46. No of accident (x) 0 1 2 3 4 5 Total Frequency (f): 46 ? ? 25 10 5 200
View SolutionFind the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 1.46. No. of accident (x) 0 1 2 3 4 5 Total Frequency (f): 46 ? ? 25 10 5 200
View SolutionIf the mean of the following distribution is 6, find the value of p. x: 2 4 6 10 p+5 f: 3 2 3 1 2
View SolutionThe number of telephone calls received at an exchange per interval for 250 successive one-minute intervals are given in the following frequency table: No. of calls (x) : 9 1 2 3 4 5 6 No. of intervals (f) : 15 24 29 46 54 43 39 Compute the mean number of calls per interval.
View SolutionIf the mean of the following distribution is 6, find the value of p
x:24610p+5f:32312View SolutionThe following data is related to the number of mistakes in each page of a book containing 180 pages :
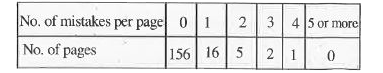
Fit a Poisson distribution to the data. Obtain the theoretical frequencies.View Solution

