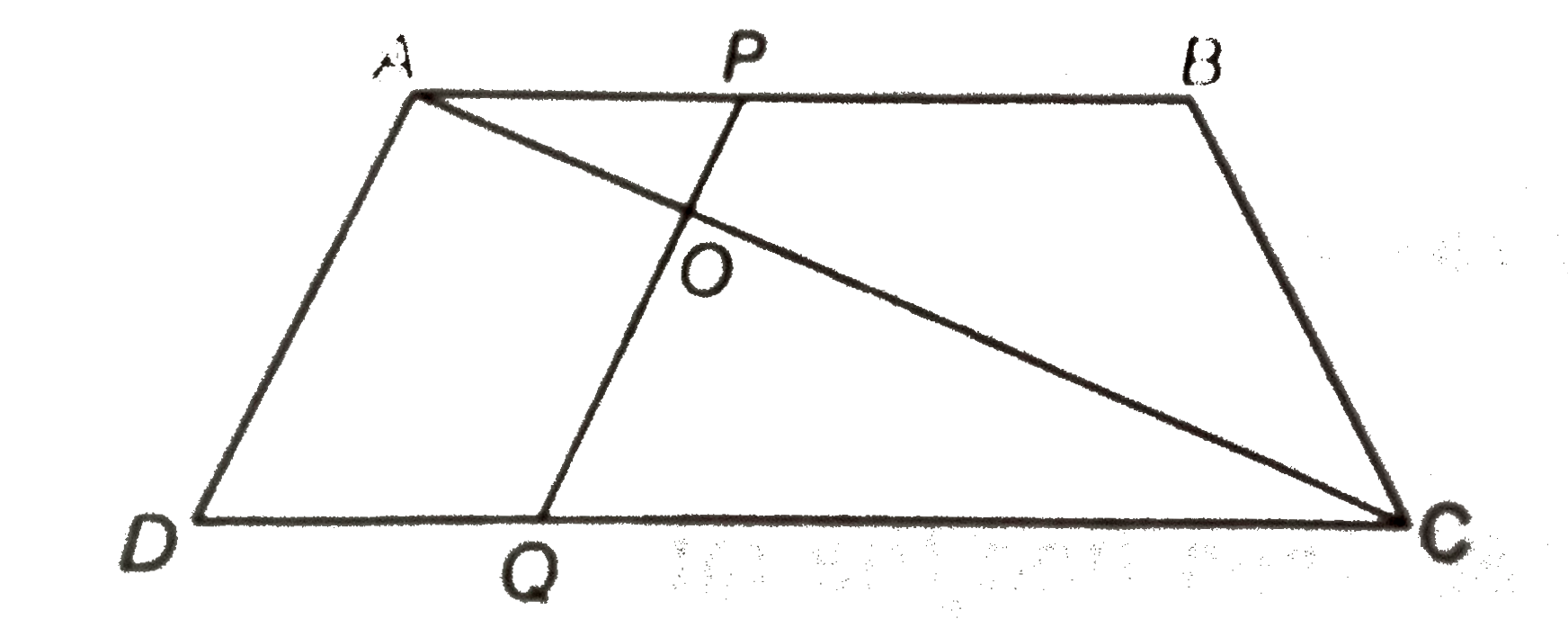
Kamala have drawn a trapezium PQRS of which PQ∣∣SR. If the diagonals PR and QS intersect each other at O, then prove that OP:OR=OQ:OS, If SR=2PQ, then prove that O is a point of trisection of both the diagonals.
Step by step video & image solution for Kamala have drawn a trapezium PQRS of which PQ||SR. If the diagonals PR and QS intersect each other at O, then prove that OP:OR=OQ:OS, If SR=2PQ, then prove that O is a point of trisection of both the diagonals. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMILARITYBOOK - UNIQUE PUBLICATIONCHAPTER - SIMILARITYEXERCISE - Unique practise Session (MCQs)
5 Videos
- SIMILARITYBOOK - UNIQUE PUBLICATIONCHAPTER - SIMILARITYEXERCISE - Unique practise Session (1 Marks Question)
3 Videos
- QUADRATIC EQUATIONS BOOK - UNIQUE PUBLICATIONCHAPTER - QUADRATIC EQUATIONS EXERCISE - ASSIGNMENT
6 Videos
- STATISTICS BOOK - UNIQUE PUBLICATIONCHAPTER - STATISTICS EXERCISE - PROBLEM SET
6 Videos
Similar Questions
Knowledge Check
ABCD is a trapezium in which AB||CD and AB=2CD. If its diagonals intersect each other at O, then ratio of areas of trianlges AOB and COD is :
A1:4B1:2C4:1D2:1ABCD is a trapezium in which AB∣∣CD and AB=2CD. It its diagonals intersect each other at O then ratio of the area of triangle AOB and COD is
A1:2B2:1C1:4D4:1
ABCD is a trapezium in which AB||DC and its diagonals intersect each other as the point O. Show that AOBO=CODO.
View SolutionABCD is a trapezium in which AB||DC and its diagonals intersect each other at the point O. Show that AOBO=CODO.
View SolutionPQ and RS are two parallel chords of a circle and lines RP and SQ intersect each other at O as shown in Figure. Prove that OP=OQ
View SolutionPQandRS are two parallel chords of a circle and lines RPandSQ intersect each other at O as shown in Figure. Prove that OP=OQ.

View SolutionIn figure , if AB||DC and AC, PQ intersect each other at the point O. Prove that OA.CQ=OC.AP.
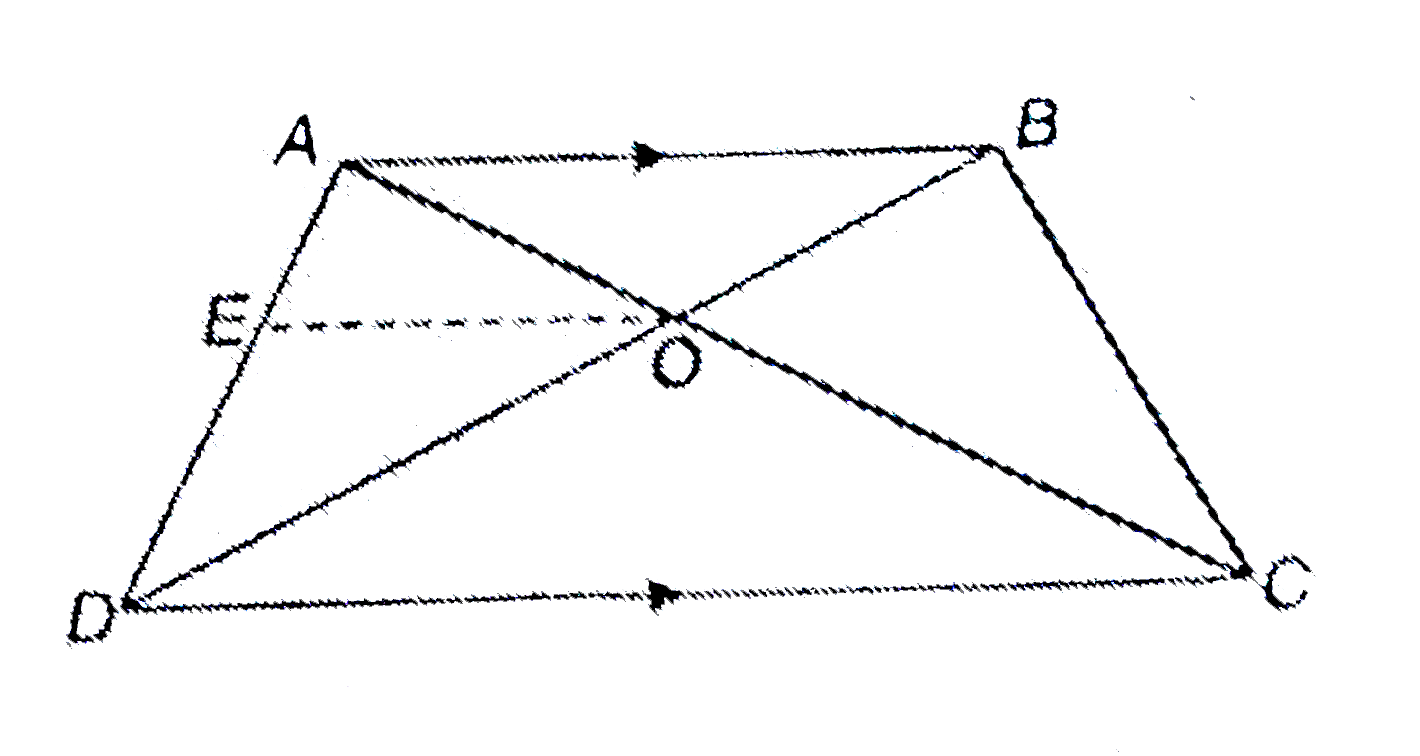
View SolutionThe diagonals of a quadrilateral ABCD intersect each other at the point O such that AOBO=CODO. Show that ABCD is a trapezium.
View Solution