Let f:[0'1]→[−1,1] and g:[−1,1]→[0,2] be two functions such that g is injective and g∘f:[0,1]→[0,2] is surjective. Then
f must be injective but need not be surjective
f must be surjective but need not be injective
f must be bijective
f must be a constant functions.
The correct Answer is:B
let h(x)=9(f(x))
and n(x) is onto (given)
∴ Co-domain of h(x)= Range of h(x)
Range of h(x)=[0,2]
and h(x)= 9(f(x)) it means g is giving [0,2] which is also co-domain of g.
So, g must be onto.
Now, Domain ofg g=[-1,1] which must be range of f.
But,co-domain of f=[-1,1]
So, f must be onto
Topper's Solved these Questions
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - PART-2 MATHMATICS
10 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part 2 Mathematics
15 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part B- Mathematics
10 Videos
- KVPY 2021BOOK - KVPY PREVIOUS YEARCHAPTER - KVPY 2021EXERCISE - PART II MATHEMATICS
4 Videos
Similar Questions
Knowledge Check
Let f : (-1,1) → R be a differentiabale function with f(0) = -1 and f'(0) = 1. Let
g(x) = [f(2f(x)+2)]2 , then g'(0)=A4B−4C0D−2Let f:[0,1]→R be an injective continuous function that satisfies the condition −1<f(0)<f(1)<1
Then the number of functions g:[−1,1]→[0,1] such that (gof)x=x for all x∈[0,1] isA0B1Cmore than 1, but finiteDinfiniteLet f: [0, 1] → [1, 2] defined as f(x) = 1 + x and g : [1, 2] → [0, 1] defined as g(x) = 2 – x then the composite function gof is
Ainjective as well as surjectiveBSurjective but not injectiveCInjective but non surjectiveDNeither injective nor surjective
Let f : (-1,1) → R be a differentiable function with f(0) =-1 and f'(0)=1
Let g(x)=[f(f(2x)+1)]2 . Then g'(0)=
View SolutionLet f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
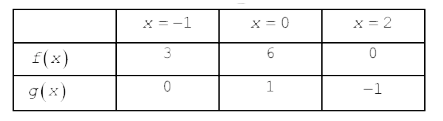
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :
View SolutionLet f and g be real valued functions defined on interval (−1,1) such that g'' (x) is continuous, g(0)≠0,g'(0)=0,g''(0)≠0,andf(x)=g''(0)≠0,andf(x)g(x)sinx.
Statement I limx→0[g(x)cosx−g(0)][cosecx]=f''(0). and
Statement II f'(0) = g(0).
View SolutionLet f:(−1,1)→R be a differentiable function with f(0)=−1 and f'(0)=1. Let g(x)=[f(2f(x)+2)]2.
Then g'(0)=
View SolutionLet f : (-1,1) → R be a differentiabale function with f(0) = -1 and f'(0) = 1. Let
g(x) = [f(2f(x)+2)]2 , then g'(0)=
View Solution
