Question

Let RS be the diameter of the circle $x^2+y^2=1,$ where S is the point $(1,0)$ Let P be a variable apoint (other than $R\text{and}S$) on the circle and tangents to the circle at $S\text{and}P$ meet at the point Q.The normal to the circle at P intersects a line drawn through Q parallel to RS at point E. then the locus of E passes through the point(s)- (A) $(\frac{1}{3},\frac{1}{\sqrt{3}})$ (B) $(\frac{1}{4},\frac{1}{2})$ (C) $(\frac{1}{3},-\frac{1}{\sqrt{3}})$ (D) $(\frac{1}{4},-\frac{1}{2})$

Options

A. `((1)/(3),(1)/(sqrt(3)))`
B. `((1)/(4),(1)/(2))`
C. `((1)/(3),- (1)/(sqrt(3)))`
D. `((1)/(4), -(1)/(2))`

Answer

1,3

Explanation

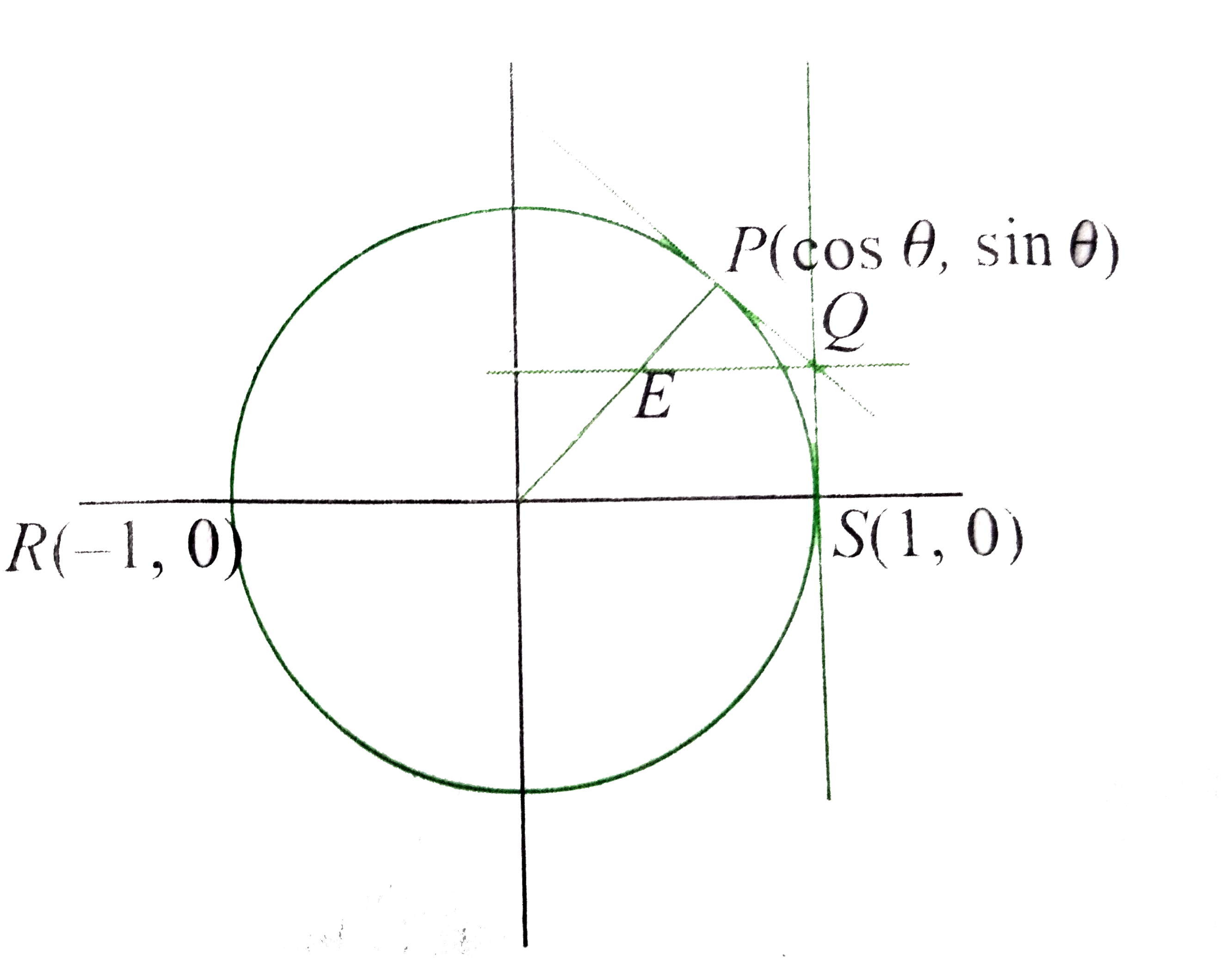
Let point P be `( cos theta , sin theta )`.
Tangent at P is `x cos theta + y sin theta =1`
`:. Q -= (1,(1cos theta )/(sin theta ))`
Normal at `P, y= tan theta x` (i)
Equation of QE` , y = (1-cos theta )/( sin theta )` (ii)
Point E is point of intersection of lines (i) and (ii) .
Eliminating `theta` from (i) and (ii), we get locus of point E.
From (i) , `tan theta = (y)/(x )`
From eq. (ii), we get
y=`(1-(x)/(sqrt(x^(2)+y^(2))))/((y)/(sqrt(x^(2)+y^(2))))=(sqrt(x^(2)+y^(2))-x)/(y)` ltbr. or `y^(2)+x= sqrt (x^(2)+y^(2))`
Clearly option (1) and (3) satisfy the above equation.