Lines $L_{1} \equiv a x + b y + c = 0$ and $L_{2} \equiv l x + m y + n = 0$ intersect at the point $P$ and make an angle $θ$ with each other. Find the equation of a line different from $L_{2}$ which passes through $P$ and makes the same angle $θ$ with $L_{1} \dot{}$

Video Solution

Text Solution

Verified by Experts

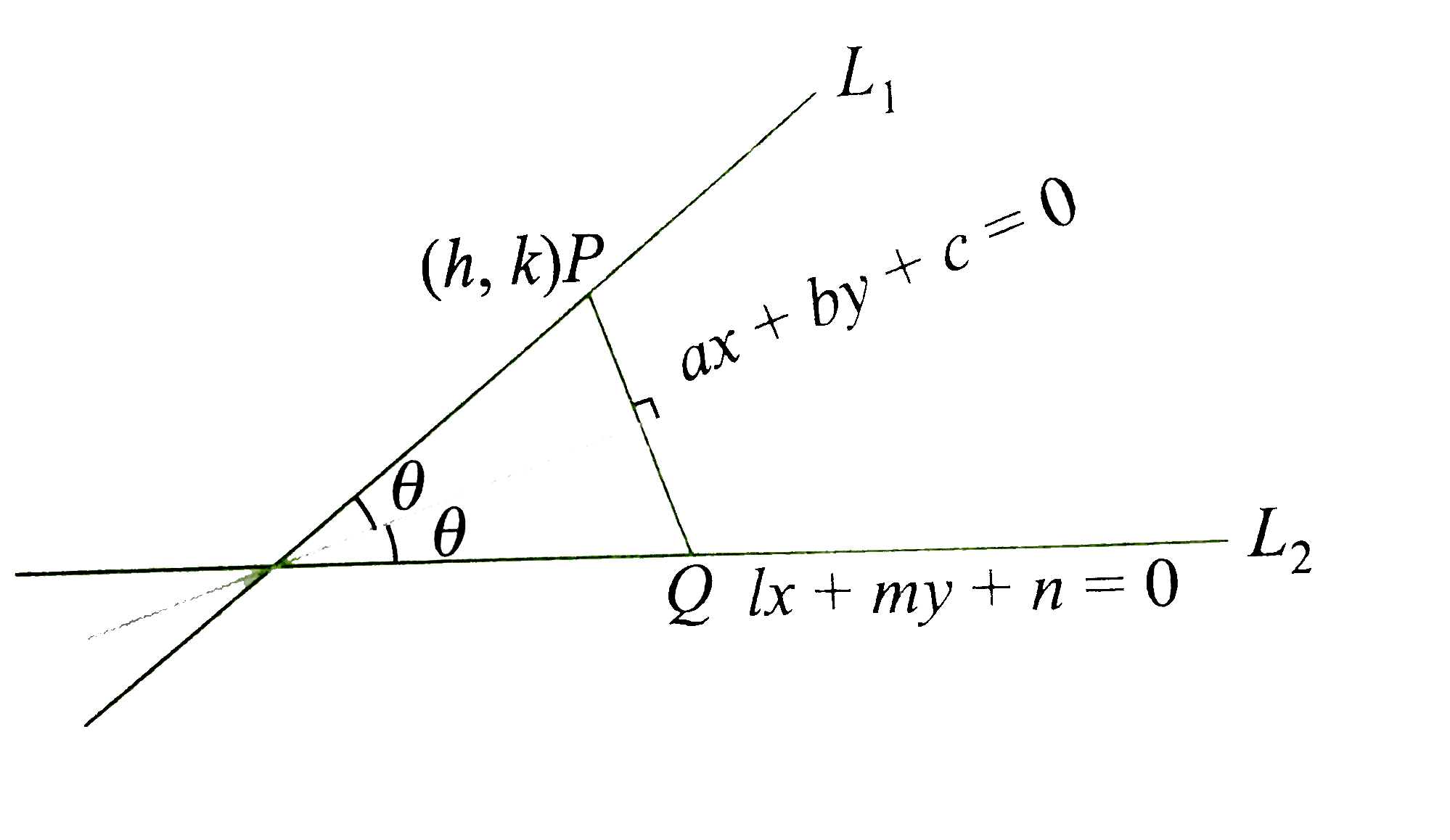
From the figure. Image of point P(h,k) on required line in the line $L_{1} lies on line L_{2}$ .
So, equation of required line is locus of point P such that its image in line $L_{1} lies on line L_{2}$ .
Let image of point P in line $L_{1} be Q (x_{1}, y_{1})$ .
$\Rightarrow \frac{x_{1} - h}{a} = \frac{y_{1} - k}{b} = \frac{2 (a h + b k + c)}{a^{2} + b^{2}}$
$\Rightarrow x_{1} = - \frac{2 a (a h + b k + c)}{a^{2} + b^{2}} + h$
$and y_{1} = - \frac{2 b (a h + b k + c)}{a^{2} + b^{2}} + k$
$Now, Q (x_{1}, y_{1}) lies on the line L_{2} .$
$∴ l [- \frac{2 a (a h + b k + c)}{a^{2} + b^{2}} + h] + m [- \frac{2 b (a h + b k + c)}{a^{2} + b^{2}} + k] + n = 0$
Therefore, equation of required line is
$2 (a l + m b) (a x + b y + c) - (a^{2} + b^{2}) (l x + m y + n) = 0$

Updated on:21/07/2023