Two lines l and m interset at the O and P is Point on a line n Passing through the point O such that P is equidistant from l and m. Prove that n is the bisectof the angle formed by l and m
Video Solution
Text Solution
Given two lines l and m intersect at the point O and P is a point on a line n Passing though O such that P is equidistant from l and m i.e PQ=PR
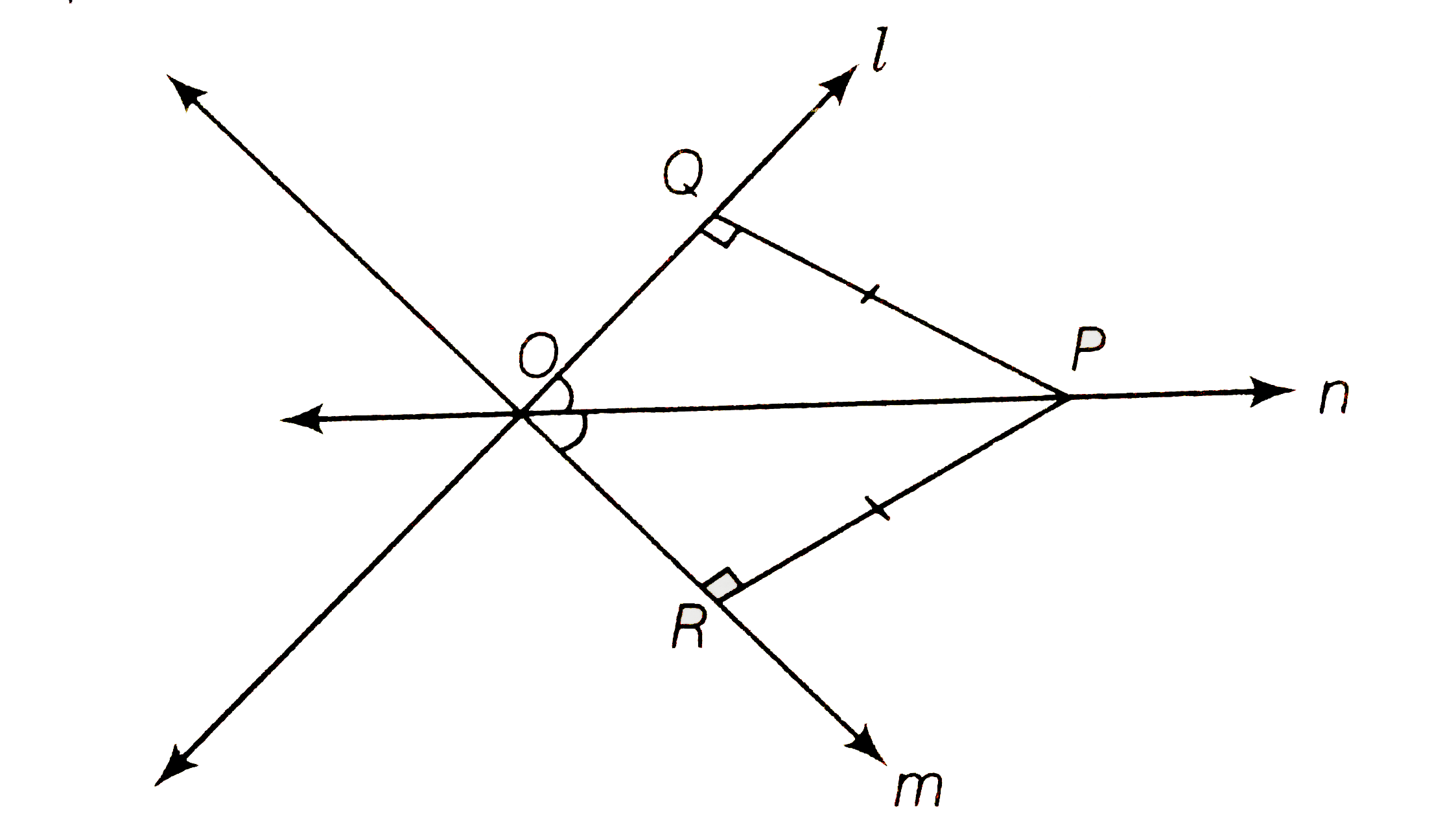
To prove n is the bisector of the angle formed by l and m i.e n is the bisector of ∠QOR.
Proof in ΔOQPandΔORP
∠PQO=∠PRO=90∘
[since P in equlidistant from l and m so PQ and PR should be Perpendicular to lines l and m repectively ]
OP =OP [Common side ]
PQ=PR [given ]
∴ΔOQP≅ΔORP [by RHS congruence rule ]
⇒∠POQ=∠POR [by CPCT]
Hence ,n is the bisector of ∠QOR
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
If l, m, n are in AP, then the line lx+my+n=0 will always pass through the point
A(−1,2)B(1,−2)C(2,4)D(3,2)The loucs of a point which is a equidistant from two non-intersecting lines l and m is a _____
Astraight line parallel to the line lBStraight line parallel to the line mCStraight line parallel to the line l and m and midway between themDstraight line that intersects both the line l and mLet L be the line passing through the point P (1, 2) such that its intercepted segment between the coordinate axes is bisected at P. If L1 is the line perpendicular to L and passing through the point (−2,1), then the point of intersection of L and L1 is :
A(45,125)B(1120,2910)C(310,175)D(35,2310)
If l,m,n are three lines such that l∣∣m and n⊥l, prove that n⊥m.
View SolutionIn figure P is a point equidistant from the lines l and m intersecting at point A. Show that the line n (along AP) bisects the angle between l and m.

View SolutionP is a point equidistant from two lines l and m intersecting at a point A see in figure, Show that AP bisects the angle between them. Figure
View SolutionIn figure l||m and M is the mid-point of the line segment AB. Prove that M is also the mid-point of line segment CD.

View SolutionLet L be the line passing through the point P (1,2) such that its intercepted segment between the co-ordinate axes is bisected at P. If L1 is the line perpendicular to L and psssing through the point (-2,1), then the point of intersection of L and L1 is
View Solution
