Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
Given : M is the midpoint of a chord AB of a circle C(O,r), and OM is produced to meet the circle at Q.
To Prove : ˆAQ≅ˆBQ
Construction : Join OA and OB
Proof : In ΔOMA and ΔOMB, we have
OA=OB [ each equal to r ]
OM = OM [ common]
AM = BM [ ∵ M is the midpoint of AB]
∴ΔOMA≅ΔOMB [ by SSS- congruence]
⇒∠AOM=∠BOM [ c.p.c.t.]
⇒m(ˆAQ)=m(ˆBQ)⇒ˆAQ≅ˆBQ
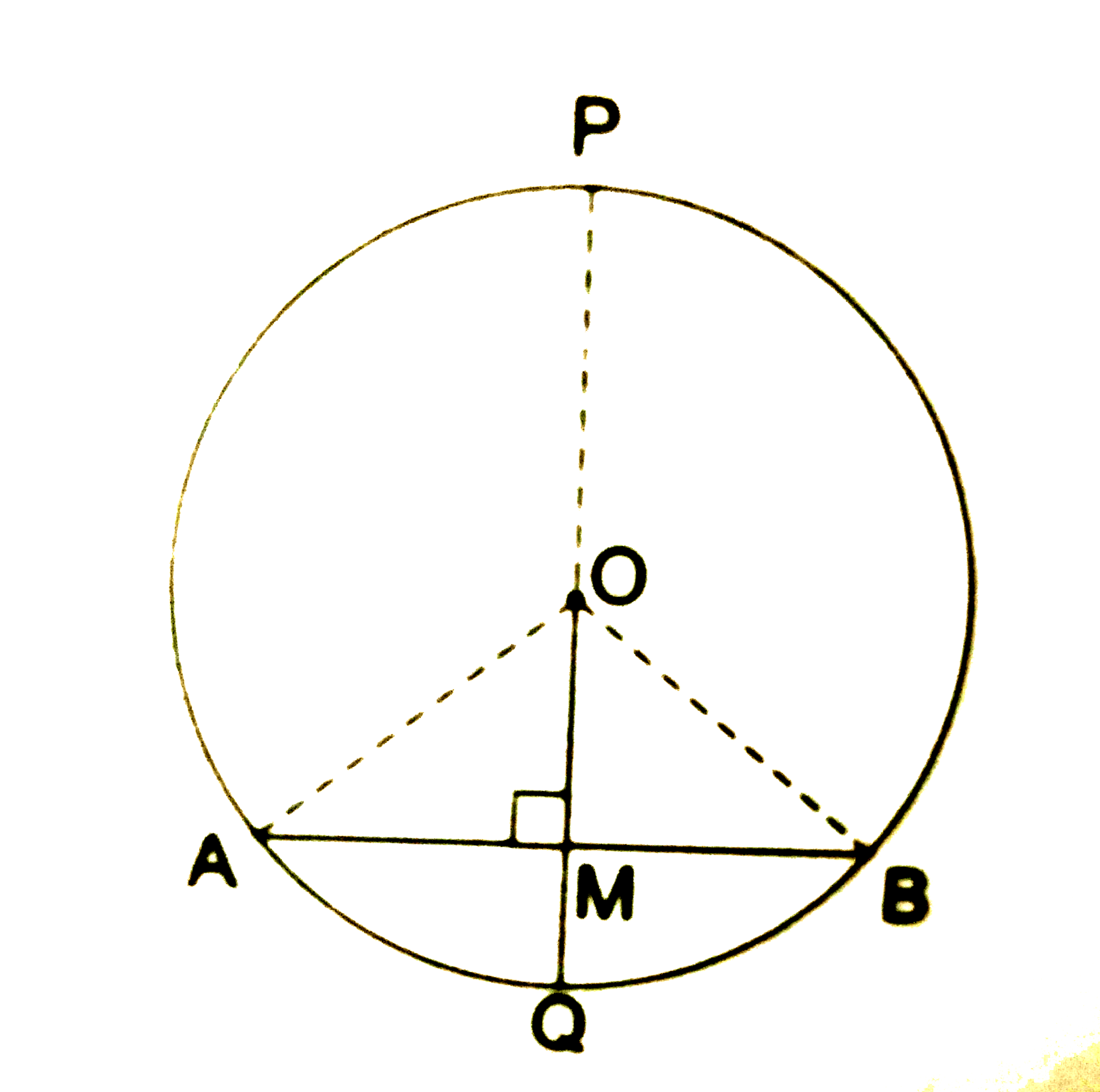
Note : MO when produced to P also passes through the midpoint of the corresponding major arc.
Similar Questions
Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
View SolutionProve that the line joining the mid-points of two parallel chords of a circle passes through the centre.
View SolutionProve that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
View SolutionProve that the line segment joining the points of contact of two parallel tangents of a circle, passes through its centre.
View SolutionProve that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
View Solutionprove that the line joining the mid-point of two equal chords of a circle subtends equal angles with the chord.
View SolutionFind the locus of the mid-points of chords of constant length in a circle
View SolutionProve that tangent drawn at the mid point of the are of a circle is pallelar to the chord joing the ends of point of the are
View SolutionThe line joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionProve that the line segment joining the mid points of two sides of a triangle is parallel to its third side.
View Solution
