Seven girls G1,G2,G3, …, G7 are such that their ages are in order G1<G2<G3<…<G7. Five girls are selected at random and arranged in increasing order of their ages. The probability that G5 and G7 are not consecutive is
A
2021
B
1921
C
1721
D
1321
Video Solution
Text Solution
The correct Answer is:C
Required probability = 1 - P (G5 and G7 are consecutive)
=1−.4C3.7C5=1721
|
Topper's Solved these Questions
- PROBABILITY I Book:CENGAGEChapter:PROBABILITY I Exercise:Exercise (Multiple)
Explore 8 Videos
- PROBABILITY I Book:CENGAGEChapter:PROBABILITY I Exercise:Exercise (Comprehension)
Explore 14 Videos
- PROBABILITY I Book:CENGAGEChapter:PROBABILITY I Exercise:Exercise 9.3
Explore 7 Videos
- PROBABILITY AND STATISTICSBook:CENGAGEChapter:PROBABILITY AND STATISTICSExercise:Question Bank
Explore 37 Videos
- PROBABILITY IIBook:CENGAGEChapter:PROBABILITY IIExercise:NUMARICAL VALUE TYPE
Explore 2 Videos
Similar Questions
Knowledge Check
G1 and G2 genetically identical because
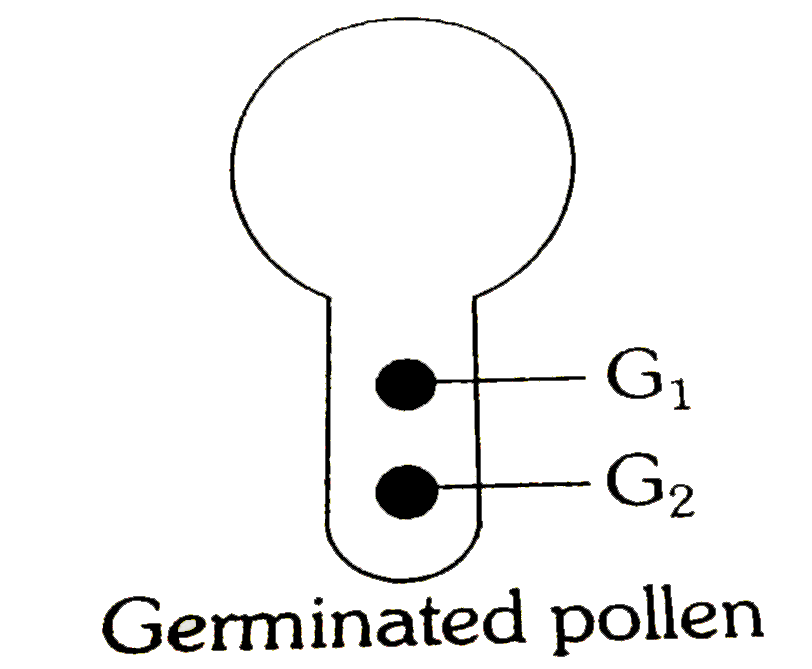 AThey are products of meiosis IBThey are products of amitosisCThey are products of meiosisDThey are products of mitosis
AThey are products of meiosis IBThey are products of amitosisCThey are products of meiosisDThey are products of mitosisG1,SandG2 are stages of
AInterphaseBProphaseCMetaphaseDAnaphaseIf A1,A2 be two A.M.'s and G1,G2 be two G.M.,s between a and b, then A1+A2G1G2 is equal to
Aa+b2abB2aba+bCa+babDa+b√ab
If a is A.M. of b and c and c,G1,G2,b are in G.P., then find the value of G31+G32−2abc
View SolutionWrite order of the following reaction:
2NH3(g)→N2(g)+3H2(g)
View SolutionThere are three girls G1,G2,G3 and three boys B1,B2,B3 in a school talk. The restriction is no two girls should speak together. If in an arrangement B1 speaks first what is the position of B2 and G2?
View SolutionIf G1.G2,g3 are three geometric means between
two positive numbers a and b , then g1g3 is equal to
View SolutionIf A1,A2 be two A.M.'s and G1,G2 be two G.M.,s between a and b, then A1+A2G1G2 is equal to
View Solution