Show that (p∨q)→r≡(p→r)∧(q→r) Solution in Tamil
Truth table for (p∨q)→r and (p→r)∧(q→r).
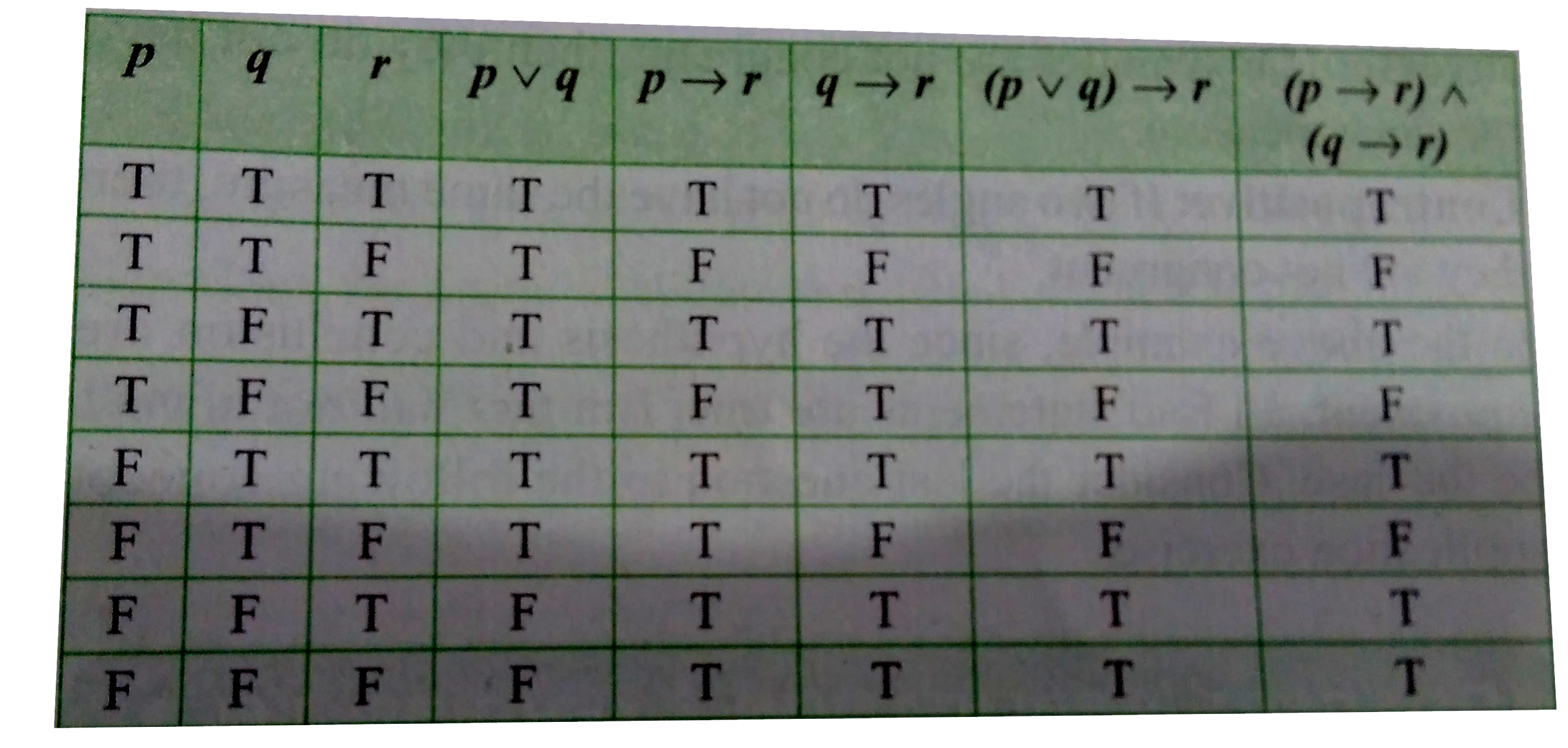
It is evident from the last two columns that
(p∨q)→r≡(p→r)∧(q→r)
Note:
1p→q≡(~p)∨q
2.p↔q≡(p→q)∧(q→p)
3.p↔q≡(~p∨q)∧(p∨~q)
Topper's Solved these Questions
- MATHMETICAL REASONINGBOOK - CENGAGECHAPTER - MATHMETICAL REASONINGEXERCISE - Exercise 10.1
8 Videos
- MATHMETICAL REASONINGBOOK - CENGAGECHAPTER - MATHMETICAL REASONINGEXERCISE - Exercise 10.2
5 Videos
- LOGARITHM AND ITS PROPERTIESBOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - JEE Previous Year
5 Videos
- MATRICESBOOK - CENGAGECHAPTER - MATRICESEXERCISE - Multiple Correct Answer
7 Videos
Similar Questions
Write down the truth table for the compound statements :
(~p∨q)∧(~p∧~q)
View SolutionShow that (p∧q)∨(~p)∨(p∧~q) is a tautology
View SolutionShow that the equation esinx−e−sinx−4=0 has no real solution.
View SolutionIf A=⎡⎢⎣100101010⎤⎥⎦, then
View SolutionShow that ∣∣ ∣ ∣∣0p−qp−rq−p0q−rr−pr−q0∣∣ ∣ ∣∣=0
View SolutionThe logical statement [~(~p∨q)∨(p∧r)]∧(~q∧r) is equivalent to (a) (~p∧~q)∧r (b) ~p∨r (c) (p∧r)∧~q (d) (p∧~q)∨r
View SolutionShow that (p∨q)→r≡(p→r)∧(q→r)
View SolutionConstruct the truth table for the followings statements :
(a) (p∧q)→~p (b)(p∧q)→(p∨q)
(c) (p∧q)→r (d)[p∧(~r)]→(q∨r)
View SolutionIf the statements p and q are true and the statement r and s are false, find the truth values of :
(1) ~[(p∨q)∧~r]∧{[(~p∨q)∨(~r)]∨s}
(2) p∧[q∧(~p∧r)∨~s]∨~r.
View SolutionIf the statement p and q are true and the statements r and s are false, fing the truth values of :
(1) (~p∨q)→(s∧~r)
(2) [p∧(q∧r)]∨[(p∨q)∧(~r∨s)]
(3) ~[(~p∧r)∨(s→~q)]⇔(p∧r).
View Solutionकिन्ही तीन कथनो p,q व r के लिए सिद्ध कीजिये कि
p∨(q∧r)≡(p∨q)∧(p∨r)
View SolutionIf p, q and r are simple propositions, then (~pvvq) rarr r If true, p, q and r will be respectively
View SolutionShow that (p∨q)→r≡(p→r)∧(q→r)
View SolutionConstruct the truth table for the followings statements :
(a) (p∧q)→~p (b)(p∧q)→(p∨q)
(c) (p∧q)→r (d)[p∧(~r)]→(q∨r)
View SolutionThe logical statement [~(~p∨q)∨(p∧r)]∧(~q∧r) is equivalent to (a) (~p∧~q)∧r (b) ~p∨r (c) (p∧r)∧~q (d) (p∧~q)∨r
View Solution
