Statement 1: There exist two points on the x−11=y−1=z+22 which are at a distance of 2 units from point (1,2,−4). Statement 2: Perpendicular distance of point (1,2,−4) form the line x−11=y−1=z+22 is 1 unit.Solution in Bengali
Both the statements are true, and Statement 2 is the correct explanation for Statement 1.
Both the Statements are true, but Statement 2 is not the correct explanation for Statement 1.
Statement 1 is true and Statement 2 is false.
Statement 1 is false and Statement 2 is true.
The correct Answer is:b
Any point on the line x−11=y−1=z+22 is
B(t+1,−t,2t−2),t∈R.
Also, AB is perpendicular to the line, where A is (1,2,−4). Thus,
1(t)−(−t−2)+2(2t+2)=0
or 6t+6=0
or t=−1
Point B is (0,1,−4)
Hence, AB=√1+1+0=√2
Topper's Solved these Questions
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - LINKED COMPREHENSION TYPE
12 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - MATRIX-MATCH TYPE
5 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CORRECT ANSWER TYPE
17 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions
291 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)
4 Videos
Similar Questions
Given four points A(2,1,0),B(1,0,1),C(3,0,1)andD(0,0,2). Point D lies on a line L orthogonal to the plane determined by the points A, B and C.
The equation of the plane ABC is
View Solution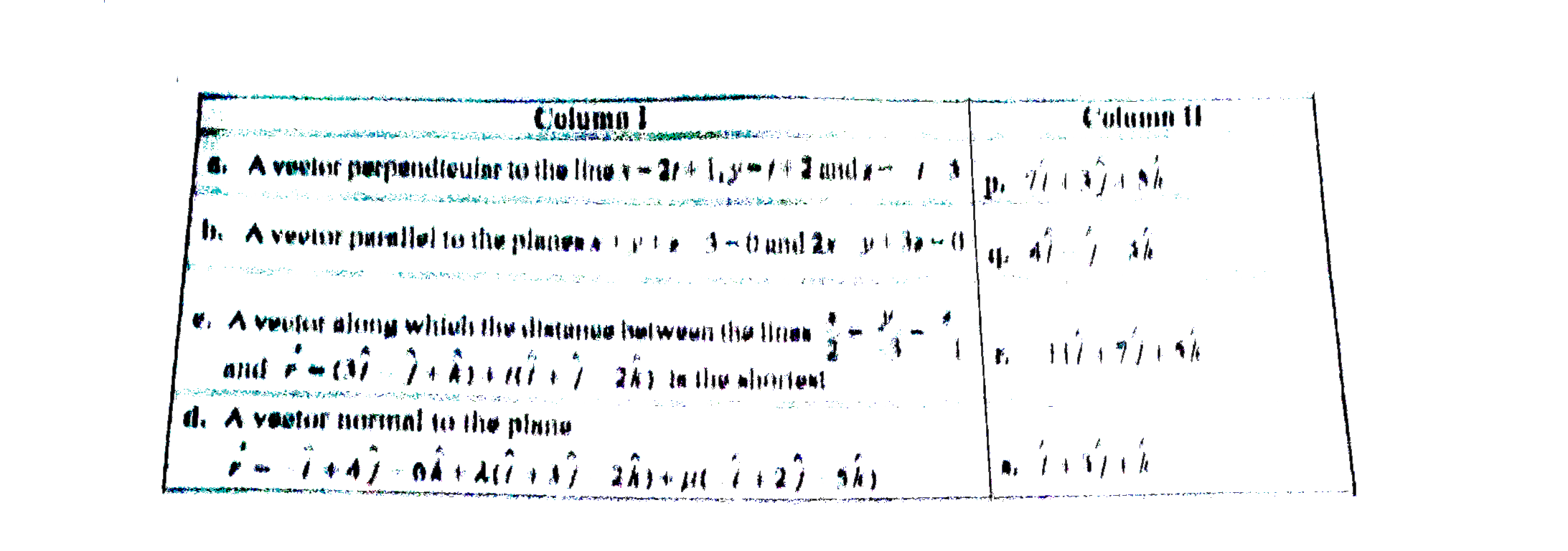
View SolutionLet PM be the perpendicular from the point P(1,2,3) to the x−y plane. If →OP makes an angle θ with the positive direction of the z− axis and→OM makes an angle φ with the positive direction of x− axis, whereO is the origin and θandφ are acute angels, then a. cosθcosφ=1/√14 b. sinθsinφ=2/√14 c. tanφ=2 d. tanθ=√5/3
View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
View SolutionStatement 1: There exist two points on the x−11=y−1=z+22 which are at a distance of 2 units from point (1,2,−4). Statement 2: Perpendicular distance of point (1,2,−4) form the line x−11=y−1=z+22 is 1 unit.
View SolutionThe perpendicular distance of the point (1,2,3) from the line (x-1)/(2)=(y-3)/(1)=(z-4)/(1)
View SolutionStatement 1: A point on the line x+23=y+12=z−32 at a distance 3√2 from the point (1,2,3) lies on the lne x+75=y+t4=z−21
Statement 2: If d is the distance between the point (−1,−5,−10) and the point of intersectionof the line x−23=y+14=z−212 with the plane x−y+z=5 then d=13
View SolutionStatement 1: The shortest distance between the lines x2=y−1=z2 and x−1−2=y−1−2=z−14 is √2. Statement 2: The shortest distance between two parallel lines is the perpendicular distance from any point on one of the lines to the other line.
View SolutionShow that the distance of the point of intersection of the line (x-2)/3=(y+1)/4=(z-2)/12 and the plane (x-y+z=5) from the point (-1,-5,-10) is 13 units.
View Solutionबिन्दु (1,2-3) से रेखा x−12=y−3−2=z1 पर लम्ब पाद ज्ञात कीजिए।
View SolutionFind the perpendicular distance of the point (1,1,1) from the line x−22=y+32=z−1.
View SolutionThe equation of line is x−12=y+1−2=z+11. The co-ordinates of the point on the line at a distance of 3 units from the point (1,−1,−1) is
View Solutionसरल रेखा x+61=y−22=z+14 पर बिंदु (-6, 2, -1) से 3 दूरी पर एक बिंदु के नियामक हैं
View Solutionबिंदु (5,4,5) से रेखा x−11=y−22=z−33 की लम्बवत् दूरी ज्ञात कीजिए ।
View SolutionThe distance of the point (1, 2, 1) from the line x−12=y−21=z−32 is
View Solution
