Study the diagram. The line l is perpendicular to line m (a) Is (b) Does bisect CG = (c) Identify any two line segments for which PE is the perpendicular bisector. (d) Are these true? (i) (ii) (iii)
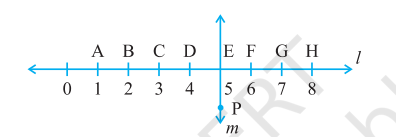
(a) Yes, CE = EG
Since, CE = 2 units and EG = 2 units
Hence, CE = EG.
(b) Yes, PE bisects CG, because CE = EG.
(c) The two line segments for which PE is perpendicular bisector are: BG and DF
(d) Yes, all three points are true
Since, AC = 2 units and FG = 1 units
CD = 1 units and GH = 1 units
BC = 1 units and EH = 3 units.
Hence,
Topper's Solved these Questions
Similar Questions
Study the diagram. The line l is perpendicular to line m (a) Is CE=EG (b) Does PE bisect CG = (c) Identify any two line segments for which PE is the perpendicular bisector. (d) Are these true? (i) AC>EG (ii) CD=GH (iii) BC<EH

View SolutionTwo chords AB and CD of a circle are parallel and a line l is the perpendicular bisector of AB. Show that l bisects CD
View Solution
Knowledge Check
Two particles each of mass 'm' are placed at A and C are such AB=BC=L. The gravitational force on the third particle placed at D at a distance L on the perpendicular bisector of the line AC is
AGm2L2 along BDBGm2√2L2 along DBCGm2L2 along ACDGm2√2L2 along BDIf p is the length of the perpendicular from the origin to the line xa+yb=1, then which of the following is ture ?
AI , II and IIIBI onlyCI and II onlyDII only
Two chords AB and CD of a circle are parallel and a line l is the perpendicular bisector of AB . Show that l bisects CD.
View SolutionWhich of the following are models for perpendicular lines :
(a) The adjacent edges of a table top.
(b) The lines of a railway track.
(c) The line segments forming the letter ‘L’.
(d) The letter V.
View SolutionAssertion : The line l is parallel to the plane P. Reason: The normal of the plane P is perpendicular to the line l. (A) Both A and R are true and R is the correct explanation of A (B) Both A and R are true R is not te correct explanation of A (C) A is true but R is false. (D) A is false but R is true.
View SolutionIn the given figure, quadrilateral ABCD is a quadrilateral in which AB = AD and BC = DC. Prove that (i) AC bisects ∠A and ∠B, (ii) AC.is the perpendicular bisector of BD.
View SolutionIdentify the parallel and perpendicular lines :
(i) Railways tracks
(ii) Hands of a clock at 12:15 ltBrgt (iii) Upright pole to the ground
(iv) Two upright trees
View SolutionThe direction cosines of a line satisfy the relations λ(l+m)=nandmn+nl+lm=0. The value of λ, for which the two lines are perpendicular to each other, is a. 1 b. 2 c. 1/2 d. none of these
View Solution
