Suppose A is a 3×3 matrix consisting of integer entries that are chosen at random from the set {−1000,999,….,999,1000}. Let P be the probability that either A2=−I or A is diagonal, where I is the 3×3 identity matrix. Then
A
P<11018
B
P=11018
C
521018≤P≤531018
D
P>541018
Video Solution
Text Solution
The correct Answer is:A
A=[]3×3
A2=−I
Taking det
|A|2=−1(Not possible)
A→diagonal matrix
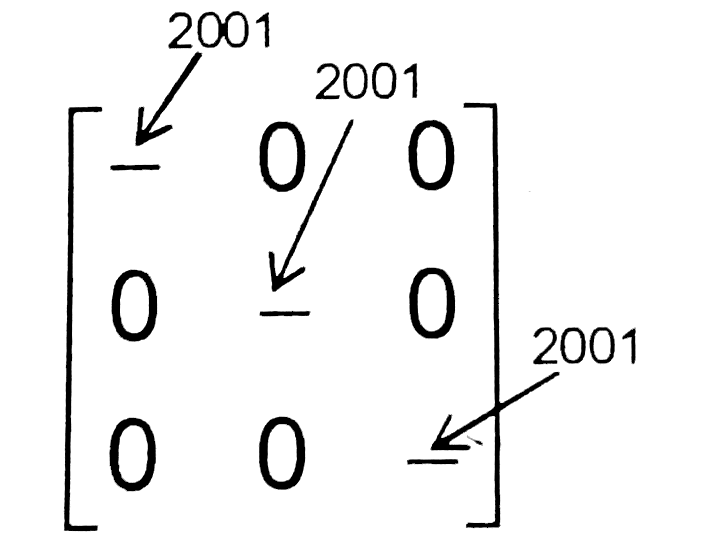
P=(2001)3(2001)9=1(2001)6
P=(1+2000)−6
=(2000−6)(1+1(2001))−6 less than ⊥
=126×1018(⏐↓)
P<11018
|
Topper's Solved these Questions
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - PART-2 MATHMATICS
10 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part 2 Mathematics
15 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part B- Mathematics
10 Videos
- KVPY 2021BOOK - KVPY PREVIOUS YEARCHAPTER - KVPY 2021EXERCISE - PART II MATHEMATICS
4 Videos
Similar Questions
Knowledge Check
If a number n is chosen at random from the set {1,2,3,4,….,1000}. Then , the probability that n is a number that leaves remainder 1, when divided by 7, is
A71500B1431000C72500D711000If for a 2×2 matrix A,A2+I=O, where I is identity matrix then A equals
A[1001]B[−i00−i]C[12−11]D[−100−1]If nine squares are chosen at random on a chess board, find the probability that they form a square of size 3×3.
A964C9B3664C9C664C9Dnone of these
A matrix of order 3×3 has determinant 2. What is the value of |A(3I)| , where I is the identity matrix of order 3×3 .
View SolutionIf A=⎡⎢⎣10−1213011⎤⎥⎦ then verify that A2+A=(A+I) , where I is 3×3 unit matrix.
View SolutionD is a 3×3 diagonal matrix. Which of the following
statements are not true?
View SolutionLet A be 3×3 matrix such that A is orthogonal idempotent then
View SolutionA matrix is chosen at random from the set of all 2×2 matrices with elements 0 and 1 only. The probability that determinant is positive, is
View Solution
