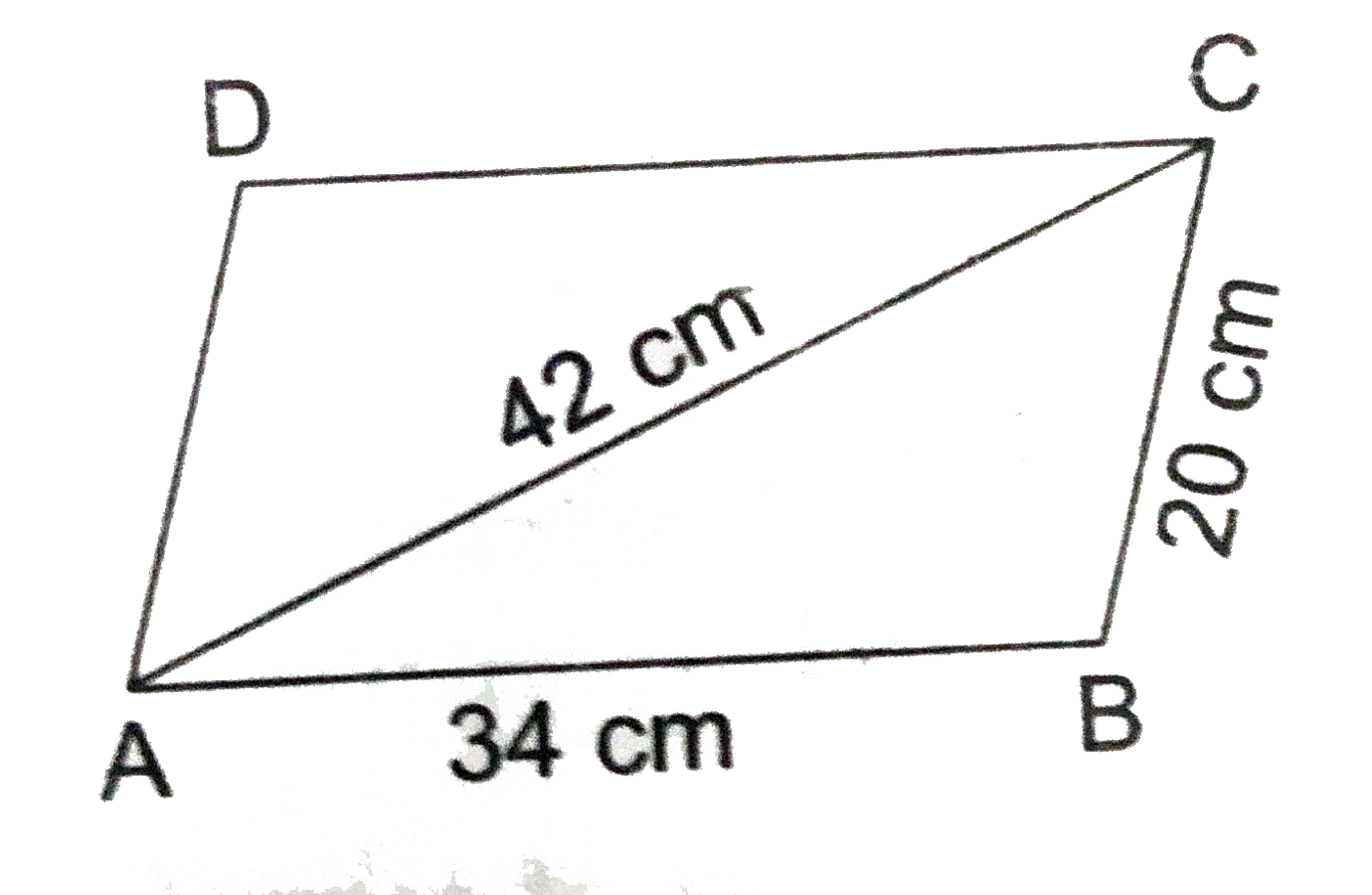
The adjacent sides of a parallelogram ABCD measure 34 cm and20 cm , and the diagonal AC measures 42 cm Find the area of the parallelogram.
To find the area of the parallelogram ABCD with adjacent sides measuring 34 cm and 20 cm, and diagonal AC measuring 42 cm, we can use Heron's formula. Here’s the step-by-step solution:
Step 1: Identify the sides of the triangles
The diagonal AC divides the parallelogram into two triangles, △ABC and △ADC. The sides of triangle ABC are:
- AB=34 cm
- BC=20 cm
- AC=42 cm
Step 2: Calculate the semi-perimeter s
The semi-perimeter s of triangle ABC is calculated using the formula:
s=AB+BC+AC2
Substituting the values:
s=34+20+422=962=48 cm
Step 3: Apply Heron's formula to find the area of triangle ABC
Heron's formula for the area A of a triangle is given by:
A=√s(s−a)(s−b)(s−c)
where a,b,c are the lengths of the sides of the triangle. Here, a=34 cm, b=20 cm, and c=42 cm.
Substituting the values into the formula:
A=√48(48−34)(48−20)(48−42)
Calculating each term:
- s−a=48−34=14
- s−b=48−20=28
- s−c=48−42=6
So the area becomes:
A=√48×14×28×6
Step 4: Simplify the expression under the square root
Calculating the product:
A=√48×14×28×6
We can simplify this step-by-step:
- First, calculate 48×6=288
- Then, calculate 14×28=392
- Now, multiply these results:
A=√288×392
Step 5: Factor and simplify
To simplify 288×392:
- 288=144×2=122×2
- 392=196×2=142×2
Thus:
288×392=(122×2)×(142×2)=122×142×4
Taking the square root:
A=12×14×2=336 cm2
Step 6: Calculate the area of the parallelogram
Since the area of the parallelogram is twice the area of triangle ABC:
Area of parallelogram=2×336=672 cm2
Final Answer
The area of the parallelogram ABCD is 672cm2.
---
The diagonal AC divides the parallelogram into two triangles, △ABC and △ADC. The sides of triangle ABC are:
- AB=34 cm
- BC=20 cm
- AC=42 cm
The semi-perimeter s of triangle ABC is calculated using the formula:
s=AB+BC+AC2
Substituting the values:
s=34+20+422=962=48 cm
Heron's formula for the area A of a triangle is given by:
A=√s(s−a)(s−b)(s−c)
where a,b,c are the lengths of the sides of the triangle. Here, a=34 cm, b=20 cm, and c=42 cm.
A=√48(48−34)(48−20)(48−42)
Calculating each term:
- s−a=48−34=14
- s−b=48−20=28
- s−c=48−42=6
A=√48×14×28×6
Calculating the product:
A=√48×14×28×6
We can simplify this step-by-step:
- First, calculate 48×6=288
- Then, calculate 14×28=392
- Now, multiply these results:
A=√288×392
To simplify 288×392:
- 288=144×2=122×2
- 392=196×2=142×2
288×392=(122×2)×(142×2)=122×142×4
Taking the square root:
A=12×14×2=336 cm2
Since the area of the parallelogram is twice the area of triangle ABC:
Area of parallelogram=2×336=672 cm2
The area of the parallelogram ABCD is 672cm2.
Topper's Solved these Questions
Similar Questions
The adjacent sides of a parallelogram ABCD measure 34 cm and 20 cm , and the diagonal ac measures 42 cm . Find the area of the parallelogram
View SolutionThe adjacent sides of a parallelogram ABCD measure 34 cm and20 cm , and the diagonal AC measures 42 cm Find the area of the parallelogram.
View Solution
Knowledge Check
Two adjacent sides of a parallelogram are 21 cms and 20 cms. The diagonal joining the end points of these two sides is 29 cms. The area of the parallelogram (in sq. cms) is
A240B120C210D420Two adjacent sides of a parallelogram are 10 cm and 12 cm. If its one diagonal is 14 cm long, find the area of the parallelogram.
A40√3cm2B49√3cm2C48√6cm2D58√6cm2The two adjacent sides of parallelogram are 25 cm and 40 cm respectively . The altitude drawn on the longer side is 18 cm, then the area of the parallelogram is :
A450cm2B720cm2C500cm2Dnone of these
The adjacent sides of a parallelogram are 5 cm and 9 cm. Its perimeter is __________.
View SolutionAdjacent sides of a parallelogram are 11 cm and 17 cm. If the length of one of its diagonal is 26 cm, find the length of the other.
View SolutionThe lengths of adjacent sides of a parallelogram are 5 cm and 12 cm. find the perimeter of the parallelogram.
View SolutionThe two adjacent sides of parallelogram are 25 cm and 40 cm respectively . The altitude drawn on the longer side is 18 cm, then the area of the parallelogram is :
View SolutionThe adjacent sides of a parallelogram are 6 cm and 8 cm and the angle between them is 30∘ . What is the area of the parallelogram ?
View Solution
