The ages of 40 students are given in the following table: Age(in yrs) 12 13 14 15 16 17 18 Frequency 2 4 6 9 8 7 4 Find the arithmetic mean.
The correct Answer is:15.35
To find the arithmetic mean of the ages of 40 students given in the table, we will follow these steps:
Step 1: Set up the table
We have the ages (x) and their corresponding frequencies (f) as follows:
| Age (x) | Frequency (f) |
|---------|---------------|
| 12 | 2 |
| 13 | 4 |
| 14 | 6 |
| 15 | 9 |
| 16 | 8 |
| 17 | 7 |
| 18 | 4 |
Step 2: Calculate the product of age and frequency (xf)
We will multiply each age by its corresponding frequency to get the xf column:
- For age 12: 12×2=24
- For age 13: 13×4=52
- For age 14: 14×6=84
- For age 15: 15×9=135
- For age 16: 16×8=128
- For age 17: 17×7=119
- For age 18: 18×4=72
Now, we can summarize this in a new table:
| Age (x) | Frequency (f) | xf |
|---------|---------------|------|
| 12 | 2 | 24 |
| 13 | 4 | 52 |
| 14 | 6 | 84 |
| 15 | 9 | 135 |
| 16 | 8 | 128 |
| 17 | 7 | 119 |
| 18 | 4 | 72 |
Step 3: Sum the xf values and the frequencies
Now we will find the total of the xf column and the total of the frequency column:
- Total of xf: 24+52+84+135+128+119+72=614
- Total of frequency (f): 2+4+6+9+8+7+4=40
Step 4: Calculate the arithmetic mean
The formula for the arithmetic mean is given by:
Mean=∑(xf)∑(f)
Substituting the values we found:
Mean=61440=15.35
Final Answer
The arithmetic mean of the ages of the 40 students is 15.35 years.
---
We have the ages (x) and their corresponding frequencies (f) as follows:
|---------|---------------|
| 12 | 2 |
| 13 | 4 |
| 14 | 6 |
| 15 | 9 |
| 16 | 8 |
| 17 | 7 |
| 18 | 4 |
We will multiply each age by its corresponding frequency to get the xf column:
- For age 13: 13×4=52
- For age 14: 14×6=84
- For age 15: 15×9=135
- For age 16: 16×8=128
- For age 17: 17×7=119
- For age 18: 18×4=72
|---------|---------------|------|
| 12 | 2 | 24 |
| 13 | 4 | 52 |
| 14 | 6 | 84 |
| 15 | 9 | 135 |
| 16 | 8 | 128 |
| 17 | 7 | 119 |
| 18 | 4 | 72 |
Now we will find the total of the xf column and the total of the frequency column:
- Total of frequency (f): 2+4+6+9+8+7+4=40
The formula for the arithmetic mean is given by:
The arithmetic mean of the ages of the 40 students is 15.35 years.
Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (B)
10 Videos
- MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (C)
10 Videos
- MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (E)
23 Videos
- MATRICESBOOK - ICSECHAPTER - MATRICESEXERCISE - Exercise 9D
25 Videos
- MIXED PRACTICE BOOK - ICSECHAPTER - MIXED PRACTICE EXERCISE - SET B
52 Videos
Similar Questions
The ages of 37 students in a class are given in the following table: Find the median
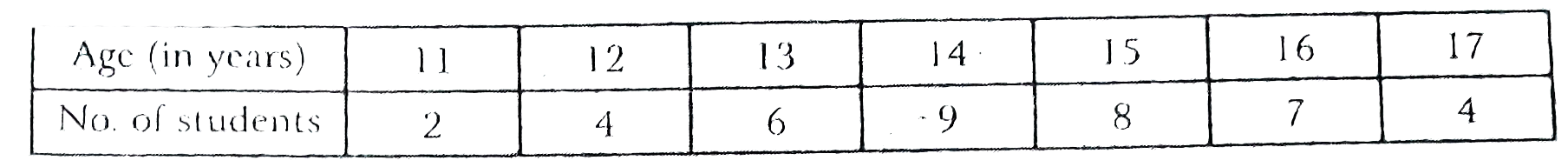
View SolutionThe age of 40 student of a class is given in the following table. Find their mean short cut method:
View SolutionThe ages (in years) of 50 students of a class in a school are given below:
Age (in years): 14 15 16 17 18
Numbers of students: 15 14 10 8 3
Find the mean age.
View SolutionThe following table gives the ages of 50 students of a class. Find the arithmetic mean of their ages.
View SolutionFind the mean deviation from the mean for the following data: Size: 1 3 5 7 9 11 13 15 Frequency: 3 3 4 14 7 4 3 4
View SolutionFind the mean deviation from the mean for the following data: Size: 1 3 5 7 9 11 13 15 Frequency: 3 3 4 14 7 4 3 4
View SolutionConstruct a frequency polygon for the following data:
Age (in year) 0-2 2-4 4-6 6-8 8-10 10-12 12-14 14-16 16-18
Frequency 2 4 6 8 9 6 5 3 1
View SolutionThe ages of 15 students in years) in a summer camp are as follows: 11, 14, 13, 15, 16, 10, 12, 12, 14, 11, 10, 12, 13, 15, 11 Find: The mean age.
View SolutionThe ages of 15 students in years) in a summer camp are as follows: 11, 14, 13, 15, 16, 10, 12, 12, 14, 11, 10, 12, 13, 15, 11 Find:
the range of age.
View SolutionThe ages of 15 students (in years) in a summer camp are as follows: 11, 14, 13, 15, 16, 10, 12, 12, 14, 11, 10, 12, 13, 15, 11 Find:
the minimum age.
View Solution

