The amount of time it takes to consume a buffalo carcass is inversely proportional to the number of vultures. IF it takes 12 vultures 3 day to consume a buffalo, how many fewer hours will it take there are 4 more vultures?
18
54
The correct Answer is:C
To solve the problem, we need to follow these steps:
Step 1: Understand the relationship
The time taken to consume a buffalo carcass (t) is inversely proportional to the number of vultures (v). This can be expressed mathematically as:
t∝1v
This means:
t=kv
where k is a constant.
Step 2: Find the constant k
We know that 12 vultures take 3 days to consume a buffalo. We can substitute these values into the equation to find k:
t=3 days
v=12
Substituting these into the equation:
3=k12
To find k, we multiply both sides by 12:
k=3×12=36
Step 3: Calculate time with 16 vultures
Now we need to find out how much time it takes with 4 more vultures, which means we will have 16 vultures (12 + 4). We can use the constant k we found:
t=kv=3616
Calculating this gives:
t=2.25 days
Step 4: Convert days to hours
Since we need the answer in hours, we convert days to hours:
t=2.25 days×24 hours/day=54 hours
Step 5: Calculate the time with 12 vultures
Next, we need to find out how many hours it took with 12 vultures:
t=3 days
Converting this to hours:
t=3 days×24 hours/day=72 hours
Step 6: Find the difference in hours
Finally, we find how many fewer hours it takes with 16 vultures compared to 12 vultures:
Difference=72 hours−54 hours=18 hours
Final Answer
Thus, it takes 18 fewer hours when there are 4 more vultures.
---
The time taken to consume a buffalo carcass (t) is inversely proportional to the number of vultures (v). This can be expressed mathematically as:
t∝1v
This means:
t=kv
where k is a constant.
We know that 12 vultures take 3 days to consume a buffalo. We can substitute these values into the equation to find k:
t=3 days
v=12
Substituting these into the equation:
3=k12
To find k, we multiply both sides by 12:
k=3×12=36
Now we need to find out how much time it takes with 4 more vultures, which means we will have 16 vultures (12 + 4). We can use the constant k we found:
t=kv=3616
Calculating this gives:
t=2.25 days
Since we need the answer in hours, we convert days to hours:
t=2.25 days×24 hours/day=54 hours
Next, we need to find out how many hours it took with 12 vultures:
t=3 days
Converting this to hours:
t=3 days×24 hours/day=72 hours
Finally, we find how many fewer hours it takes with 16 vultures compared to 12 vultures:
Difference=72 hours−54 hours=18 hours
Thus, it takes 18 fewer hours when there are 4 more vultures.
Topper's Solved these Questions
- ADVANCED ARITHMETICBOOK - PRINCETONCHAPTER - ADVANCED ARITHMETICEXERCISE - Advanced Arithmetic Drill: Calculator-Permitted Section
16 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #9
3 Videos
- ALEGBRABOOK - PRINCETONCHAPTER - ALEGBRAEXERCISE - QUICK QUIZ #6
3 Videos
Similar Questions
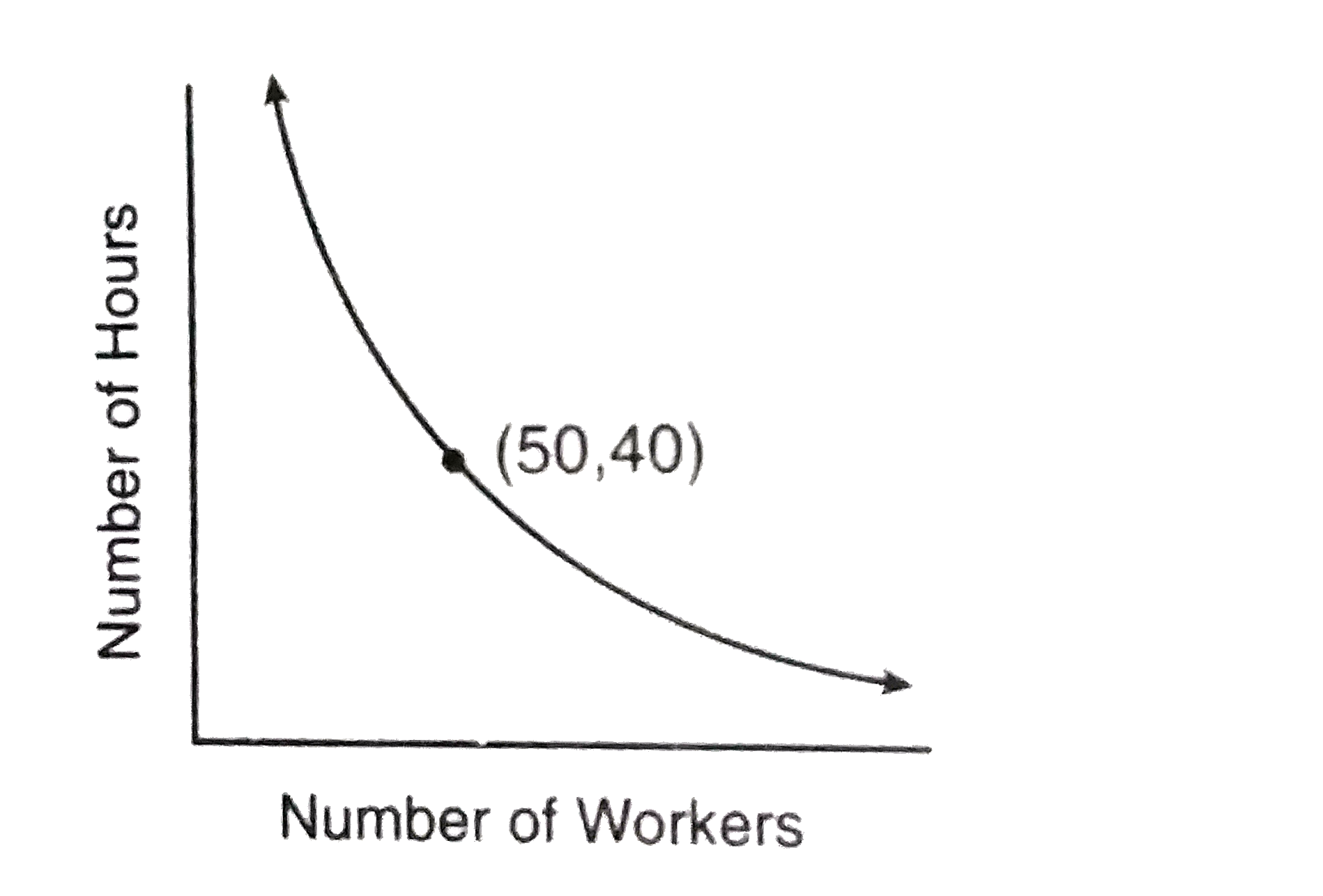
A political campaign organizer has determined that the number of hours needed to get out a mailing for her candiadate is inversely related to the number of campaign workers she has. If she uses the information in the accompanying graph, how many hours would it take to do the mailing if 125 workers are used?
View SolutionIf 10 men take 6 days to construct a garage, how many more men are needed if the garage is to be constructed in 5 days ?
View Solution
Knowledge Check
The amount of time it take to consume a buffalo carcasess is inversly proporation to the number of vultures. If it takes 12 vultures 3 days to consume a buffalo, how many fewer hours will it take if there are 4 more vultures?
A14B34C18D54A cyclist travels at 12 miles per hour. How many minutes will it take him to travel 24 miles
A1B120C30D60
5 men can complete a job in 8 days. How many days will it take if 12 men do the job?
View SolutionThe amount of time that three people worked on a special project was in the ratio of 2 to 3 to 5. If the project took 110 hours, how many more hours did the person who worked the most hours work than the person who worked the least hours?
View SolutionA new printing press can print 5,000 flyers in half the amount of time it take for an older printing press the same 5,000 flayers. Working together, the two printing presses can complete the entire job in 3 hours. How long would it take the faster printing press working alone to complete the job?
View SolutionA man working 8 hours a day takes 5 days to complete a project. How many hours a day must he work to complete it in 4 days ?
View SolutionWater is being poured into a tank at the rate of approximately 4 cubic feet per hour. If the tank is 6 feet long, 4 feet wide, and 8 feet deep, how many hours will it take to fill up the tank ?
View SolutionGiven a starting population of 100 bacteria, the formula b(t)=100(2t) can be used to determine the number of bacteria, b, after t periods of time. If each time period is 15 minutes long, how many minutes will it take for the population of bacteria to reach 51,200?
View Solution
