The angle of elevation of the top of a tower at a point on the ground, 50 m away from the foot of the tower, is 60°. Find the height of the tower.
The correct Answer is:50√3m
To find the height of the tower given the angle of elevation and the distance from the tower, we can use trigonometric ratios. Here’s a step-by-step solution:
Step 1: Understand the Problem
We have a right triangle formed by the height of the tower, the distance from the point on the ground to the foot of the tower, and the line of sight to the top of the tower. The angle of elevation from the point on the ground to the top of the tower is given as 60°, and the distance from the foot of the tower to the point on the ground is 50 m.
Step 2: Identify the Right Triangle
Let:
- AB be the height of the tower (which we need to find).
- AC be the distance from the foot of the tower to the point on the ground (50 m).
- BC be the line of sight from the point on the ground to the top of the tower.
Step 3: Use the Tangent Function
In a right triangle, the tangent of an angle is defined as the ratio of the opposite side to the adjacent side. Here, we can use the tangent of the angle of elevation:
tan(θ)=OppositeAdjacent=ABAC
Given that θ=60° and AC=50m:
tan(60°)=AB50
Step 4: Substitute the Value of Tangent
The value of tan(60°) is √3:
√3=AB50
Step 5: Solve for the Height of the Tower (AB)
To find AB, we can rearrange the equation:
AB=50⋅√3
Step 6: Calculate the Height
Now, we can calculate the height:
AB=50⋅√3≈50⋅1.732≈86.6m
Final Answer
The height of the tower is approximately 86.6m.
---
We have a right triangle formed by the height of the tower, the distance from the point on the ground to the foot of the tower, and the line of sight to the top of the tower. The angle of elevation from the point on the ground to the top of the tower is given as 60°, and the distance from the foot of the tower to the point on the ground is 50 m.
Let:
- AB be the height of the tower (which we need to find).
- AC be the distance from the foot of the tower to the point on the ground (50 m).
- BC be the line of sight from the point on the ground to the top of the tower.
In a right triangle, the tangent of an angle is defined as the ratio of the opposite side to the adjacent side. Here, we can use the tangent of the angle of elevation:
tan(θ)=OppositeAdjacent=ABAC
Given that θ=60° and AC=50m:
tan(60°)=AB50
The value of tan(60°) is √3:
√3=AB50
To find AB, we can rearrange the equation:
AB=50⋅√3
Now, we can calculate the height:
AB=50⋅√3≈50⋅1.732≈86.6m
The height of the tower is approximately 86.6m.
Topper's Solved these Questions
- INTRODUCTION TO TRIGNOMETRY AND ITS APPLICATIONS BOOK - EDUCART PUBLICATIONCHAPTER - INTRODUCTION TO TRIGNOMETRY AND ITS APPLICATIONS EXERCISE - SHORT ANSWER (SA-I) TYPE QUESTIONS
15 Videos
- INTRODUCTION TO TRIGNOMETRY AND ITS APPLICATIONS BOOK - EDUCART PUBLICATIONCHAPTER - INTRODUCTION TO TRIGNOMETRY AND ITS APPLICATIONS EXERCISE - SHORT ANSWER (SA-II) TYPE QUESTIONS
27 Videos
- INTRODUCTION TO TRIGNOMETRY AND ITS APPLICATIONS BOOK - EDUCART PUBLICATIONCHAPTER - INTRODUCTION TO TRIGNOMETRY AND ITS APPLICATIONS EXERCISE - OBJECTIVE TYPE QUESTIONS ( FILL IN THE BLANKS )
11 Videos
- COORDINATE GEOMETRY BOOK - EDUCART PUBLICATIONCHAPTER - COORDINATE GEOMETRY EXERCISE - LONG ANSWER (LA) TYPE QUESTIONS (4 MARKS)
7 Videos
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLES BOOK - EDUCART PUBLICATIONCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - LONG ANSWER Type Questions [4 marks]
10 Videos
Similar Questions
The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower at a point on the ground 20 m from the foot of the tower is 30∘. What is the height of the tower?
View Solution
Knowledge Check
Assertion (A): The angle of elevation of the top of the tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. The height of the tower is 10 m.
Reason (R): The angle of depression from B to A and Angle of elevation from A to B are equal.ABoth A and R are true and R is the correct explanation of A.BBoth A and R are true and R is not correct explanation of A.CA is true but R is false.DA is false but R is true.The angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower , is 30∘ . Then , the height ( in m) of the tower is .
A10√3B30√3C10√3D10
The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution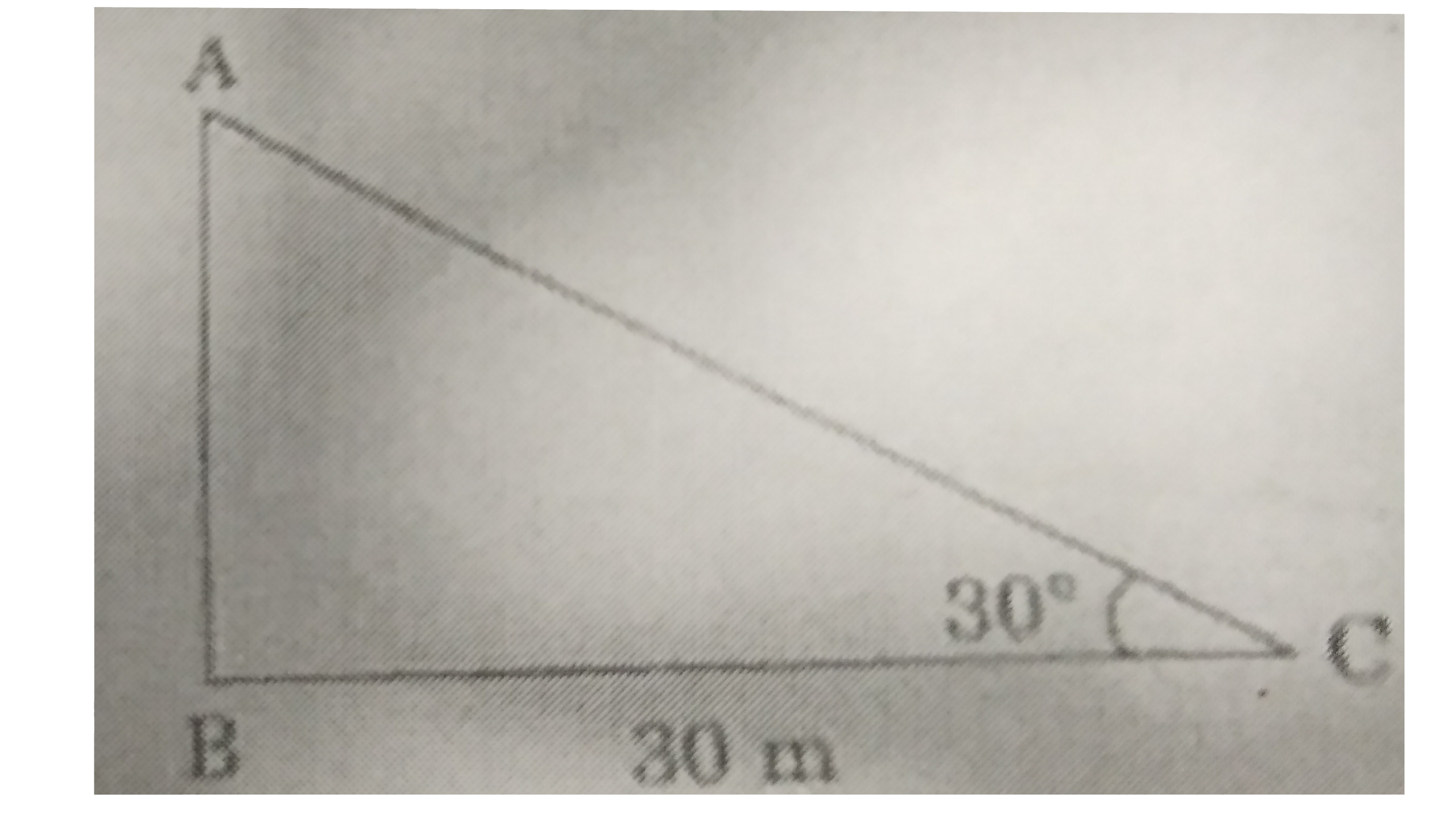
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View Solution