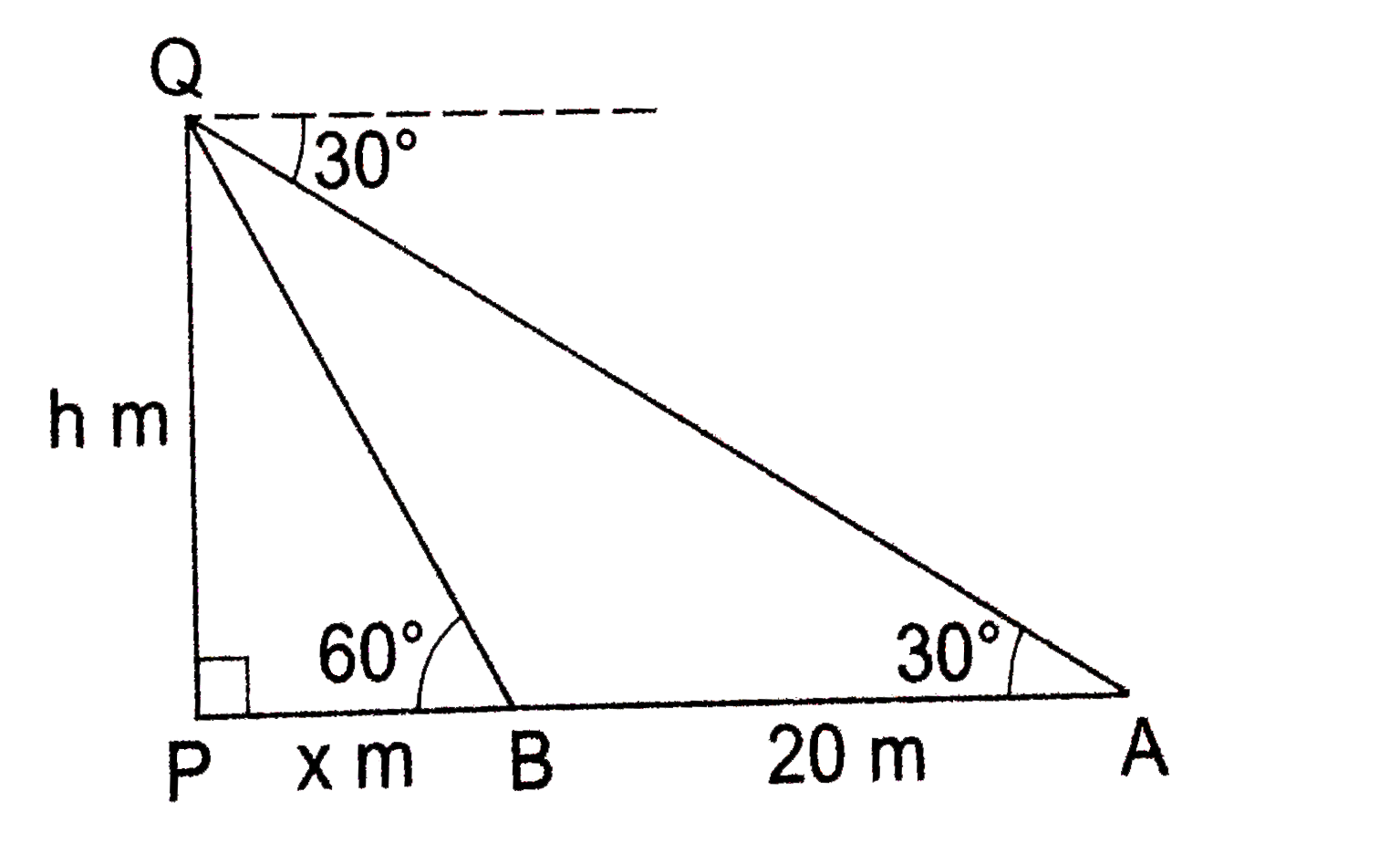
The angle of elevation of the top of a tower from a point A on the ground is 300. On moving a distance of 20 metres towards the foot of the tower to a point B the angle of elevation increases to 600. Find the height of the ttower and the distance of the tower from the point A.
The correct Answer is:17.32m,30m
From right ΔAPQ, we have
PQPA=tan30∘⇒hx+20=1√3 .
⇒h=x+20√3……….(i).
From right ΔBPQ , we have
PQPB=tan60∘⇒hx=√3⇒h=x√3………..(ii)
From (i) and (ii) , we get
(x+20)√3=x√3⇒x=10.
∴h=10√3=10×1.732=17.32.
Distance AP=(20+x)m=(20+10)m=30m.
Topper's Solved these Questions
- HEIGHTS AND DISTANCESBOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)
25 Videos
- HEIGHTS AND DISTANCESBOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)
25 Videos
- COORDINATE GEOMETRYBOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Multiple Choice Questions (Mcq)
34 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - RS AGGARWALCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - QUESTION
2 Videos
Similar Questions
The angle of elevation of a tower from a point on the same level as the foot of the tower is 300. On advancing 150 metres towards the foot of the tower, the angle of elevation of the tower becomes 600. Show that the height of the tower is 129.9 metres (Use √3=1.732 ).
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution
Knowledge Check
The angle of elevation of the to of a tower from a point A on the ground is 30∘. On moving a distance of 20 meters towards the foot of the tower to a point B, the angle of elevation increases to 60∘. What is the height of the tower ?
A√3 mB5√3 mC10√3 mD20√3 mThe angle of elevation of the top of a tower at any point on the ground is 30∘ and moving 20 metres towards the tower it becomes 60∘. The height of the tower is
A10 mB10√3 mC10√3 mDnone of these
The angle of elevation of the top of a tower from a point P on the ground is α. After walking a distance d meter towards the foot of the tower, angle of elevation is found to be β. Which angle of elevation is greater?
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower at a point on the ground is 30o . What will be the angle of elevation, if the height of the tower is tripled?
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View Solution
