The area of a cyclic quadrilateral ABCD is 3√34 . The radius of the circle circumscribing cyclic quadrilateral is 1.If AB=1 and BD=√3, then BC⋅CD is equal to
To solve the problem step by step, we will use the given information about the cyclic quadrilateral ABCD and apply the properties of triangles and cyclic quadrilaterals.
Given:
- Area of cyclic quadrilateral ABCD = 3√34
- Radius of the circumcircle R=1
- AB=1
- BD=√3
We need to find BC⋅CD.
Step 1: Understand the cyclic quadrilateral
A cyclic quadrilateral is one where all vertices lie on the circumference of a circle. The area can be calculated using the formula:
Area=12⋅d1⋅d2
where d1 and d2 are the lengths of the diagonals.
Step 2: Use the sine rule
From the sine rule in a triangle, we know that:
asinA=bsinB=csinC=2R
Here, R=1, so:
asinA=bsinB=csinC=2
Step 3: Calculate angle A
We can find angle A using the area of triangle ABD. The area of triangle ABD can be calculated as:
AreaABD=12⋅AB⋅BD⋅sinA
Substituting the known values:
3√34=12⋅1⋅√3⋅sinA
This simplifies to:
3√34=√32⋅sinA
Dividing both sides by √32:
sinA=3√34⋅2√3=32
Since sinA cannot exceed 1, we need to check our calculations.
Step 4: Calculate angle C
Using the property of cyclic quadrilaterals, we know that the opposite angles sum to 180∘. If A is 60∘, then C would be 120∘.
Step 5: Area of triangle BDC
The area of triangle BDC can be calculated as:
AreaBDC=12⋅BC⋅CD⋅sinC
Substituting sinC=sin120∘=√32:
AreaBDC=12⋅BC⋅CD⋅√32
Step 6: Total area of quadrilateral
The total area of the quadrilateral is:
3√34=√32+14BC⋅CD⋅√3
Setting this up gives:
3√34=√32+√34BC⋅CD
Multiplying through by 4 to eliminate the fractions:
3√3=2√3+√3BC⋅CD
This simplifies to:
√3BC⋅CD=3√3−2√3=√3
Dividing both sides by √3:
BC⋅CD=1
Final Answer:
Thus, BC⋅CD=1.
- Area of cyclic quadrilateral ABCD = 3√34
- Radius of the circumcircle R=1
- AB=1
- BD=√3
A cyclic quadrilateral is one where all vertices lie on the circumference of a circle. The area can be calculated using the formula:
Area=12⋅d1⋅d2
where d1 and d2 are the lengths of the diagonals.
From the sine rule in a triangle, we know that:
asinA=bsinB=csinC=2R
Here, R=1, so:
asinA=bsinB=csinC=2
We can find angle A using the area of triangle ABD. The area of triangle ABD can be calculated as:
AreaABD=12⋅AB⋅BD⋅sinA
Substituting the known values:
3√34=12⋅1⋅√3⋅sinA
This simplifies to:
3√34=√32⋅sinA
Dividing both sides by √32:
sinA=3√34⋅2√3=32
Since sinA cannot exceed 1, we need to check our calculations.
Using the property of cyclic quadrilaterals, we know that the opposite angles sum to 180∘. If A is 60∘, then C would be 120∘.
The area of triangle BDC can be calculated as:
AreaBDC=12⋅BC⋅CD⋅sinC
Substituting sinC=sin120∘=√32:
AreaBDC=12⋅BC⋅CD⋅√32
The total area of the quadrilateral is:
3√34=√32+14BC⋅CD⋅√3
Setting this up gives:
3√34=√32+√34BC⋅CD
Multiplying through by 4 to eliminate the fractions:
3√3=2√3+√3BC⋅CD
This simplifies to:
√3BC⋅CD=3√3−2√3=√3
Dividing both sides by √3:
BC⋅CD=1
Thus, BC⋅CD=1.
Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 7
10 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 8
5 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 5
7 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
51 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
In a cyclic quadrilateral ABCD , if ∠A=3∠C. Find ∠A
View SolutionIf the sides of a cyclic quadrilateral are 3,3,4,4, show that a circle be inscribed in it.
View SolutionIf ABCD is a cyclic quadrilateral such that 12tanA−5=0 and 5cosB+3=0 then tanC+tanD is equal to :
View SolutionIf ABCD is a cyclic quadrilateral such that 12tanA−5=0 and 5cosB+3=0 then tanC+tanD is equal to :
View SolutionABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC=140∘, than ∠BAC is equal to
View SolutionIn cyclic quadrilateral ABCD,∠A=3∠C and ∠D=5∠B. Find the measure of each angle of the quadrilateral.
View SolutionIf bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
View SolutionIn a cyclic quadrilateral ABCD, if AB || CD and ∠B=700, find the remaining angles.
View SolutionShow that in a quadrilateral ABCD AB+BC+CD+DA>AC+BD
View SolutionIn the given figure quadrilateral ABCD is circumscribed and AD⊥DC. Find x if radius of circle is 10 cm.
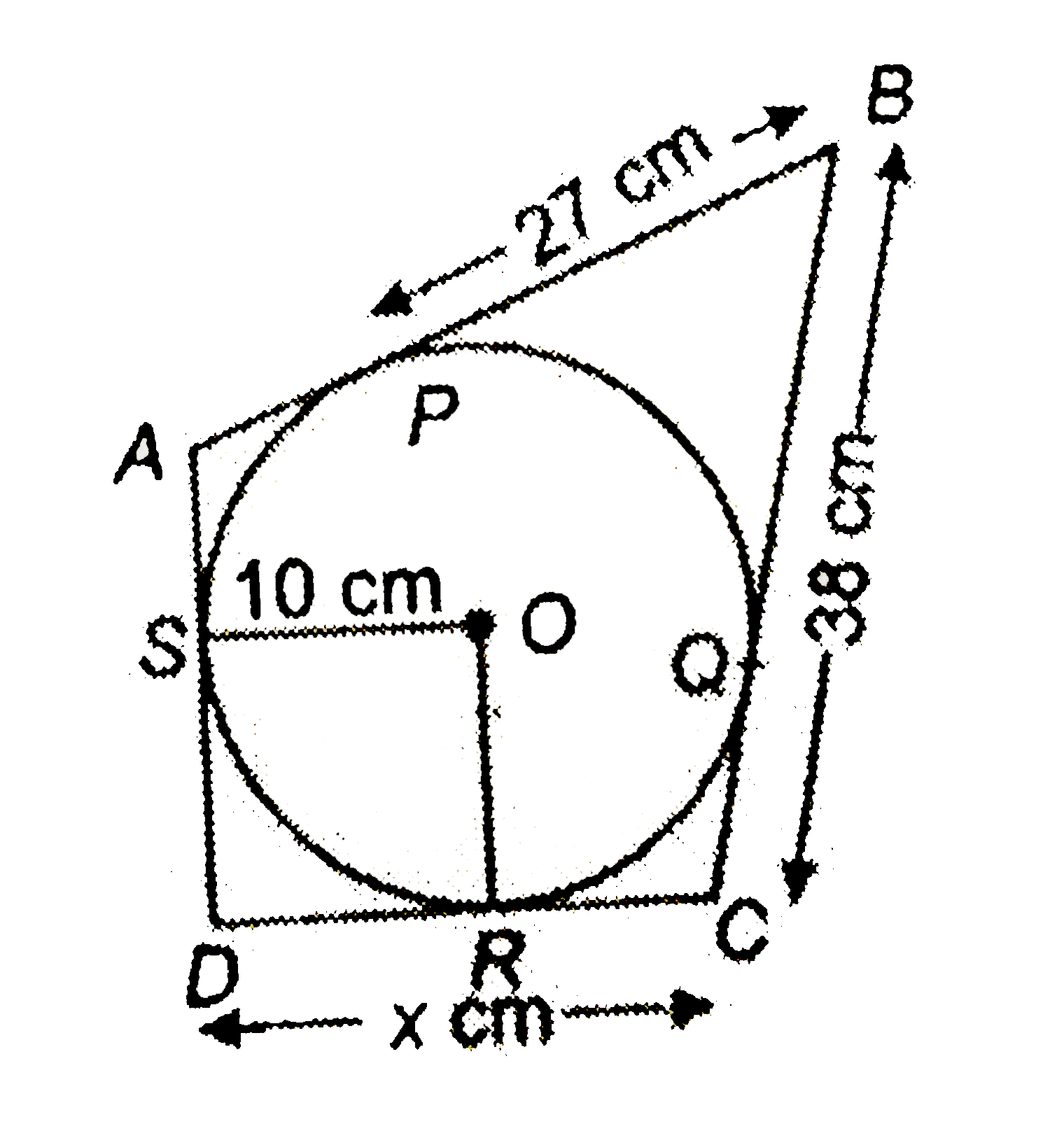
View Solution
