Question

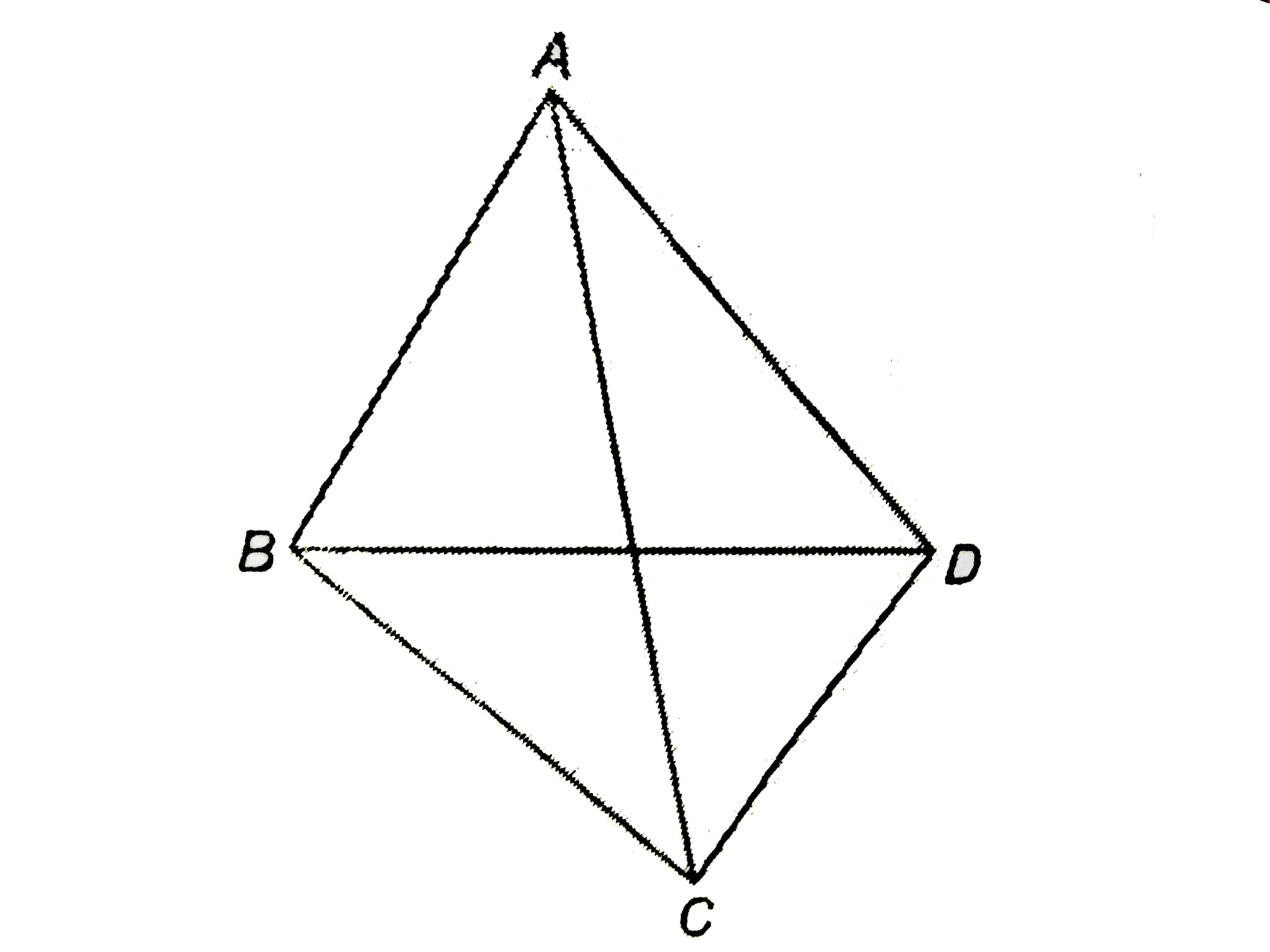
Show that in a quadrilateral ABCD ${\displaystyle AB+BC+CD+DA>AC+BD}$

Explanation

To prove that in a quadrilateral ABCD, the sum of the lengths of the sides is greater than the sum of the lengths of the diagonals, we need to show that:

$$AB+BC+CD+DA>AC+BD$$

1. Consider Triangle ABC:
- In triangle ABC, by the triangle inequality, we have:
\( AB + BC > AC \quad \text{(Equation 1)} \)

2. Consider Triangle BCD:
- In triangle BCD, again by the triangle inequality, we have:
\( BC + CD > BD \quad \text{(Equation 2)} \)

3. Consider Triangle ACD:
- In triangle ACD, applying the triangle inequality gives us:
\( AD + CD > AC \quad \text{(Equation 3)} \)

4. Consider Triangle ADB:
- In triangle ADB, we can state:
\( AD + AB > BD \quad \text{(Equation 4)} \)

5. Add All Inequalities:
- Now, we will add all four inequalities (Equation 1, 2, 3, and 4):
\( (AB + BC > AC) + (BC + CD > BD) + (AD + CD > AC) + (AD + AB > BD) \)

- This gives us:
\( (AB + BC) + (BC + CD) + (AD + CD) + (AD + AB) > AC + BD + AC + BD \)

6. Combine Like Terms:
- On the left-hand side, we can combine like terms:
\( 2AB + 2BC + 2CD + 2AD > 2AC + 2BD \)

7. Simplify:
- Dividing the entire inequality by 2:
\( AB + BC + CD + DA > AC + BD \)

8. Conclusion:
- We have shown that:
\( AB + BC + CD + DA > AC + BD \)
- Thus, we have proved that the sum of the lengths of the sides of quadrilateral ABCD is greater than the sum of the lengths of its diagonals.