The average gas mileage for n vehicles on one car lot is 21 miles per gallon. The average gas mileage for p vehicles on another car lot is 25 miles per gallon. When the vehicles on both lots are combined, the average gas mileage is 22.5 miles per gallon. What is the value of pn?
38
12
35
1
The correct Answer is:C
To solve the problem, we need to find the ratio pn where n is the number of vehicles on one car lot with an average gas mileage of 21 miles per gallon, and p is the number of vehicles on another car lot with an average gas mileage of 25 miles per gallon. The combined average gas mileage of both lots is 22.5 miles per gallon.
1. Define the Variables:
- Let n = number of vehicles on lot A (average mileage = 21 mpg)
- Let p = number of vehicles on lot B (average mileage = 25 mpg)
2. Write the Equations for the Sums:
- The total mileage for lot A: SumA=21n
- The total mileage for lot B: SumB=25p
3. Write the Equation for the Combined Average:
The combined average mileage for both lots can be expressed as:
SumA+SumBn+p=22.5
Substituting the sums:
21n+25pn+p=22.5
4. Cross-Multiply to Eliminate the Fraction:
Multiply both sides by n+p:
21n+25p=22.5(n+p)
5. Distribute on the Right Side:
21n+25p=22.5n+22.5p
6. Rearrange the Equation:
Move all terms involving n to one side and all terms involving p to the other side:
21n−22.5n=22.5p−25p
This simplifies to:
−1.5n=−2.5p
7. Divide Both Sides by -1:
1.5n=2.5p
8. Rearranging to Find the Ratio:
Divide both sides by n and p:
pn=1.52.5
9. Simplify the Fraction:
To simplify 1.52.5, multiply the numerator and denominator by 2 to eliminate the decimal:
1.5×22.5×2=35
10. Final Answer:
Thus, the value of pn is 35.
- Let n = number of vehicles on lot A (average mileage = 21 mpg)
- Let p = number of vehicles on lot B (average mileage = 25 mpg)
- The total mileage for lot A: SumA=21n
- The total mileage for lot B: SumB=25p
The combined average mileage for both lots can be expressed as:
SumA+SumBn+p=22.5
Substituting the sums:
21n+25pn+p=22.5
Multiply both sides by n+p:
21n+25p=22.5(n+p)
21n+25p=22.5n+22.5p
Move all terms involving n to one side and all terms involving p to the other side:
21n−22.5n=22.5p−25p
This simplifies to:
−1.5n=−2.5p
1.5n=2.5p
Divide both sides by n and p:
pn=1.52.5
To simplify 1.52.5, multiply the numerator and denominator by 2 to eliminate the decimal:
1.5×22.5×2=35
Thus, the value of pn is 35.
Topper's Solved these Questions
Similar Questions
A man motors from A to B. A large part of the distace is uphill and he gets a mileage of only 10 miles per gallon of gasoline. ON the return trip, he makes 15 miles per gallon, then the average of his mileage (assuming that the distance from A to B is 60 miles ) is
View Solution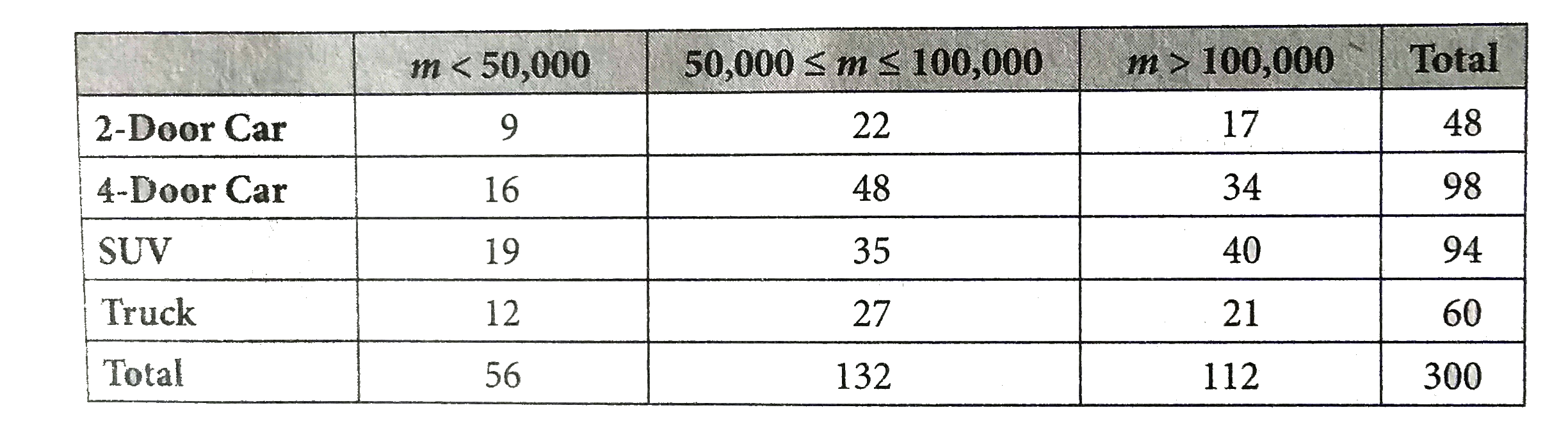
The table above shows the distribution of vehicles at a used car lot by type and by mileage. According to the data, which type of vehicles on the lot has the smallest percentage with less than 50, 000 miles?View Solution
Knowledge Check
- Question 1 - Select One
The gas mileage for Jan's car is 28 miles per gallon when the car travels at an average speed of 55 miles per hour. The car's gas tank has 20 gallons of gas at the beginning of a trip. If Jan drives at an average speed of 55 miles per hour, Which of the following functions f models the number of gallons of gas remaining in the tank t hours after the trip begins ?
Af(t)=20−2855tBf(t)=20−55t28Cf(t)=20−28t55Df(t)=20−55t28 - Question 2 - Select One
In the United States, the maintanance and contruction of airports, transit systems, and major roads are largely funded through a federal excise tax on gasoline. Based on the 2011 statistics given below, how much did the average house hold pay per year in federal gasoline taxes?
* The federal gasoline tax rate was 18.4 cents per gallon.
* The average motor vehicles was driven approximately 11,340 miles per year.
* The national average fuel economy for noncommercial vehicles was 21.4 miles per gallon.
* The average American household owned 1.75 vehicles.A55.73B68.91C97.52D170.63 - Question 3 - Select One
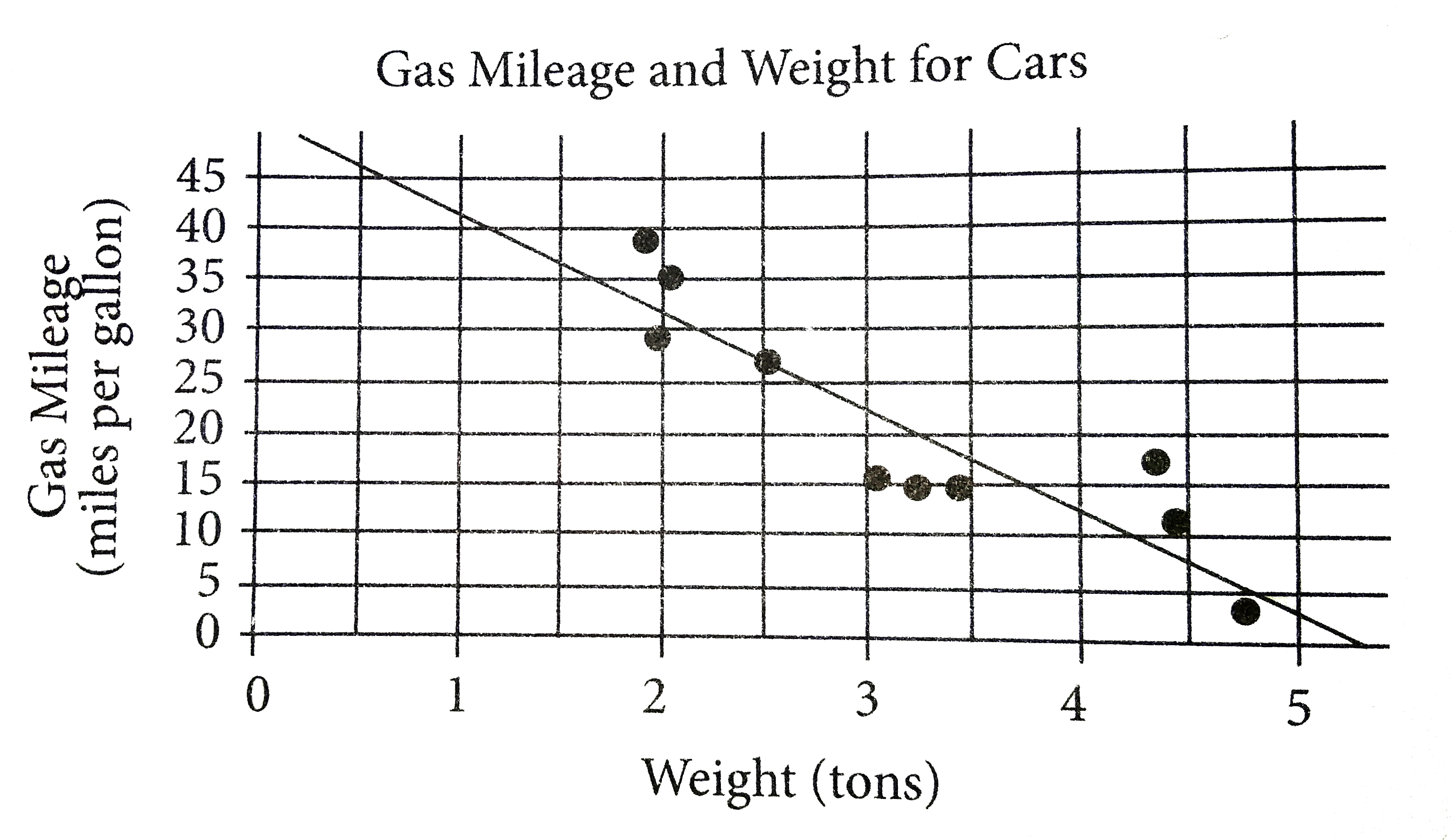
The scatterplot above shows the relationship between gas mileage, in miles per gallon, and weight , in tons, for 10 cars selected at random. The line of best fit models the gas mileage based on the weight of the car. What is the weight, in tons, of the car for which the actual gas mileage was closest to the predicted value?A2.5B4.5C15D27
A car travels from Twon A to Twon B at an average speed of 40 miles per hour, and returns immediately along the same route at an average speed of 50 miles per hour. What is the average speed in miles per hour for the round - trip?
View SolutionA bus diver drove at an average speed of 45 miles per hour for 3 hours while the bus consumed fuel at a rate of 15 miles per gallon. How many gallons of fuel did the bus use for the entire 3 - hour trip?
View SolutionA commercial airline has calculated that the approximately fuel mileage for its 600-passenger airplane is 0.2 miles per gallon when the plane travels at an average speed of 500 miles per hour. Flight 818's fuel tank has 42,000 gallons of fuel at the beginning of an international flight. If the plane travels at average speed of 500 miles per hour, which of the following functions f models the number of gallons of fuel remaining in the tank t hours after the flight begins?
View SolutionPaula jogged for a total of 30 minutes. Her average speed for the first 10 minutes was 5 miles per hour. During the remainder of her time, she jogged 2.5 miles. What was Paula's average for her entire jog ?
View SolutionA car starts a trip with 20 gallons of gas in its tank. The at traveled at an average speed of 65 miles per hour for 3 hours and consumed gas at a rate of 30 miles per gallon. What percent of the gas in the tank was used for the 3-hour trip?
View Solution

