The circumference of a circle circumscribing an equilateral triangle is 24πunits. Find the area of the circle inscribed in the equilateral triangle.
Text Solution
The correct Answer is:36π sq. unit
In the figure, circumcircle of equilateral triangle ABC has circumference 24π.
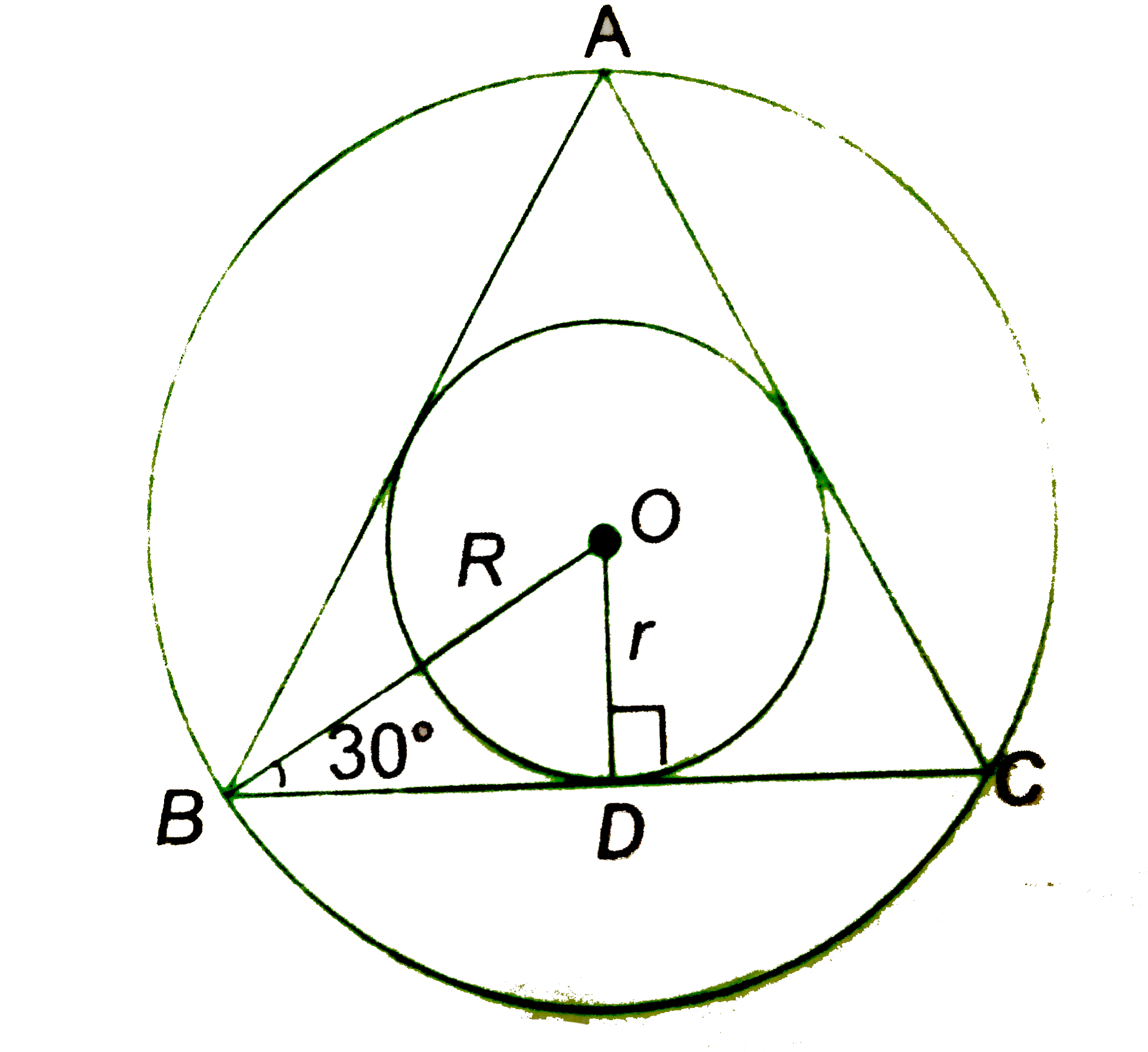
Let R be the radius of the circle and O be the centre.
∴2πR=24π
⇒R=12
Circle inscribed in triangle touches BC at D, which is midpoint of BC.
In triangle ODB, sin30∘=ODOB=rR (r is the radius of incircle)
∴r=122=6
∴ Area of inscrived circle =πr2=36π sq. unit
|
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.2
8 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.3
4 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE
38 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)
1 Videos
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGECHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type
1 Videos
Similar Questions
Knowledge Check
The area of circle inscribed in an equilateral triangle of side 12 cm is
A12cm2Bπcm2C12πcm2DNone of theseThe area of circle inscribed in an equilateral triangle of side 12 cm is
A12cm2Bπcm2C12πcm2DNone of theseIf the area of a circle inscribed in an equilateral triangle is 4π cm2 then what is the area of the triangle ?
A12√3 cm2B9√3 cm2C8√3 cm2D18 cm2
A circle is inscribed in an equilateral triangle of side a. Find the area of any square inscribed in this circle.
View SolutionA circle is cirumscribed in an equilateral triangle of side 'l'. The area of any square inscribed in the circle is :
View SolutionThe area of circle inscribed in an equilateral triangle of side 24 cms is -----
View SolutionA circle is inscribed in an equilateral triangle of side a. What is the area of any square inscribed in the circle ?
View SolutionA circle is cirumscribed in an equilateral triangle of side 'l'. The area of any square inscribed in the circle is :
View Solution
