The following table gives the distribution of total household expenditure (in rupees) of manual workers in a city. Expenditure: (in Rs.) 100-150 150-200 200-250 250-300 300-350 350-400 400-450 450-500 Frequency: 24 40 33 28 30 22 16 7 Find the average expenditure (in Rs.) per household.
To find the average expenditure per household from the given frequency distribution, we will follow these steps:
Step 1: Identify the midpoints (xi) of each class interval
The midpoint (xi) for each class interval can be calculated using the formula:
xi=Lower limit+Upper limit2
- For the class 100-150:
x1=100+1502=125
- For the class 150-200:
x2=150+2002=175
- For the class 200-250:
x3=200+2502=225
- For the class 250-300:
x4=250+3002=275
- For the class 300-350:
x5=300+3502=325
- For the class 350-400:
x6=350+4002=375
- For the class 400-450:
x7=400+4502=425
- For the class 450-500:
x8=450+5002=475
Step 2: Create a table for xi and fi
Now we can summarize the midpoints and the frequencies in a table:
| Expenditure (in Rs.) | Frequency (fi) | Midpoint (xi) |
|-----------------------|----------------|----------------|
| 100 - 150 | 24 | 125 |
| 150 - 200 | 40 | 175 |
| 200 - 250 | 33 | 225 |
| 250 - 300 | 28 | 275 |
| 300 - 350 | 30 | 325 |
| 350 - 400 | 22 | 375 |
| 400 - 450 | 16 | 425 |
| 450 - 500 | 7 | 475 |
Step 3: Calculate the product of xi and fi (xi * fi)
Now we will calculate the product of each midpoint (xi) and its corresponding frequency (fi):
- 24×125=3000
- 40×175=7000
- 33×225=7425
- 28×275=7700
- 30×325=9750
- 22×375=8250
- 16×425=6800
- 7×475=3325
Step 4: Summation of fi and xi * fi
Now we will find the summation of frequencies and the summation of the products (xi * fi):
- Σfi=24+40+33+28+30+22+16+7=200
- Σ(xi⋅fi)=3000+7000+7425+7700+9750+8250+6800+3325=53250
Step 5: Calculate the average expenditure (mean)
Using the formula for mean:
Mean=Σ(xi⋅fi)Σfi
Substituting the values:
Mean=53250200=266.25
Final Answer
The average expenditure per household is Rs. 266.25.
---
The midpoint (xi) for each class interval can be calculated using the formula:
xi=Lower limit+Upper limit2
x1=100+1502=125
- For the class 150-200:
x2=150+2002=175
- For the class 200-250:
x3=200+2502=225
- For the class 250-300:
x4=250+3002=275
- For the class 300-350:
x5=300+3502=325
- For the class 350-400:
x6=350+4002=375
- For the class 400-450:
x7=400+4502=425
- For the class 450-500:
x8=450+5002=475
Now we can summarize the midpoints and the frequencies in a table:
|-----------------------|----------------|----------------|
| 100 - 150 | 24 | 125 |
| 150 - 200 | 40 | 175 |
| 200 - 250 | 33 | 225 |
| 250 - 300 | 28 | 275 |
| 300 - 350 | 30 | 325 |
| 350 - 400 | 22 | 375 |
| 400 - 450 | 16 | 425 |
| 450 - 500 | 7 | 475 |
Now we will calculate the product of each midpoint (xi) and its corresponding frequency (fi):
- 40×175=7000
- 33×225=7425
- 28×275=7700
- 30×325=9750
- 22×375=8250
- 16×425=6800
- 7×475=3325
Now we will find the summation of frequencies and the summation of the products (xi * fi):
- Σ(xi⋅fi)=3000+7000+7425+7700+9750+8250+6800+3325=53250
Using the formula for mean:
Mean=Σ(xi⋅fi)Σfi
Substituting the values:
Mean=53250200=266.25
The average expenditure per household is Rs. 266.25.
Topper's Solved these Questions
Similar Questions
The following table gives the distribution of total household expenditure (in rupees) of manual workers in a city. Expenditure (in rupees) (xi) Frequency (fi) 100-150 24 150-200 40 200-250 33 250-300 28 300-350 30 350-450 22 400-450 16 450-500 7 Find the average expenditure (in rupees) per household.
View SolutionThe following data gives the distribution of total household expendilture ( in Rs.) of workers is a city :
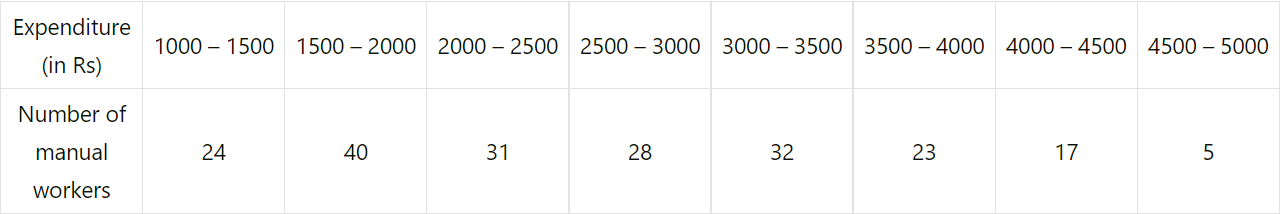
Find the average expenditure which is being done by maximum number of manual workers.View SolutionThe following data gives the distribution of total household expenditure (in rupees) of manual workers in a city: Expenditure (Rs.) Frequency Expenditure ( Rs.) Frequency 1000-1500 24 3000-3500 30 1500-2000 40 3500-4000 22 2000-2500 33 4000-4500 16 2500-3000 28 4500-5000 7 Find the average expenditure which is being done by the maximum number of manual workers.
View SolutionThe following data gives the distribution of total monthly household expenditure of 200 families of a village. Find the modal monthly expenditure of the families. Also, find the mean monthly expenditure: Expenditure (Rs.) Frequency Expenditure (Rs.) Frequency 1000-1500 24 3000-3500 30 1500-2000 40 3500-4000 22 2000-2500 33 4000-4500 16 2500-3000 28 4500-5000 7
View SolutionThe table below shows the daily expenditure on food of 25 households in a locality. daily expenditure(in rs) 100-150 150-200 200-250 250-300 300-350 no. of households 4 5 12 2 2 Find the mean daily expenditure on food by a suitable method.
View SolutionThe cost of 30 mangoes is less then cost of 40 mangoes by Rs 50. Find the cost of 50 mangoes in (Rs)
(a) 400
(b) 300
(c) 250
(d) 350View SolutionFind the median of the following data: Classes: 0-50 50-100 100-150 150-200 200-250 250-300 300-350 Frequency: 2 3 5 6 5 3 1
View SolutionThe following table shows the daily production of T.V. sets in an industry for 7 days of a week: Day, Mon, Tue, Wed, Thurs, Fri, Sat, Sun No. of T.V. Sets, 300, 400, 150, 250, 100, 350, 200 Represent the above information by a pictograph
View SolutionThe monthly profits (in Rs.) of 100 shops are distributed as follows: Profits per shop: 0-50 50-100 100-150 150-200 200-250 250-300 No. of shops: 12 18 27 20 17 6 Draw the frequency polygon for it.
View SolutionThe following table gives the weights (in grams) of 30 boxes of fruits :
Weight (in grams)350425500375475400450Number of boxes5364237
Find the mean and the median.
Using empirical formula, calculate its mode.View Solution

