
The function f, shown in the graph above, is defined for −7≤x≤7. For which of the following values of x does f(x)=4 ?
I. −4
II. −3
III. 5
A
III only
B
I and II only
C
II and III only
D
I, II, and III
Text Solution
The correct Answer is:C
The term f (x) is equivalent to the y-value of the function at x. So this question is aksing. "which vlaues of x produce a y-value of 4?" Draw a horizontal line at the point y=4 and identify where that line intercepts the function. The two points on intersection are -3 and 5. (C ) is correct.
|
Topper's Solved these Questions
- FUNCTIONS BOOK - KAPLANCHAPTER - FUNCTIONS EXERCISE - FUNCTION NOTATION
1 Videos
- FUNCTIONS BOOK - KAPLANCHAPTER - FUNCTIONS EXERCISE - GRAPHS OF FUNCTIONS
1 Videos
- FUNCTIONS BOOK - KAPLANCHAPTER - FUNCTIONS EXERCISE - DESCRIBING REAL-LIFE SITUATIONS WITH FUNCTIONS
1 Videos
- EXPONENTS, RADICALS, POLYNOMIALS, AND RATIONAL EXPRESSIONSBOOK - KAPLANCHAPTER - EXPONENTS, RADICALS, POLYNOMIALS, AND RATIONAL EXPRESSIONSEXERCISE - RATIONAL EXPRESSIONS AND EQUATIONS
1 Videos
- GEOMETRY BOOK - KAPLANCHAPTER - GEOMETRY EXERCISE - THREE-DIMENSIONAL FIGURES
1 Videos
Similar Questions
Knowledge Check
The function f (x) is defined as under :
f(x)={3x−1≤x≤14−x1<x<4
The above function is:Acontinuous at x = 1Bdifferentiable at x = 1Ccontinuous but not differentiable at x = 1Dnone of theseFor the function f(x)=x2−6x+8,2≤x≤4, the value of x for which f'(x) vanishes is
A3B52C94D72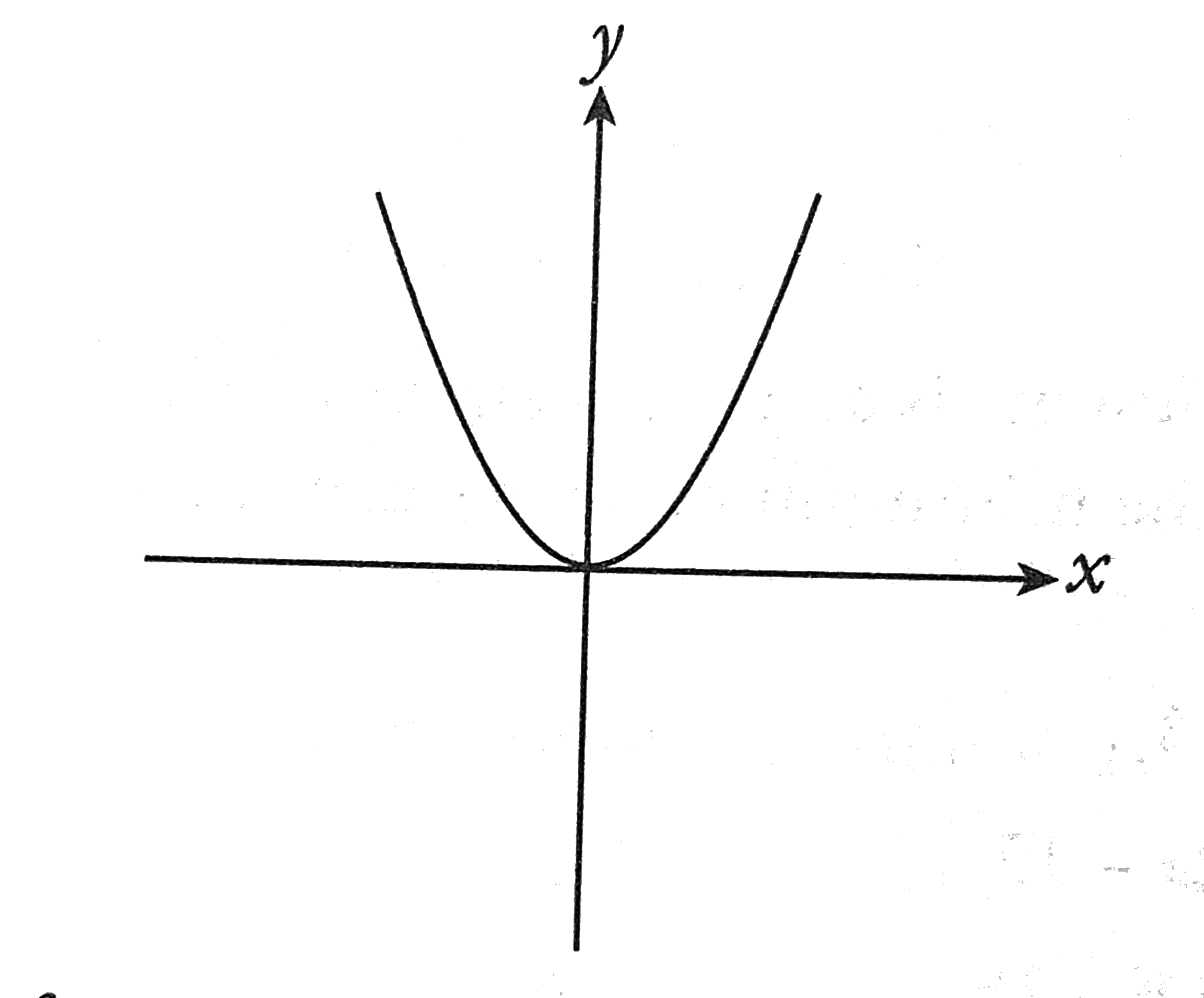
The quadratic y=f(x) is shown above. Which of the following graphs represents the function y=f(x+3)−4?A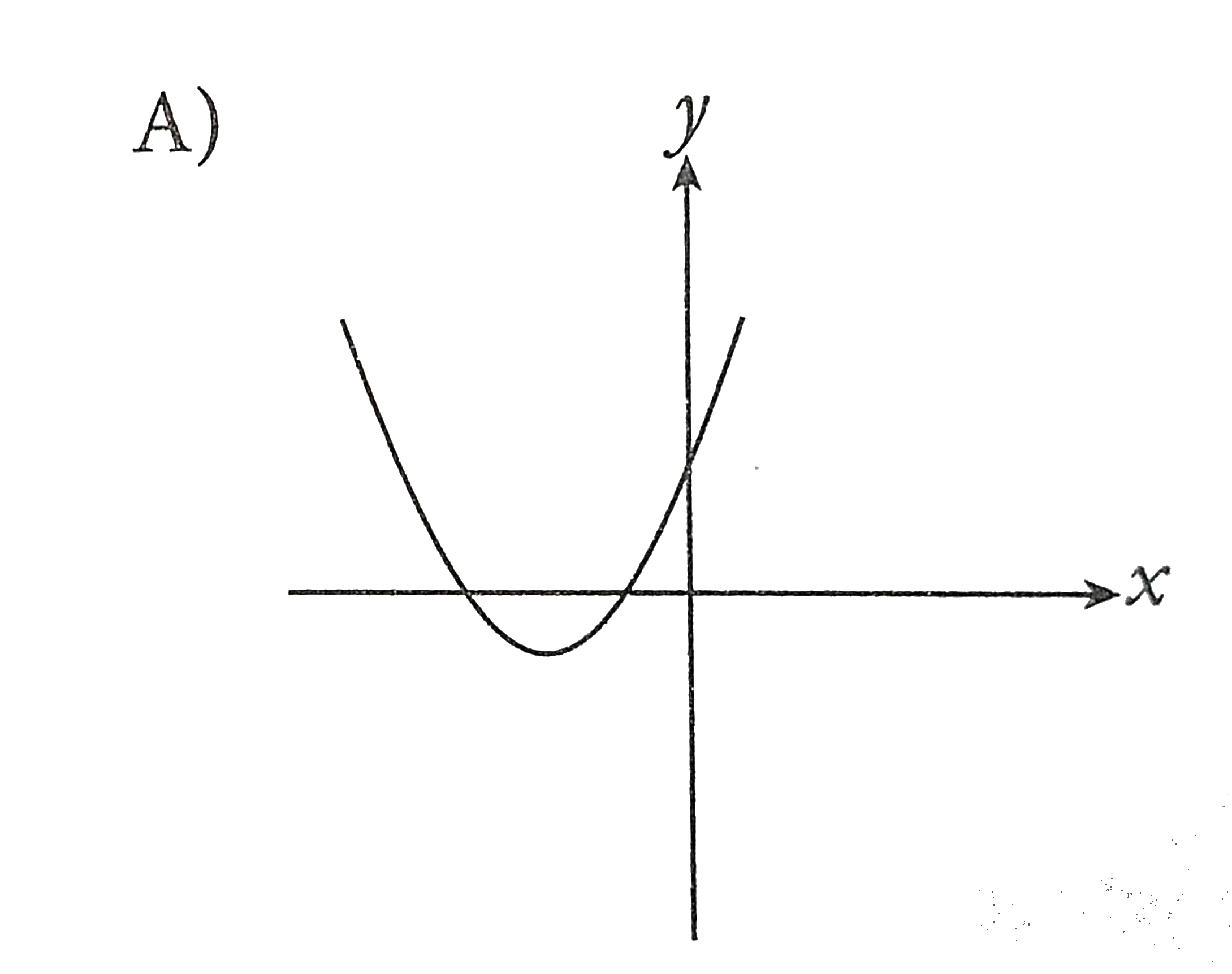 B
B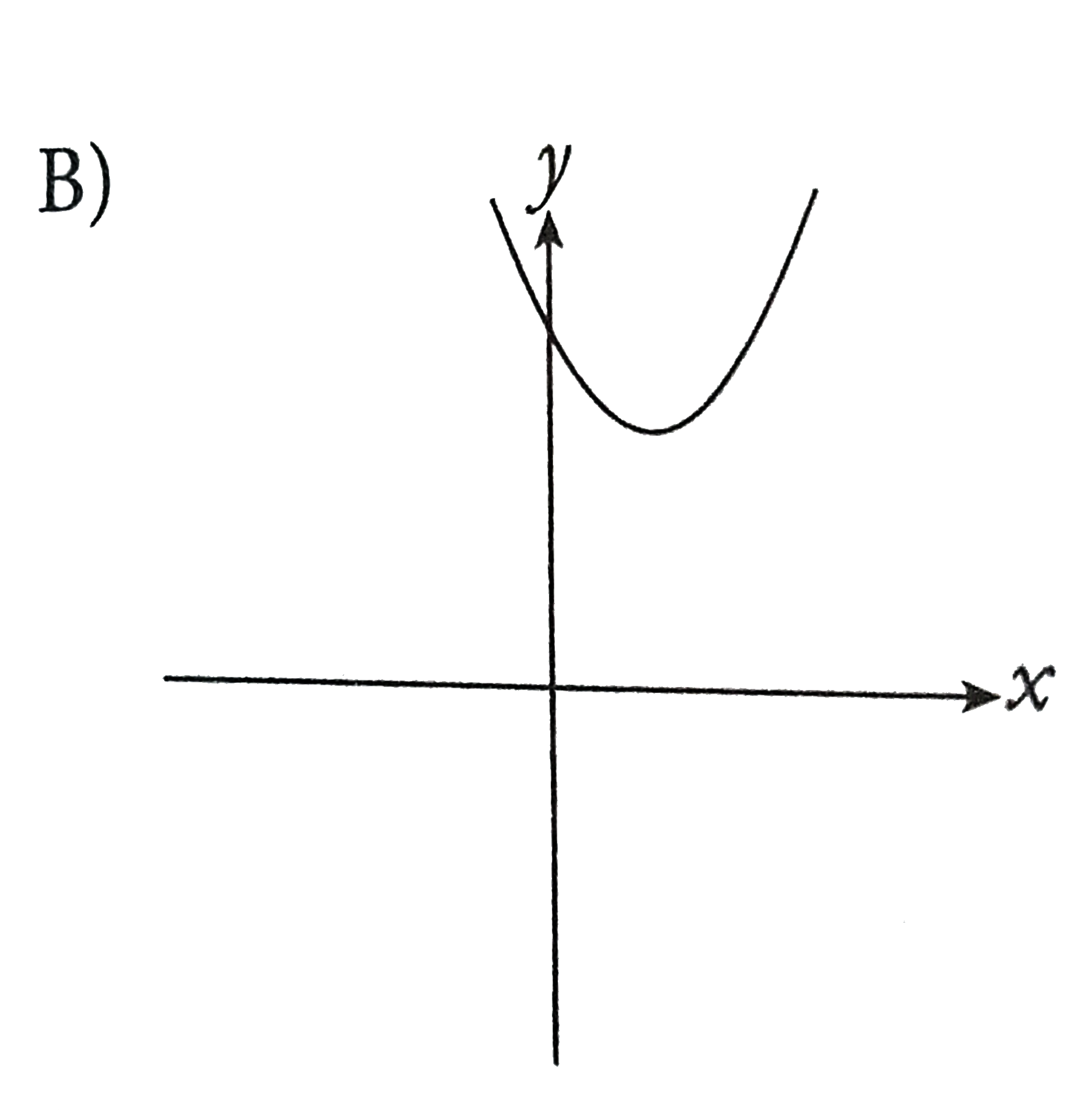 C
C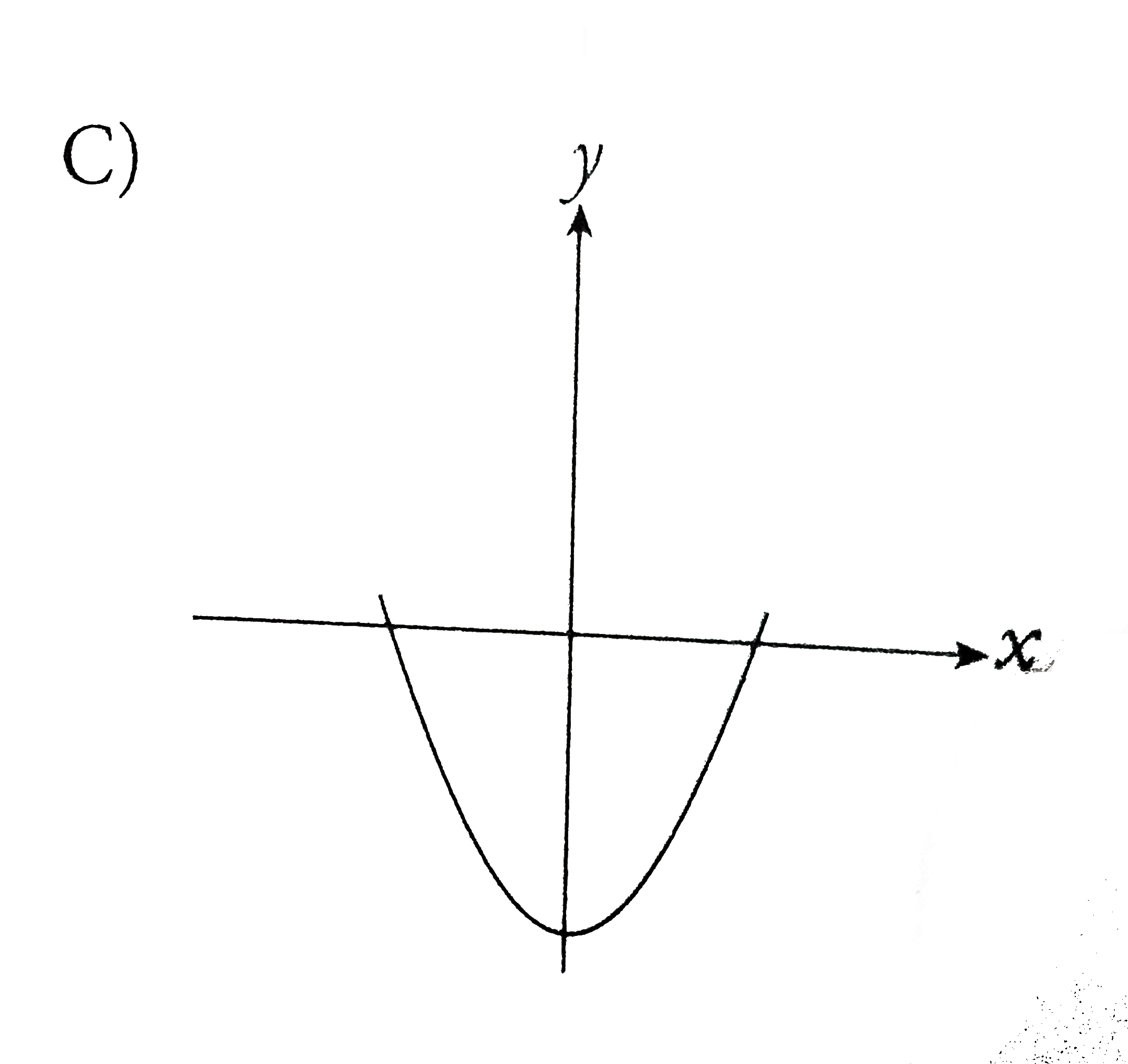 D
D
For the function f(x)=x2−6x+8,2≤x≤4, the value of x for which f'(x) vanishes is
View SolutionLet A={x:−1≤x≤1}=B be a function f:A→B. Then find the nature of each of the following functions.
(i) f(x)=|x| (ii) f(x)=x|x|
(iii) f(x)=x3 (iv) f(x)=sinπx2
View SolutionLet A={x:−1≤x≤1}=B be a function f:A→B. Then find the nature of each of the following functions.
(i) f(x)=|x| (ii) f(x)=x|x|
(iii) f(x)=x3 (iv) f(x)=sinπx2
View SolutionDiscuss the continuity of the function f, where f is defined by f(x)⎧⎪⎨⎪⎩3,if0≤x≤14,if1<x<35,if3≤x≤10
View SolutionIf f(x)=4x−3, where −3≤x≤1 then the range of the function f is given by
View Solution
